Conservation of Mass - Clarkson University

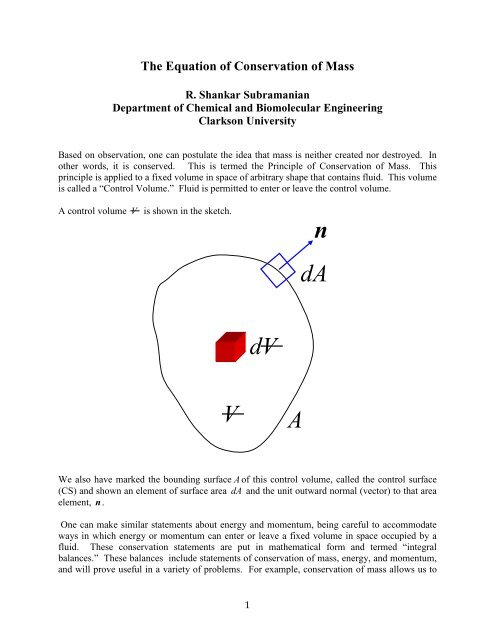
estimate the rate of change of the level of liquid in a process vessel or the rate at which theamount of gas left in a tank decreases due to leakage. Conservation of mass and energy allow usto size pumps and turbines, and help in the evaluation of flow rates using flow measurementdevices. Conservation of momentum is used in calculating the forces on the supports used forpipe bends and the forces flange bolts need to withstand. Also, by using these balances together,one can calculate the losses in a sudden expansion, design a jet ejector, and make calculationsinvolving pipe manifolds.Now, we shall proceed to express the idea that the total mass in the control volume that is shownon page 1 is conserved.Rate of increase of mass of material within the control volume = Net rate at which materialenters the control volume.Let us write a mathematical representation of the above statement. If we designate the total massof material in the control volume as M and the net rate of entry of mass into the control volumeas m , conservation of mass can be written asdMdt= m (1)Now, we need to work out suitable results for the left and right sides of the above equation. Ifwe consider a differential volume dV , the mass of fluid in that volume is obtained bymultiplying the volume by the local density at that point ρ . By adding up all the differentialvolumes within the total volume V , we can obtain the total mass of fluid M . Therefore, wecan writeM= ∫ ρ dV(2)VThe time rate of change of this mass is then dM dt. Therefore,dMdtd= ρ dVdt∫ (3)VNow, we need to develop a result for the net rate of entry of fluid into the control volumethrough the control surface. For this, we consider the differential area element dA . If thevelocity vector is V , the component of this velocity that is directed into the control volume isgiven by − V • n , because the unit normal vector n points outward from the control volume.This result, multiplied by the area of the element dA , gives the volumetric rate at which fluidenters the control volume through this area element, labeled dQ .2

Here is an example of how we may use this statement of “Conservation of Mass” at steady state.A fluid is in steady flow through a pipe of changing cross-section as shown in the sketch., V,Aρ , V , Aρ1 1 12 2 2n 1n 2Location 1 is at the inlet and location 2 is at the outlet. Assume the velocity profiles are flat.The control volume is indicated by the dashed boundary. It is shown as being slightly separatedfrom the physical boundary only for clarity. In reality, its surface coincides with the physicalsurface of the pipe. Note that there is no flow through most of the control surface, that is,V • n =0 . There is flow only at the inlet (1) and exit (2). At the inlet surface the velocity pointsin a direction opposite to that of the normal vector. Therefore, V • n becomes − V1. In a likemanner, at the exit surface, the velocity points in the same direction as the normal vector whichleads to V • n becoming V2. Because V1and V2are assumed to be constant across thesurfaces involved, the integrals are easy to evaluate and we get− ρVA+ ρ VA = (9)1 1 1 2 2 20orρVA= ρ VA(10)1 1 1 2 2 2The product of the uniform velocity and the area is the volumetric flow rate Q . Therefore, wecan rewrite this asρQ= ρ Q(11)1 1 2 24

The mass flow rate m = ρ Q so that m = m1 = m 2is constant. If the density is constant, the aboverelationship reduces to Q1 = Q2. This assumption of constant density is known as theassumption of “incompressible flow.” Even though the concept of incompressibility refers tochanges in density associated with pressure changes, the term is used loosely to signify “constantdensity.”In isothermal single-component liquids, the assumption of constant density is nearly always anexcellent one to make. In the case of gases flowing at velocities that are small compared withthe speed of sound in the gas, it continues to be a good assumption. When density changes in afluid caused by pressure, temperature, or composition variations are significant when comparedwith the average density, a precise calculation must accommodate such density variations.Accommodating Velocity Variation Across the Cross-SectionIn the simplification of the principle of conservation of mass, we assumed the velocity profile tobe flat at the inlet and the exit. Realistically, the velocity of a fluid satisfies the no-slip conditionat a solid boundary, and varies across the cross-section. This variation of velocity can be easilyaccommodated by using an average velocity Vavin Equations (9) and (10). The averagevelocity across the cross-section is defined as follows.Vav∫V • ndAQA1= = = • dAAA∫V n (12)AdA∫AIn Equation (12), the cross-sectional area A is oriented normal to the direction of flow, and n isa unit normal to the area in the direction of flow. The symbol dA stands for a differential areaV relement. As an example, for a circular tube of radius R , in which the velocity distribution ( )is symmetric about the tube axis, the cross-section and differential area dA = 2πrdr areillustrated in the sketch below.RrdrRing of area dA = 2πrdr5

Applying the definition given in Equation (12) yieldsRQ 1 2V = av2πrV ( r) dr rV ( r)drπR = πR = R2 2 20 0R∫ ∫ (13)Two example problems are considered next. The first example involves using the steady versionof the equation of conservation of mass to make calculations involving a natural gas pipeline(source: Fluid Mechanics for Chemical Engineers by Noel de Nevers). The second exampleshows how to use the unsteady version of the equation of conservation of mass to calculate therate of change of the height of liquid in a tank.Example 1 -- Natural Gas PipelineD1 = 2.00 ftD2 = 2.50V1 = 33.7 ft / sV2= ?ft1 2p1 = 820 psiap2 = 450 psiaT1 = 70 FT2 = 58 FGas Constant R = 10.73g( f/ )2 3lb in • ftMolecular Weight = 16• R( lb mole)lblb moleFind m, V2 2This is a long pipeline, which is shown schematically. The natural gas flows through a section ofcircular pipe of diameter 2.00 feet for some distance, and then through a larger section of circularpipe of diameter 2.50 feet. The pressure and temperature at locations 1 and 2 are specified. Weneed to find the velocity at location 2, and the mass flow rate at location 2.6

π πA ( ) 21= D1 = × 2.00 ft = 3.14 ft4 42 2A π π( ) 22= D2 = × 2.50 ft = 4.91 ft4 42 2Let us find the mass flow rate.⎛ lb ⎞ ⎛ ft ⎞2 lbm2 = m = m1= ρ1V 1A1 = 2.31⎜ 33.7 3.143 ⎟× ⎜ ⎟× ( ft ) = 244⎝ ft ⎠ ⎝ s ⎠sNow, we can calculate the velocity V2fromV( lb s)m244 /= = =22ρ2A21.30 /34.912( lb ft ) × ( ft )38.2ftsExample 2 – Unsteady Mass BalanceDia D=2 m1V1 = 1.5 m/sD = cm15Free surfacehV2 = 1.0 m/sD = cm282Is the liquid level in the above tank rising or falling? How fast?To solve this problem, we must use the unsteady version of the conservation of mass equationapplied to a control volume that is shown in the sketch using a dashed line. The control volumeoccupies the entire interior of the tank, including the inlet and exit pipes. There is an entrance tothe control volume at location 1 and an exit at location 2. Liquid flows into the tank at location 1and flows out at location 2, as shown in the sketch.8

We begin withdMdtCS( )= − ∫ ρ V • nwhere M ( t ) is the mass of the liquid in the control volume, which depends on time t . Thedensity of the liquid ρ can be assumed to be constant.We can write the mass content in the control volume as the sum of the mass of the liquid in the2tank, given by ( π /4) ρ Dht ( ) , and the constant mass in the inlet and outlet pipe sections thatare contained the control volume, termed C . The integral on the right side of the unsteady massconservation equation works out to ρ ( Q1 − Q2). Using this information, the unsteady massconservation equation becomesdAd ⎛π⎞ π dh⎜ ρ ⎟ ρ ρdt ⎝ 4 ⎠ 4 dt( )2 2Dh+ C = D = Q1 −Q2ordhdt=( − )4 Q Q1 22π DTherefore, we must calculate the numerical value of the right side in the above equation. Theareas of the inlet and outlet can be calculated as follows.π π( ) 2−A1 = D1 = × 0.05 m = 1.96 × 104 4mπ π( ) 2−A2 = D2 = × 0.08 m = 5.03 × 104 4m2 3 22 3 2Therefore, the volumetric flow rates are⎛m⎞−Q1 = VA1 1= 1.5 ⎜ ⎟× 1.96 × 10 ( m ) = 2.94 × 10⎝ s ⎠3 2 −3⎛m⎞Q2 = VA2 2= 1.0 ⎜ ⎟× 5.03× 10 ( m)= 5.03×10⎝ s ⎠−3 2 −3Substitute the above information in the differential equation for the height of the liquid in thetank.−−( Q − Q ) ( × − × )3 3 3dh 44 2.94 10 5.03 10 m / s1 2 −4m= = = − 6.66 × 1022dt π D πs( 2.0 m)ms3ms39

Because the rate of change of the height of liquid in the tank is negative, the liquid level isfalling.10