Exam 1 Solutions – 100 points

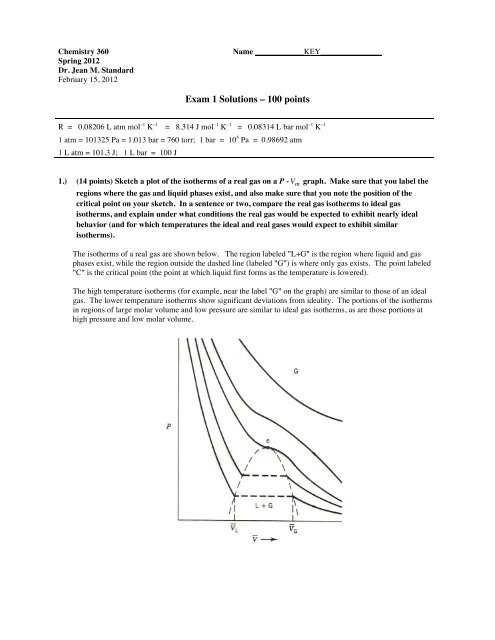
Chemistry 360Spring 2012Dr. Jean M. StandardFebruary 15, 2012Name ____________KEY_______________Exam 1 Solutions – 100 pointsR = 0.08206 L atm mol –1 K –1 = 8.314 J mol –1 K –1 = 0.08314 L bar mol –1 K –11 atm = 101325 Pa = 1.013 bar = 760 torr; 1 bar = 10 5 Pa = 0.98692 atm1 L atm = 101.3 J; 1 L bar = 100 J1.) (14 points) Sketch a plot of the isotherms of a real gas on a P - V m graph. Make sure that you label theregions where the gas and liquid phases exist, and also make sure that you note the position of thecritical point on your sketch. In a sentence or two, compare the real gas isotherms to ideal gasisotherms, and explain under what conditions the real € gas would be expected to exhibit nearly idealbehavior (and for which temperatures the ideal and real gases would expect to exhibit similarisotherms).The isotherms of a real gas are shown below. The region labeled "L+G" is the region where liquid and gasphases exist, while the region outside the dashed line (labeled "G") is where only gas exists. The point labeled"C" is the critical point (the point at which liquid first forms as the temperature is lowered).The high temperature isotherms (for example, near the label "G" on the graph) are similar to those of an idealgas. The lower temperature isotherms show significant deviations from ideality. The portions of the isothermsin regions of large molar volume and low pressure are similar to ideal gas isotherms, as are those portions athigh pressure and low molar volume.

2 a.) continued3To determine the internal energy change, we can start with the exact differential for an ideal gas andintegrate,dU = C v dTU 2T∫ dU = ∫2C v dTT1U 1ΔU = C vNote that here we have used that the heat capacity of an ideal gas is constant. Finally, we can substitute theinitial and final temperatures, and use the information that the constant volume heat capacity of an ideal€monatomic gas equals 3/2nR.ΔU = C v ΔT= 3 2 nRΔT= 3 2 1 molΔU = 1870 J∫ T1T 2ΔU = C v ΔT .The change in enthalpy may be calculated in a similar way, this time using the information that the constantpressure heat capacity€of an ideal monatomic gas equals 5/2nR.ΔH = C p ΔTdT( )( 8.314 J/molK) ( 250 −100 K )= 5 2 nRΔT= 5 2 1 molΔH = 3120 J( )( 8.314 J/molK) ( 250 −100 K )Finally, the heat may be calculated using the First Law, or by noting that at constant pressure the heatabsorbed or lost is equal to the enthalpy change,€q p = ΔH = 3120 J.€

4.) (15 points) True/false, short answer, multiple choice.7a.) True or False : The compression factor Z of a real gas approaches 1 at very high pressures.b.) True or False : A gas with a negative Joule-Thomson coefficientµ JT may be used as a refrigerant.€c.) Short answerThe _____heat capacity_____ is a quantity that provides a measure of the ability of a substance to storeenergy.d.) Short answerAn _____intensive______ variable is independent of the size of the system.e.) Multiple Choice. The Equipartition Theorem predicts that the molar internal energy of an ideal monatomicgas has the following form.1)U m = 5 2 RT .€2) U m = 3 2 RT .€€3) U m = 5 2 R.4) U m = 3 2 R.€

5.) (14 points) The temperature dependence of the molar constant pressure heat capacity of CO 2 gas may berepresented by the function8C p,m = α + β T + γ T 2 ,€€where α , β , and γ are constants. For CO 2 , these constants have the values α = 18.86 J K −1 mol −1 ,β = −0.01452 J K −2 mol −1 , and € γ = 3.142 ×10 −5 J K −3 mol −1 .In € this case, € 2.5 mol of CO 2 gas is heated reversibly from 300 to 1200 € K at a constant pressure of 1.0 bar.Calculate ΔH for the € process, assuming ideal gas behavior.In this problem, we know that the heat at constant pressure is the enthalpy,differential € of enthalpy isdq p = dH . For an ideal gas, thedH = C p dT .€On a molar basis, this equation becomes€dH = nC p,m dT .Integrating,€H 2T∫ dH = ∫2nC p,m dTT1H 1Substituting the values of the parameters, we have€TΔH = n ∫2( α + β T + γ T 2) dTT1ΔH = nα ( T 2 −T 1 ) + nβ 2 T 2 2 2( −T 1 ) + nγ 3 T 2 3 3( −T 1 ).ΔH = nα ( T 2 −T 1 ) + nβ 2 T 2 2 2( −T 1 ) + nγ 3 T 2 3 3( −T 1 )ΔH = ( 2.5 mol) ( 18.86 J mol −1 K −1)( 1200 K − 300 K)( 2.5 mol) ( −0.01452 J mol −1 K −2) 1200 K+ 1 ( ) 2 − ( 300 K) 22( )( )( 3.142 ×10 −5 J mol −1 K −3)(( 1200 K) 3 − ( 300 K) 3)( )( 16974 J/mol − 9801 J/mol + 17815J/mol)+ 1 2.5 mol3= 2.5 molΔH = 62470 J .Thus, at constant pressure,€q p = ΔH = 62470 J .€

6.) (14 points) Consider an equation of state for a gas given by9"$P +#CV m2%' V m = RT ,&where C is a constant. Starting from the definition of work, w = − P ext dV , derive an expression forthe work done in a reversible € isothermal expansion from V 1 to V 2 at temperature T.∫Starting from the definition of work, we have€w = −∫ P ext dV .Since the process is reversible,P ext = P , and so we have€w = − ∫ P dV .€We can obtain an expression for P from the equation of state given above. Solving the equation for P yields€"$P +#P +CV m2CV m2P = RTV m%' V m = RT&= RTV mC−2V m.Writing this in terms of V rather thanV m gives the relation€€P = nRTV− n2 CV 2 .Substituting this relation into the equation for work gives€w = −w = −∫ V1V 2#%%$nRTV− n2 CV 2V nRT∫2VdV +V 1&(( dV'V n 2 C∫2V 2 dV .V 1Since the process is isothermal, T can be pulled out of the integral along with the other constants to give€Integrating leads to the resultw = − nRTV 1∫2V dV + n 2 V 1CV 1∫2V 2 dV .V 1€#w = − nRT ln V &2%$ V 1 '( + n 2 C #− 1 V + 1 &%$ 2 V (.1 '€