Exercise 12: Drag on Circular Cylinder and Wall ... - KTH Mechanics

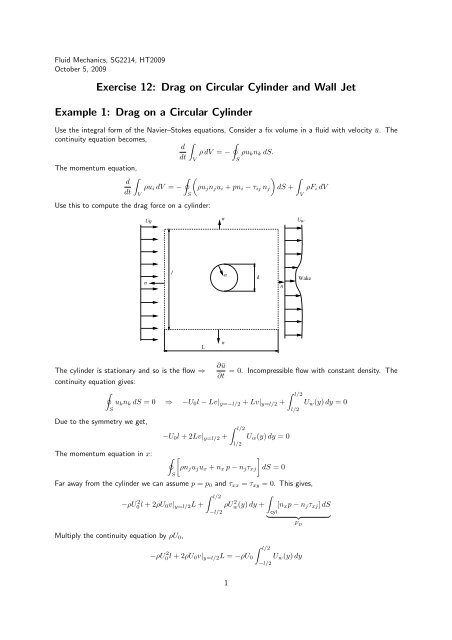
Fluid Mechanics, SG2214, HT2009October 5, 2009<strong>Exercise</strong> <strong>12</strong>: <strong>Drag</strong> on Circular Cylinder and Wall JetExample 1: <strong>Drag</strong> on a Circular CylinderUse the integral form of the Navier–Stokes equations, Consider a fix volume in a fluid with velocity ū. Thecontinuity equation becomes, ∫ ∮dρdV = − ρu k n k dS.dt VSThe momentum equation,∫ ∮) ∫dρu i dV = −(ρu j n j u i + pn i − τ ij n j dS + ρF i dVdt VSVUse this to compute the drag force on a cylinder:U0nUwnlndnWakeLnThe cylinder is stationary and so is the flow ⇒∂ū = 0. Incompressible flow with constant density. The∂tcontinuity equation gives:∮∫ l/2u k n k dS = 0 ⇒ −U 0 l − Lv| y=−l/2 + Lv| y=l/2 + U w (y)dy = 0Due to the symmetry we get,The momentum equation in x:S−U 0 l + 2Lv| y=l/2 +∮S∫ l/2l/2U w (y)dy = 0[ρn j u j u x + n x p − n j τ xj]dS = 0Far away from the cylinder we can assume p = p 0 and τ xx = τ xy = 0. This gives,∫ l/2 ∫−ρU0l 2 + 2ρU 0 v| y=l/2 L + ρUw(y)dy 2 + [n x p − n j τ xj ]dSMultiply the continuity equation by ρU 0 ,−l/2cyll/2} {{ }F D∫ l/2−ρU0l 2 + 2ρU 0 v| y=l/2 L = −ρU 0 U w (y)dy1−l/2

Insert into momentum equation:F D = ρ∫ l/2−l/2−ρU 0∫ l/2−l/2Make the variable substitution r = y/d,Now if d

Insert into equation (1):U m f ′ (U ′ mf ′ − U mg ′U m U mf ′ ′2 − Um2 g ′g ηf ′ f ′′ + Um2( Uf ′′′ ′+ m g 2∂ 2 u∂y 2 = ∂ (U m f ′′ 1 )= U m f ′′′ 1 ∂y g g 2g ηf ′′ ) + (U m g ′ ηf ′ − U ′ mgf − U m g ′ f)U m1g f ′′ = νU m1g 2 f ′′′g ′g ηf ′ f ′′ − U m U mff ′ ′′ − Um2+ U mg ′ )gff ′′ − U mg ′ 2f ′2 = 0ν} {{ν}β}ν{{ }αf ′′′ + αff ′′ + βf ′2 = 0Boundary conditions: ⎧ ⎧⎪⎨ u(0) = 0 ⎪⎨ f ′ (0) = 0v(0) = 0 ⇒ f(0) = 0⎪ ⎩ ⎪⎩u(∞) = 0 f ′ (∞) = 1Similarity requires that α and β are constant. Assume the x-dependence:U m = ax m , g = bx nThen we getβ = − 1 ν amxm−1 b 2 x 2n = − ab2 m, 2n + m = 1νg ′g ff′′ = νU m1g 2 f ′′′α = 1 ν amxm−1 b 2 x 2n + 1 ν axm bnx n−1 bx n = ab2 (m + n)νWe can choose the length scale g such that α = 1:So we have:ab 2ν = 1m + n⇒ β = − mm + nf ′′′ + ff ′′ + βf ′2 = 0 (3)For what β is the boundary conditions fulfilled? Work on (3):Integrate:Multiply with f ′ :Integrate:f ′′′ + ff ′′ + βf ′2 = 0⇒f ′′′ + (ff ′′ + f ′2 ) + (β − 1)f ′2 = 0f ′′ + ff ′ + (β − 1)g = 0, g(η) =f ′′ f ′ + ff ′2 + (β − 1)gf ′ = 0∫ ∞η⇒f ′2 dη > 0⇒f ′′ f ′ + (ff ′2 + gf ′ ) + (β − 2)gf ′ = 0<strong>12</strong> f ′2 + fg + (β − 2)Put in η = 0 and use the boundary conditions:Solve for m and n:(β − 2)∫ ∞0∫ ∞ηgf ′ dη = 0gf ′ dη = 0 ⇒ β = 2 ⇒β = −m = 2 ⇒ 3m + 2n = 0m + n⇒⇒m = − 1 2 , n = 3 43