Exercise 10: Potential Flow Example 1: Half body ... - KTH Mechanics

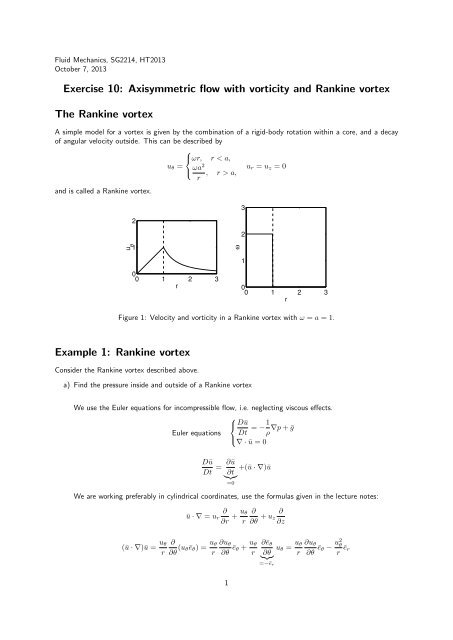
Fluid Mechanics, SG2214, HT2013October 7, 2013Exercise 10: Axisymmetric flow with vorticity and Rankine vortexThe Rankine vortexA simple model for a vortex is given by the combination of a rigid-body rotation within a core, and a decayof angular velocity outside. This can be described by⎧⎨ωr, r < a,u θ =⎩ωa 2r , r > a, u r = u z = 0and is called a Rankine vortex.232u θ1ω100 1 2 3r00 1 2 3rFigure 1: Velocity and vorticity in a Rankine vortex with ω = a = 1.Example 1: Rankine vortexConsider the Rankine vortex described above.a) Find the pressure inside and outside of a Rankine vortexWe use the Euler equations for incompressible flow, i.e. neglecting viscous effects.⎧⎨DūEuler equations Dt = − 1 ρ ∇p + ḡ⎩∇ · ū = 0DūDt = ∂ū +(ū · ∇)ū}{{}∂t=0We are working preferably in cylindrical coordinates, use the formulas given in the lecture notes:(ū · ∇)ū = u θr∂ū · ∇ = u r∂r + u θr∂∂θ (u θē θ ) = u θ ∂u θr ∂θ ēθ + u θr1∂∂θ + u ∂z∂z∂ē θu θ = u θ}{{}∂θ r=−ē r∂u θ∂θ ēθ − u2 θr ēr

c) Calculate the shape of a free surface at atmospheric pressure p 0 .Find the difference in z between r = 0 and r → ∞{r = 0 : p 0 = −ρgz 0 + C 1⇒ z ∞ − z 0 = C 2 − C 1= ω2 a 2r → ∞ : p 0 = −ρgz ∞ + C 2 ρg gDetermine the shape of the free surface:{p0 = ρω2 r 22− ρgz + C 1 r < a z ∼ r 2 ⇒ z = ω2 r 22g+ C1−p0ρgp 0 = − ρω2 a 42r− ρgz + C 2 2 r > a z ∼ 1r⇒ z = − ω2 a 42 2gr 2Set z = 0 at r = 0. Then C 1 = p 0 and we further get+ C2−p0ρgz = ω2 r 22gr < aandz = − ω2 a 42gr 2 + C 2 − C 1= − ω2 a 4ρg 2gr 2 + ω2 a 2= ω2 a 2 ) (1 − a2g g 2r 2r > aSo we have⎧⎨z(r) =⎩ω 2 r 22gω 2 a 2gr < a( )1 − a22r 2r > a .z0.10.090.080.070.060.050.040.030.020.0100 0.5 1 1.5 2 2.5 3rFigure 2: The free surface of a Rankine vortex with ω = a = 1 and g = 9.82.Example 2Show that the inviscid vorticity equationreduces to the equationin the case of axisymmetric flowD¯ωDt= (¯ω · ∇)ū)D ω= 0Dt(rū = u r (r, z, t)ē r + u z (r, z, t)ē z .3

The vorticity in an axisymmetric flow( ∂ur¯ω = ∇ × ū =∂z − ∂u )zē θ = ωē θ∂r} {{ }ωStudy the right hand side of the inviscid vorticity equation(¯ω · ∇) = ω ∂⇒ (¯ω · ∇)ū = ω )∂(u r (r, z, t)ē r + u z (r, z, t)ē z =r ∂θr ∂θωr∂u r∂θ}{{}=0ē r + ω r u ∂ē rr + ω}{{}∂θ r=ē θ∂u z∂θ}{{}=0The left hand side of the inviscid vorticity equation givesD¯ωDt = ∂ ¯ω∂t + (ū · ∇)¯ω = {¯ω = ωē θ} =ē z + ω r u z∂ē z}{{}∂θ=0( ∂ω∂t + (u r∂This gives that the inviscid vorticity equation now is()∂ω∂t + ∂u r∂r + u ∂z ω = ω ∂z r u r= ω r u rē θ∂r + u ∂z∂z) )ω ē θMultiply by 1 rNotice thatThis means we can write∂∂t( ωr)∂ ω+∂t( 1 (∂u rr r ∂r + u z− ω r 2 u ∂ 1r = ωu r∂r r)+ 1 (∂u rr∂r + u ∂z∂z∂∂zand that) (∂ω + ω u r)ω − ω r 2 u r = 0∂ 1ωu z∂z r = 0)∂r + u ∂ 1z∂z r = 0And thus we have)∂ ω+∂t( 1 ()∂u rr r ∂r + u ∂ ωz∂z r Dt( = D ) ω= 0rExample 3: Inviscid and Irrotational VorticesConsider a circular flow with ū = u θ (r)ē θ . Which vortices are inviscid and which vortices are irrotational?The Navier–Stokes equation for u θFor inviscid flow we require,Make the ansatz u θ = r n ,∂u θ∂t= − 1 ( (∂p 1ρ r ∂r + ν ∂r ∂u )θ− u )θr ∂r ∂r r 21r∂∂r(r ∂u )θ− u θ∂r r 2 = 00 = 1 r∂∂r (rnrn−1 ) − r n−2 = 1 r n2 r n−1 − r n−2 → n 2 − 1 = 0 ⇒ n = ±14

We get the inviscid flow,The vorticity is,u θ (r) =}{{}Ar + B rsolid body rotation }{{}irrotational¯ω = ∇ × ū = {A.32} = 1 r∂∂r (ru θ) ē z¯ω = 0 ⇒ ∂ ∂r (ru θ) = 0 ⇒ u θ = C rConclusion:Irrotational ⇒ inviscidInviscid irrotational5