Vandermonde systems on Gauss-Lobatto Chebyshev nodes

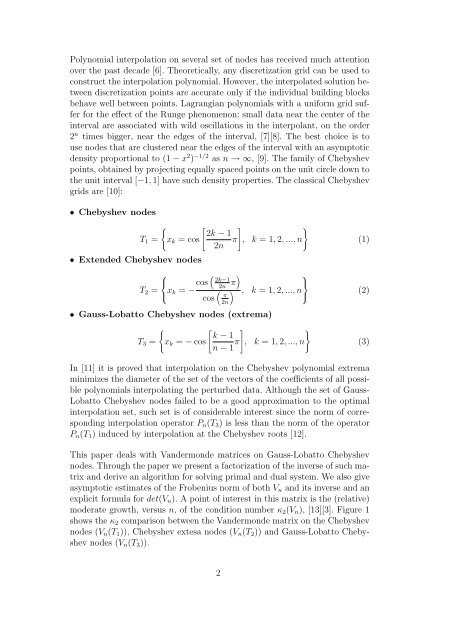
Polynomial interpolation on several set of nodes has received much attentionover the past decade [6]. Theoretically, any discretization grid can be used toconstruct the interpolation polynomial. However, the interpolated solution betweendiscretization points are accurate only if the individual building blocksbehave well between points. Lagrangian polynomials with a uniform grid sufferfor the effect of the Runge phenomenon: small data near the center of theinterval are associated with wild oscillations in the interpolant, on the order2 n times bigger, near the edges of the interval, [7][8]. The best choice is touse nodes that are clustered near the edges of the interval with an asymptoticdensity proportional to (1 − x 2 ) −1/2 as n → ∞, [9]. The family of Chebyshevpoints, obtained by projecting equally spaced points on the unit circle down tothe unit interval [−1, 1] have such density properties. The classical Chebyshevgrids are [10]:• Chebyshev nodesT 1 ={x k = cos• Extended Chebyshev nodes[ ]}2k − 12n π , k = 1, 2,...,n⎧⎨T 2 =⎩ x k = − cos ( ⎫2k−12n π)⎬cos ( ) , k = 1, 2,...,nπ⎭2n• Gauss-Lobatto Chebyshev nodes (extrema)T 3 ={x k = − cos[ ]}k − 1n − 1 π , k = 1, 2,...,n(1)(2)(3)In [11] it is proved that interpolation on the Chebyshev polynomial extremaminimizes the diameter of the set of the vectors of the coefficients of all possiblepolynomials interpolating the perturbed data. Although the set of Gauss-Lobatto Chebyshev nodes failed to be a good approximation to the optimalinterpolation set, such set is of considerable interest since the norm of correspondinginterpolation operator P n (T 3 ) is less than the norm of the operatorP n (T 1 ) induced by interpolation at the Chebyshev roots [12].This paper deals with <strong>Vandermonde</strong> matrices on Gauss-Lobatto Chebyshevnodes. Through the paper we present a factorization of the inverse of such matrixand derive an algorithm for solving primal and dual system. We also giveasymptotic estimates of the Frobenius norm of both V n and its inverse and anexplicit formula for det(V n ). A point of interest in this matrix is the (relative)moderate growth, versus n, of the condition number κ 2 (V n ), [13][3]. Figure 1shows the κ 2 comparison between the <strong>Vandermonde</strong> matrix on the Chebyshevnodes (V n (T 1 )), Chebyshev extesa nodes (V n (T 2 )) and Gauss-Lobatto Chebyshevnodes (V n (T 3 )).2