The Heisenberg Antiferromagnet and the Lanczos Algorithm Abstract

More documents

Recommendations

Info

BSc Final Year Report - Matthew HolmesWe may now introduce the exchange energy term, Eq. (3) for spin interactions [1],E = −2Js ⋅s, (3)12where s z =±/2. Conventionally we work in the z-basis, and +1/2 is described as 'spin up'. Throughoutthe report /2 is absorbed into J. The exchange parameter J is an integral describing the type ofinteraction. The negative sign is included by convention so that in ferromagnets J>0, favouringpolarised pairs, whilst Jˆi⋅Sˆˆ x y z= Sˆi + Sˆj + SˆkS , where [*San*], (4)jS (5)Where i, j, k are orthogonal unit vectors and indicates summation over nearest neighbour pairs.Interactions do occur over a longer range but diminish exponentially with distance and can bedisregarded [9].Anisotropy in the exchange parameter J ij describes interpenetrating sub-lattices of characteristicexchange type. Thus J is different for inter- and intra- lattice interactions [10]. Within a particularlattice then, J ij is isotropic and can be taken outside the summation. Here we consider only isotropicantiferromagnetic interactions along one dimension.The spin operators in Eq. (5) are the Pauli spin matrices [15],01S ˆ x = , ˆ 0− iS y = , ˆ 10 z = 10 i 0 0−1S . (6)We work in the z-basis where the spin vectors (spinors), Eq. (7), are eigenvectors of z [15].+ 1− 0s = , = 01s . (7)Once a basis is chosen we must stick to it due to the non-commutation of the components of witheach other.Real Materials: Quasi-1D Spin ChainsIf magnetic order is strongly confined to particular directions it can be considered to exist on a distinctsub-lattice, and can be modelled in isolation from others. A measure of the isolation is given by theratio J/J´ of the exchange constants within and between sub-lattices [10,12].Purely 1D (or 2D) long range order cannot occur via Eq. (4) [1,7] but some materials with localisedspin-1/2 and appropriate exchange ratios, permit accurate 1D approximations. The quasi-1Dantiferromagnet model can be applied when J/J´~0.05 [12,21]. The antiferromagnetic couplingstrength J has a wide range, from ~1meV in copper benzoate [8], through ~30meV in KCuF 3 [5,3], to~100meV in La 2 CuO 4 [19].4
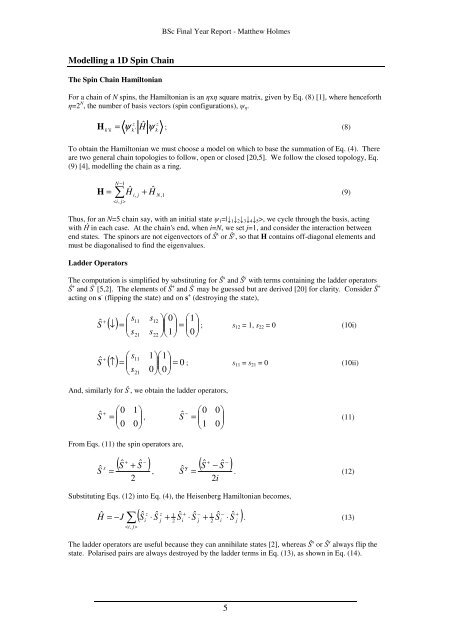
BSc Final Year Report - Matthew HolmesModelling a 1D Spin ChainThe Spin Chain HamiltonianFor a chain of N spins, the Hamiltonian is an x square matrix, given by Eq. (8) [1], where henceforth=2 N , the number of basis vectors (spin configurations), .H ψ H ˆ ψ ; (8)k ' k=zk 'zkTo obtain the Hamiltonian we must choose a model on which to base the summation of Eq. (4). Thereare two general chain topologies to follow, open or closed [20,5]. We follow the closed topology, Eq.(9) [4], modelling the chain as a ring.N 1= − ˆ + Hˆ>HH (9)< i,ji,jN ,1Thus, for an N=5 chain say, with an initial state 1 =| 1 2 3 4 5 >, we cycle through the basis, actingwith in each case. At the chain's end, when i=N, we set j=1, and consider the interaction betweenend states. The spinors are not eigenvectors of x or y , so that H contains off-diagonal elements andmust be diagonalised to find the eigenvalues.Ladder OperatorsThe computation is simplified by substituting for x and y with terms containing the ladder operators + and - [5,2]. The elements of + and - may be guessed but are derived [20] for clarity. Consider +acting on s - (flipping the state) and on s + (destroying the state),ˆs 11 12 ( ↓) = = +S ; s 12 = 1, s 22 = 0 (10i)ˆ ss21ss122011 11 ( ↑) = = 0 s210010+S ; s 11 = s 21 = 0 (10ii)And, similarly for - , we obtain the ladder operators,+ 01− 00Ŝ = , = 0010From Eqs. (11) the spin operators are,( ˆ ˆ −ˆ S )+ + S( SˆSˆ−)S x =, Sˆ+ y −=2Ŝ (11)Substituting Eqs. (12) into Eq. (4), the Heisenberg Hamiltonian becomes,H = −J2iz z + − − +( Sˆ⋅ Sˆ+ Si⋅ Sj+ Si⋅ Sj)i j1 ˆ ˆ 1 ˆ ˆ22< i,j>. (12)ˆ . (13)The ladder operators are useful because they can annihilate states [2], whereas x or y always flip thestate. Polarised pairs are always destroyed by the ladder terms in Eq. (13), as shown in Eq. (14).5
Page 1 and 2: The Heisenberg Antiferromagnet and
Page 3: BSc Final Year Report - Matthew Hol
Page 7 and 8: BSc Final Year Report - Matthew Hol
Page 17: BSc Final Year Report - Matthew Hol

The Heisenberg Antiferromagnet and the Lanczos Algorithm Abstract

Create successful ePaper yourself

Delete template?

Save as template?