MT4514: Graph Theory

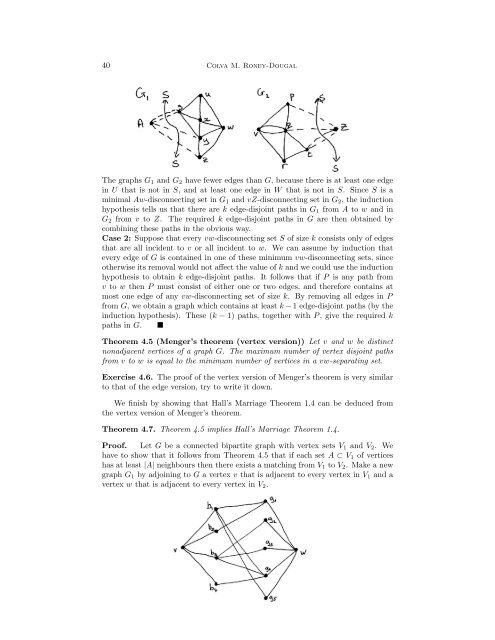
40 Colva M. Roney-DougalThe graphs G 1 and G 2 have fewer edges than G, because there is at least one edgein U that is not in S, and at least one edge in W that is not in S. Since S is aminimal Aw-disconnecting set in G 1 and vZ-disconnecting set in G 2 , the inductionhypothesis tells us that there are k edge-disjoint paths in G 1 from A to w and inG 2 from v to Z. The required k edge-disjoint paths in G are then obtained bycombining these paths in the obvious way.Case 2: Suppose that every vw-disconnecting set S of size k consists only of edgesthat are all incident to v or all incident to w. We can assume by induction thatevery edge of G is contained in one of these minimum vw-disconnecting sets, sinceotherwise its removal would not affect the value of k and we could use the inductionhypothesis to obtain k edge-disjoint paths. It follows that if P is any path fromv to w then P must consist of either one or two edges, and therefore contains atmost one edge of any vw-disconnecting set of size k. By removing all edges in Pfrom G, we obtain a graph which contains at least k − 1 edge-disjoint paths (by theinduction hypothesis). These (k − 1) paths, together with P , give the required kpaths in G. Theorem 4.5 (Menger’s theorem (vertex version)) Let v and w be distinctnonadjacent vertices of a graph G. The maximum number of vertex disjoint pathsfrom v to w is equal to the minimum number of vertices in a vw-separating set.Exercise 4.6. The proof of the vertex version of Menger’s theorem is very similarto that of the edge version, try to write it down.We finish by showing that Hall’s Marriage Theorem 1.4 can be deduced fromthe vertex version of Menger’s theorem.Theorem 4.7. Theorem 4.5 implies Hall’s Marriage Theorem 1.4.Proof. Let G be a connected bipartite graph with vertex sets V 1 and V 2 . Wehave to show that it follows from Theorem 4.5 that if each set A ⊂ V 1 of verticeshas at least |A| neighbours then there exists a matching from V 1 to V 2 . Make a newgraph G 1 by adjoining to G a vertex v that is adjacent to every vertex in V 1 and avertex w that is adjacent to every vertex in V 2 .