14-Stellar Interiors - Physics

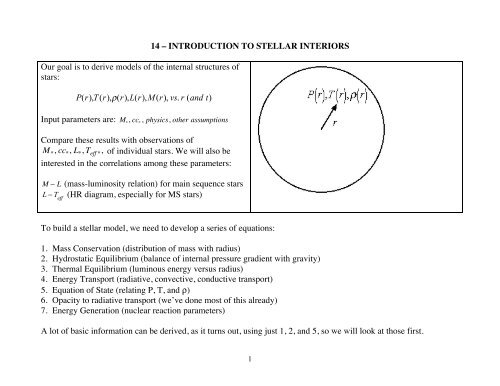
Our goal is to derive models of the internal structures ofstars:P(r),T (r),ρ(r),L(r),M(r), vs. r (and t)Input parameters are: M *, cc *, physics, other assumptionsCompare these results with observations ofM * , cc * , L * , T eff * , of individual stars. We will also beinterested in the correlations among these parameters:M − L (mass-luminosity relation) for main sequence starsL − T eff(HR diagram, especially for MS stars)14 – INTRODUCTION TO STELLAR INTERIORSTo build a stellar model, we need to develop a series of equations:1. Mass Conservation (distribution of mass with radius)2. Hydrostatic Equilibrium (balance of internal pressure gradient with gravity)3. Thermal Equilibrium (luminous energy versus radius)4. Energy Transport (radiative, convective, conductive transport)5. Equation of State (relating P, T, and ρ)6. Opacity to radiative transport (we’ve done most of this already)7. Energy Generation (nuclear reaction parameters)A lot of basic information can be derived, as it turns out, using just 1, 2, and 5, so we will look at those first.1

dpdAdt = P =∞∫ dn v p cos 2 θ dωp=0 p ∫4π∞2π π cos= ∫2 θ sinθdθdφdnp=0 pv p∫φ=0∫θ =04π ∞so, P = 1 3∫ v pdn p=0 p= 1 3ω13∫∞p=0p 2mdn pdn p= n pdp =EQUATION OF STATE FOR PHOTONSh 38π p 2 dp⎡e hν kT⎣⎢( ) −1so, P = 1 ∞ cp8π p 2 dp3∫ = 1 8π k 4 T 4 ∞ x 3 dx0h 3 ⎡e( hν kT ) −1⎤ 3∫h 3 c 3 0e x −1 ⎣⎢ ⎦⎥P = 1 3 aT 4⎛⎝⎜where a = 8π 5 k 4using x = cpkT⎞⎠⎟⎤⎦⎥π 4 1515c 3 h 3 = 7.56x10−15 erg cm −3 K −4⎡note, a = 4σ ⎤⎢⎣ c⎥⎦"radiation constant"ENERGY DENSITY OF PHOTONS∞∞ 8π pU r= ∫ 2 dphνdn0 p= ∫ hν0h 3 ⎛e hν ⎞kT − 1⎝⎜⎠⎟use p = hν cU r=∫∞0let x = hνkT ,U r= 8πhc 3to get8πhν 3 dνc 3 ⎛e hν ⎞kT − 1⎝⎜⎠⎟⎛⎝⎜xkT dxkTso ν = and dν =hhkTh⎞⎠⎟4∫∞U r= 8π 5 k 415c 3 h 3 T 4 = aT 4U r= 3P r0x 3 dxe x − 1= 8πhc 3⎛⎝⎜kTh⎞⎠⎟4π4153

GAS PRESSURE – Ideal Gas LawFor a maxwellian momentum distributiondn p= 4πn( 2πmkT ) − 3 ⎛( 2 ) p 2 e− p2 ⎝⎜ 2mkT⎞⎠⎟dpSo,P = 1 3= 4πn3m∫∞0p 2mdn p4πn( 2πmkT ) − 3 2 p 2 e( 2πmkT ) − 3 ∞2 p 4 e∫0⎛− p2 ⎞⎝⎜ 2mkT ⎠⎟⎛− p2 ⎞⎝⎜ 2mkT ⎠dp⎟dp( ) − 3 24πn 2πmkT=3mP = nkT = ρkTµm H38 π 1 2( 2mkT ) 5 2 = nkTwhere µ = mean atomic weight per particle, m particlem Hm H= 1.66x10 −24 g = 1.66x10 −27 kg4

DEGENERATE FERMIONSWe will look at the case of total degeneracy (partial degeneracy is a little uglier to deal with…).For a simple way to envision this, consider a cell in phase space of volume 1 cm 3 and momentum volume 4π p 2 dp ,2 spin statesdn p=ΔVolume= 4π p2 dph 3n = 8π∫ p 2 dp ( using ΔxΔyΔzΔ 3 p ≥ h 3)h 3If all states are filled for p ≤ p 0and empty for p > p 0(complete degeneracy).n = 8πh 3∫0p 2p 0p 2 dp= 8π3h p 33 0P = 1 p 03∫ dn0 m p= 8π p 0∫ p 4 dp = 8π3h 3 m03h 3 m⎛ 3 ⎞=⎝⎜ 8π ⎠⎟23 h 25m n 5 3⎛ 3 ⎞=⎝⎜ 8π ⎠⎟So, for a completely degenerate, non-relativistic gas,⎧K5 1= 1.00x10 13⎛ ρ ⎞ 3 ⎪P = K 1⎝⎜µ ⎠⎟ electrons → ⎨⎪K 1= 1.00x10 7⎪⎩where µ eand µ pwill be defined later.dyncm−2( g cm −3) 5 3N m−2( kg m −3) 5 323 h2⎡or p 0= 3h3 n ⎤⎢ ⎥⎣⎢8π⎦⎥p 05⎛ ρ ⎞5 m⎜ µm⎟⎝ H ⎠m e forelectrondegeneracy⎫⎪⎬and µ = µ e⎪⎪⎭135 = 8π15mh p 53 053protons → K 1= 5.4x10 19 (cgs)and µ = µ p5

RELATIVISATIC DEGENERACYP = 8π3h 3∫0p 0p 4 dpm= 8πc p 0p 3 ⎛∫ dp replacing p3h 3 0 ⎝⎜m with c ⎞⎠⎟= 2πc3h p 4 3 0= 2πc3h 3ρ 3h 34⎡ ⎤ 3⎢ ⎥ = hc⎣⎢µm H8π ⎦⎥8m H1⎛ 3π ⎞ 3⎛ ρ ⎞⎜ ⎟⎝ ⎠ ⎝⎜µ ⎠⎟m H43⎛ ρ ⎞P = K 2⎝⎜µ ⎠⎟43⎧K 2= 1.241x10 15⎪with ⎨⎪K 2= 1.241x10 10⎪⎩dyncm−2( g cm −3) 4 3N m−2( kg m −3) 5 3⎫⎪⎬ ( electrons and protons)⎪⎪⎭6

EQUATION OF STATE FOR PERFECT GAS WITH ABUNDANCES X, Y, ZIf µ ≡ m m Hwhere:then P g= ρkTµm Hm = µm H=P g= nkT = ρkTmwhere m =∑mass of sample# free particles = mass densityparticle number density =i∑im in iin i∑∑im in in i=∑zn z∑iweight of atom A zm H( )n z ( 1+ η z )A z = atomic weight of element of atomic number zη z = # of electrons contributed per atom of element z (1+ η is # of electrons plus the nucleus).So,1µm H=∑z∑zn z ( 1+ η z )n zA zm HLet the fractional mass density of element z be X z= n z A z m Hρ,=∑zn z ( 1+ η z )ρ1µm H=∑zX zρA zm Hρ( 1+ η z )or 1 µ =X∑zzA z( 1+ η z )8

Let X = mass fraction of HY = mass fraction of HeZ = mass fraction of everything else combined = 1 - X - YVALUES OF X z ( 1+ η z )= 1 A zµTYPE OF GAS H He “Heavies”FULLYX 1+ 1 Y 1+ 2IONIZED= 2X= 3Y 4 Z A z2( )1NEUTRAL X ( 1+ 0)= X1FULLYIONIZED(electrons only)( )1X 1= X( )4Y ( 1+ 0)= Y 4 4Y ( 2)= Y 4 2roughly( )( )A z= Z 2Z 1+ 0A z(>> 1) ≈ 0 usually( )A z= Z 2Z A z2µ FIG=µ NG=µ e FIG− E=12X + 3 Y + 1 Z = 46X + Y + 24 212 ≤ µ FIG ≤ 21 ⎛X + 1 Y unless H is all H 2 → 11X + 1 Y = 2 ⎞X + 1 Y4 ⎝⎜2 4 2 ⎠⎟1X + 1 2 Y + 1 2Z=1− X −Y=2X + 1 1 ≤ µ FIG ≤ 2Where we have used the fact that X +Y + Z =1 to simplify.So, P FIG=NGFIG−EρkTµ FIGNGFIG−Em HEquation of State (Ideal Gas Law)Here, all the compositional effects are included in oneparameter - µ. This will be our Equation of State for mostof the models we will be considering.9

Equation of Mass Continuity (Conservation)If M ris the total mass inside radius r , thenr( )M r = ∫ 4πr 2 ρ( r)dr, dM0r = 4πr 2 dr ρ rSo,dL rdr = 4πr2 ρ rdM rdr = 4πr2 ρ r( )ε rvol.of sphericalshell at r withthicknessdr( )( ) = dL rdr( )( ) = dM rdrEquation of Mass ContinuityAs we will see later, most modern models will use M rinstead of r as the dependent variable in the models(integrating dM rinstead of dr ), so this equation will be used to do the conversion.Equation of Energy ConservationIn a similar manner, we can track the energy generated within a radius r due to the energy being generated per unitmass ε ( r) or per unit volume, which would be ρ( r)ε ( r).∫rL r = 4πr 20ρ( r)ε ( r)dr, dL r = 4πr 2 drρ( r)ε rdL rdr = 4πr2 ρ( r)ε r( ) = dL ( r )drEquation of Energy Conservation( )( )10

Equation of Hydrostatic EquilibriumThe force downward due to gravity on a mass element ofvolume Adr is GM rρAdrr 2The net force outward on the same mass element due to( ) A − PA = dPthe pressure gradient is P + dPdrdr drAFor equilibrium, dPdr drA + GM r ρ ( r )dr= 0r 2So,( )dPdr = −GM rρ rr 2Equation of Hydrostatic EquilibriumOne can also use Poisson’s Equation for a gravitational field with potential ΩIn spherical coordinates,For static fluids,d ⎡dr r 2 dΩ ⎤⎢⎣ dr⎥⎦∇P = −ρ∇Ω,∇ 2 Ω = 4πGρ= 4πGρ →dΩdr = G r 2so dΩdr = − 1 dPρ dr∫r04πr 2 ρ dr = GM rr 2( )or dPdr = − GM r ρ r , as beforer 211