On Nearly Orthogonal Lattice Bases and ... - Researcher - IBM

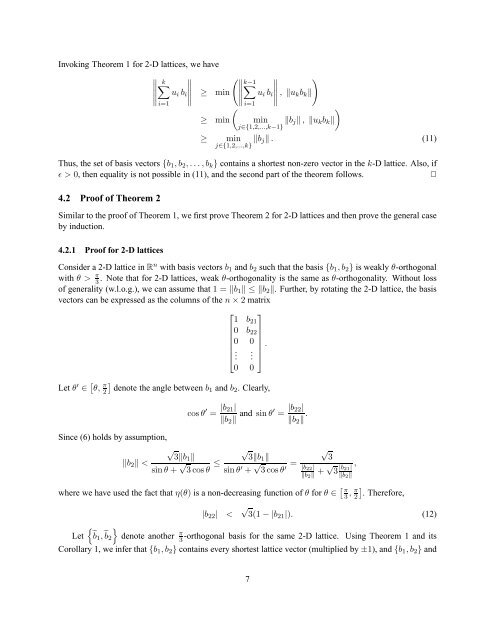
Invoking Theorem 1 for 2-D lattices, we have∥ (∥ ∥ )k∑ ∥∥∥∥ ∥∥∥∥ ∑k−1∥∥∥∥ u i b i ≥ min u i b i , ‖u∥k b k ‖i=1i=1()≥ min min ‖b j‖, ‖u k b k ‖j∈{1,2,...,k−1}≥min ‖b j‖ . (11)j∈{1,2,...,k}Thus, the set of basis vectors {b 1 ,b 2 ,... ,b k } contains a shortest non-zero vector in the k-D lattice. Also, ifɛ > 0, then equality is not possible in (11), and the second part of the theorem follows.✷4.2 Proof of Theorem 2Similar to the proof of Theorem 1, we first prove Theorem 2 for 2-D lattices and then prove the general caseby induction.4.2.1 Proof for 2-D latticesConsider a 2-D lattice in R n with basis vectors b 1 and b 2 such that the basis {b 1 ,b 2 } is weakly θ-orthogonalwith θ > π 3. Note that for 2-D lattices, weak θ-orthogonality is the same as θ-orthogonality. Without lossof generality (w.l.o.g.), we can assume that 1 = ‖b 1 ‖ ≤ ‖b 2 ‖. Further, by rotating the 2-D lattice, the basisvectors can be expressed as the columns of the n × 2 matrix⎡ ⎤1 b 210 b 220 0.⎢ ⎥⎣ . . ⎦0 0Let θ ′ ∈ [ θ, π 2]denote the angle between b1 and b 2 . Clearly,Since (6) holds by assumption,‖b 2 ‖