designing optimal spatial meshes: cutting by parallel trihedra ...
designing optimal spatial meshes: cutting by parallel trihedra ...
designing optimal spatial meshes: cutting by parallel trihedra ...
You also want an ePaper? Increase the reach of your titles
YUMPU automatically turns print PDFs into web optimized ePapers that Google loves.
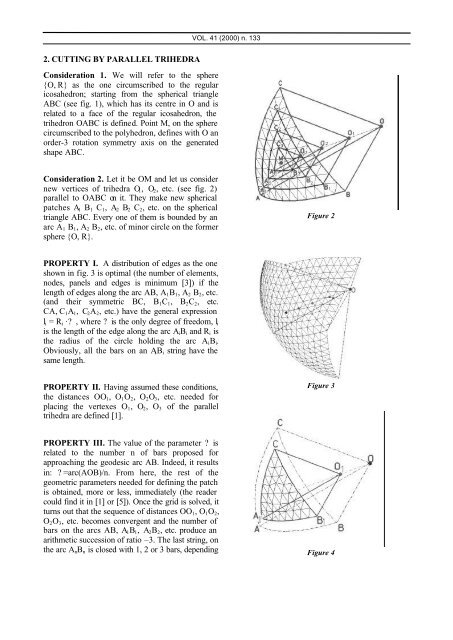
VOL. 41 (2000) n. 1332. CUTTING BY PARALLEL TRIHEDRAConsideration 1. We will refer to the sphere{O, R} as the one circumscribed to the regularicosahedron; starting from the spherical triangleABC (see fig. 1), which has its centre in O and isrelated to a face of the regular icosahedron, thetrihedron OABC is defined. Point M, on the spherecircumscribed to the polyhedron, defines with O anorder-3 rotation symmetry axis on the generatedshape ABC.Consideration 2. Let it be OM and let us considernew vertices of <strong>trihedra</strong> O 1 , O 2 , etc. (see fig. 2)<strong>parallel</strong> to OABC on it. They make new sphericalpatches A 1 B 1 C 1 , A 2 B 2 C 2 , etc. on the sphericaltriangle ABC. Every one of them is bounded <strong>by</strong> anarc A 1 B 1 , A 2 B 2 , etc. of minor circle on the formersphere {O, R}.Figure 2PROPERTY I. A distribution of edges as the oneshown in fig. 3 is <strong>optimal</strong> (the number of elements,nodes, panels and edges is minimum [3]) if thelength of edges along the arc AB, A 1 B 1 , A 2 B 2 , etc.(and their symmetric BC, B 1 C 1 , B 2 C 2 , etc.CA, C 1 A 1 , C 2 A 2 , etc.) have the general expressionl i = R i ·? , where ? is the only degree of freedom, l iis the length of the edge along the arc A i B i and R i isthe radius of the circle holding the arc A i B i .Obviously, all the bars on an A i B i string have thesame length.PROPERTY II. Having assumed these conditions,the distances OO 1 , O 1 O 2 , O 2 O 3 , etc. needed forplacing the vertexes O 1 , O 2 , O 3 of the <strong>parallel</strong><strong>trihedra</strong> are defined [1].Figure 3PROPERTY III. The value of the parameter ? isrelated to the number n of bars proposed forapproaching the geodesic arc AB. Indeed, it resultsin: ? =arc(AOB)/n. From here, the rest of thegeometric parameters needed for defining the patchis obtained, more or less, immediately (the readercould find it in [1] or [5]). Once the grid is solved, itturns out that the sequence of distances OO 1 , O 1 O 2 ,O 2 O 3 , etc. becomes convergent and the number ofbars on the arcs AB, A 1 B 1 , A 2 B 2 , etc. produce anarithmetic succession of ratio –3. The last string, onthe arc A n B n is closed with 1, 2 or 3 bars, depending Figure 4