Distributing labels on infinite trees

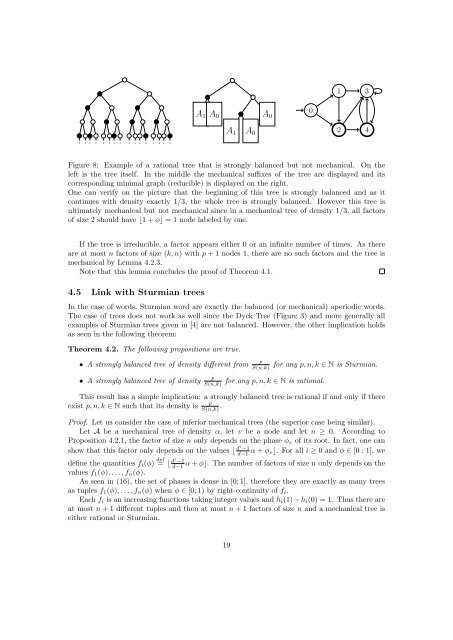
13A 1 A 0A 1 A 0A 0024Figure 8: Example of a rational tree that is strongly balanced but not mechanical. On theleft is the tree itself. In the middle the mechanical suffixes of the tree are displayed and itscorresponding minimal graph (reducible) is displayed on the right.One can verify on the picture that the beginning of this tree is strongly balanced and as itcontinues with density exactly 1/3, the whole tree is strongly balanced. However this tree isultimately mechanical but not mechanical since in a mechanical tree of density 1/3, all factorsof size 2 should have ⌊1 + φ⌋ = 1 node labeled by one.If the tree is irreducible, a factor appears either 0 or an infinite number of times. As thereare at most n factors of size (k, n) with p + 1 nodes 1, there are no such factors and the tree ismechanical by Lemma 4.2.3.Note that this lemma concludes the proof of Theorem 4.1.4.5 Link with Sturmian treesIn the case of words, Sturmian word are exactly the balanced (or mechanical) aperiodic words.The case of trees does not work as well since the Dyck Tree (Figure 3) and more generally allexamples of Sturmian trees given in [4] are not balanced. However, the other implication holdsas seen in the following theorem:Theorem 4.2. The following propositions are true.• A strongly balanced tree of density different from• A strongly balanced tree of densitypS(n,k)pS(n,k)for any p, n, k ∈ N is Sturmian.for any p, n, k ∈ N is rational.This result has a simple implication: a strongly balanced tree is rational if and only if thereexist p, n, k ∈ N such that its density ispS(n,k) .Proof. Let us consider the case of inferior mechanical trees (the superior case being similar).Let A be a mechanical tree of density α, let v be a node and let n ≥ 0. According toProposition 4.2.1, the factor of size n only depends on the phase φ v of its root. In fact, one canshow that this factor only depends on the values ⌊ di −1d−1 α + φ v⌋. For all i ≥ 0 and φ ∈ [0 : 1], wedefine the quantities f i (φ) def= ⌊ di −1d−1α + φ⌋. The number of factors of size n only depends on thevalues f 1 (φ), . . . , f n (φ).As seen in (16), the set of phases is dense in [0; 1], therefore they are exactly as many treesas tuples f 1 (φ), . . . , f n (φ) when φ ∈ [0; 1) by right-continuity of f i .Each f i is an increasing functions taking integer values and h i (1) − h i (0) = 1. Thus there areat most n + 1 different tuples and then at most n + 1 factors of size n and a mechanical tree iseither rational or Sturmian.19