Distributing labels on infinite trees

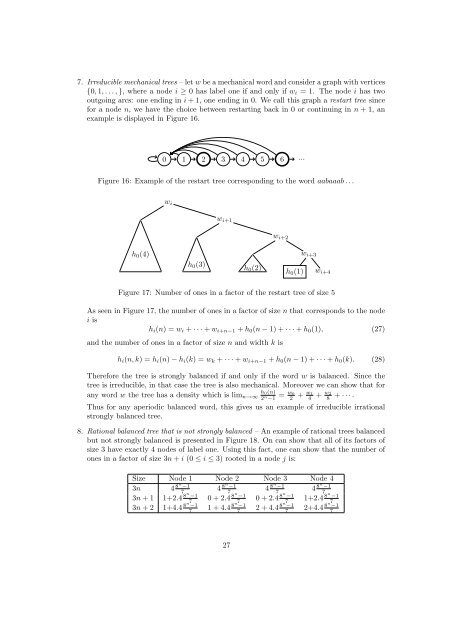
7. Irreducible mechanical trees – let w be a mechanical word and consider a graph with vertices{0, 1, . . . , }, where a node i ≥ 0 has label one if and only if w i = 1. The node i has twooutgoing arcs: one ending in i + 1, one ending in 0. We call this graph a restart tree sincefor a node n, we have the choice between restarting back in 0 or continuing in n + 1, anexample is displayed in Figure 16.0 1 2 3 4 5 6 ...Figure 16: Example of the restart tree corresponding to the word aabaaab . . .h 0 (4)w iw i+1w i+2w i+3w i+4h 0 (3)h 0 (2)h 0 (1)Figure 17: Number of ones in a factor of the restart tree of size 5As seen in Figure 17, the number of ones in a factor of size n that corresponds to the nodei ish i (n) = w i + · · · + w i+n−1 + h 0 (n − 1) + · · · + h 0 (1), (27)and the number of ones in a factor of size n and width k ish i (n, k) = h i (n) − h i (k) = w k + · · · + w i+n−1 + h 0 (n − 1) + · · · + h 0 (k). (28)Therefore the tree is strongly balanced if and only if the word w is balanced. Since thetree is irreducible, in that case the tree is also mechanical. Moreover we can show that forhany word w the tree has a density which is lim 0(n)n→∞ 2 n −1 = w02 + w14 + w28 + · · · .Thus for any aperiodic balanced word, this gives us an example of irreducible irrationalstrongly balanced tree.8. Rational balanced tree that is not strongly balanced – An example of rational trees balancedbut not strongly balanced is presented in Figure 18. On can show that all of its factors ofsize 3 have exactly 4 nodes of label one. Using this fact, one can show that the number ofones in a factor of size 3n + i (0 ≤ i ≤ 3) rooted in a node j is:Size Node 1 Node 2 Node 3 Node 43n 4 8n −174 8n −174 8n −174 8n −173n + 1 1+2.4 8n −170 + 2.4 8n −170 + 2.4 8n −171+2.4 8n −173n + 2 1+4.4 8n −171 + 4.4 8n −172 + 4.4 8n −172+4.4 8n −1727