838 Foundations of Trigonometryfind the angle of inclination, labeled θ below, satisfies tan(θ) = 612 = 1 2. Since θ is an acute angle,we can use the arctangent function and we find θ = arctan ( 12)radians ≈ 26.56 ◦ .6 feetθ12 feet<strong>10.6</strong>.4 Solving Equations Using the <strong>Inverse</strong> <strong>Trigonometric</strong> <strong>Functions</strong>.In <strong>Section</strong>s 10.2 and 10.3, we learned how to solve equations like sin(θ) = 1 2for angles θ andtan(t) = −1 for real numbers t. In each case, we ultimately appealed to the Unit Circle and reliedon the fact that the answers corresponded to a set of ‘common angles’ listed on page 724. If, onthe other hand, we had been asked to find all angles with sin(θ) = 1 3or solve tan(t) = −2 forreal numbers t, we would have been hard-pressed to do so. With the introduction of the inversetrigonometric functions, however, we are now in a position to solve these equations. A good parallelto keep in mind is how the square root function can be used to solve certain quadratic equations.<strong>The</strong> equation x 2 = 4 is a lot like sin(θ) = 1 2in that it has friendly, ‘common value’ answers x = ±2.<strong>The</strong> equation x 2 = 7, on the other hand, is a lot like sin(θ) = 1 3 . We know8 there are answers, butwe can’t express them using ‘friendly’ numbers. 9 To solve x 2 = 7, we make use of the square rootfunction and write x = ± √ 7. We can certainly approximate these answers using a calculator, butas far as exact answers go, we leave them as x = ± √ 7. In the same way, we will use the arcsinefunction to solve sin(θ) = 1 3, as seen in the following example.Example <strong>10.6</strong>.7. Solve the following equations.1. Find all angles θ for which sin(θ) = 1 3 .2. Find all real numbers t for which tan(t) = −23. Solve sec(x) = − 5 3for x.Solution.1. If sin(θ) = 1 3, then the terminal side of θ, when plotted in standard position, intersects theUnit Circle at y = 1 3. Geometrically, we see that this happens at two places: in Quadrant Iand Quadrant II. If we let α denote the acute solution to the equation, then all the solutions8 How do we know this again?9 This is all, of course, a matter of opinion. For the record, the authors find ± √ 7 just as ‘nice’ as ±2.
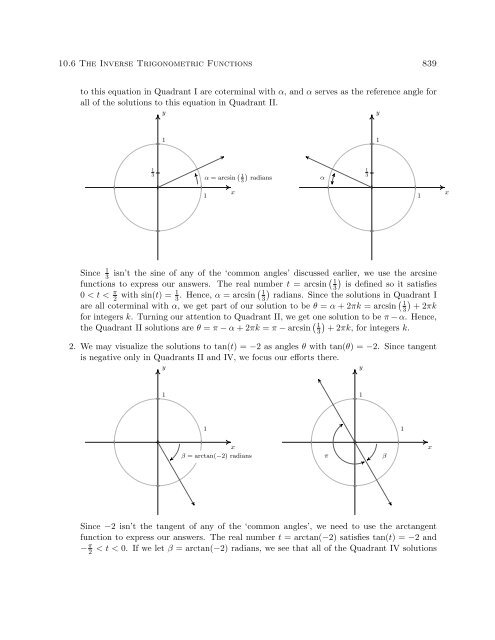
<strong>10.6</strong> <strong>The</strong> <strong>Inverse</strong> <strong>Trigonometric</strong> <strong>Functions</strong> 839to this equation in Quadrant I are coterminal with α, and α serves as the reference angle forall of the solutions to this equation in Quadrant II.yy1113α = arcsin ` 1´ 13 radians3αxx11Since 1 3isn’t the sine of any of the ‘common angles’ discussed earlier, we use the arcsinefunctions to express our answers. <strong>The</strong> real number t = arcsin ( 13)is defined so it satisfies0 < t < π 2 with sin(t) = 1 3 . Hence, α = arcsin ( 13)radians. Since the solutions in Quadrant Iare all coterminal with α, we get part of our solution to be θ = α + 2πk = arcsin ( 13)+ 2πkfor integers k. Turning our attention to Quadrant II, we get one solution to be π − α. Hence,the Quadrant II solutions are θ = π − α + 2πk = π − arcsin ( 13)+ 2πk, for integers k.2. We may visualize the solutions to tan(t) = −2 as angles θ with tan(θ) = −2. Since tangentis negative only in Quadrants II and IV, we focus our efforts there.yy1111xβ = arctan(−2) radiansπβxSince −2 isn’t the tangent of any of the ‘common angles’, we need to use the arctangentfunction to express our answers. <strong>The</strong> real number t = arctan(−2) satisfies tan(t) = −2 and− π 2< t < 0. If we let β = arctan(−2) radians, we see that all of the Quadrant IV solutions