integration

368 INTEGRAL CALCULUS
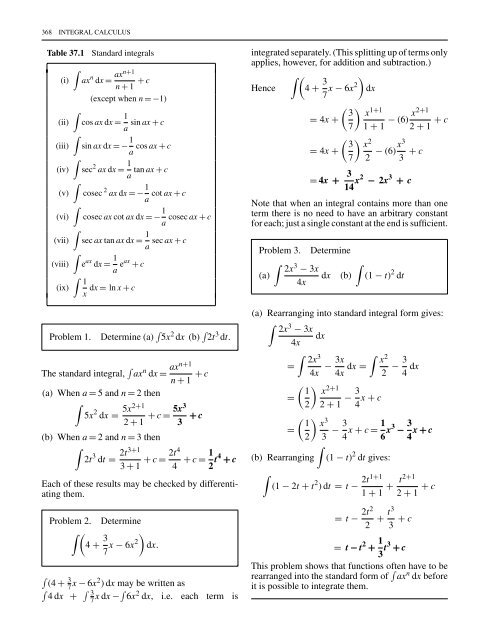
Table 37.1
Standard integrals
∫
(i) ax n dx = axn+1
n + 1 + c
(except when n =−1)
∫
(ii) cos ax dx = 1 sin ax + c
a
∫
(iii) sin ax dx =− 1 cos ax + c
a
∫
(iv) sec 2 ax dx = 1 tan ax + c
a
∫
(v) cosec 2 ax dx =− 1 cot ax + c
a
∫
(vi) cosec ax cot ax dx =− 1 cosec ax + c
a
∫
(vii) sec ax tan ax dx = 1 sec ax + c
a
∫
(viii) e ax dx = 1 a eax + c
∫ 1
(ix) dx = ln x + c
x
Problem 1.
Determine (a) ∫ 5x 2 dx (b) ∫ 2t 3 dt.
The standard integral, ∫ ax n dx = axn+1
n + 1 + c
(a) When a = 5 and n = 2 then
∫
5x 2 dx = 5x2+1
2 + 1 + c = 5x3
3 + c
(b) When a = 2 and n = 3 then
∫
2t 3 dt = 2t3+1
3 + 1 + c = 2t4
4 + c = 1 2 t4 + c
Each of these results may be checked by differentiating
them.
Problem 2. Determine
∫ (4 + 3 )
7 x − 6x2 dx.
∫
(4 +
3
7
x − 6x 2 )dx may be written as
∫
4dx +
∫ 37
x dx − ∫ 6x 2 dx, i.e. each term is
integrated separately. (This splitting up of terms only
applies, however, for addition and subtraction.)
∫
Hence
(4 + 3 )
7 x − 6x2 dx
( 3 x
1+1
= 4x +
7)
1 + 1
− (6)
x2+1
2 + 1 + c
( 3 x
2
= 4x +
7)
2 − (6)x3 3 + c
= 4x + 3
14 x2 − 2x 3 + c
Note that when an integral contains more than one
term there is no need to have an arbitrary constant
for each; just a single constant at the end is sufficient.
Problem 3. Determine
∫ 2x 3 ∫
− 3x
(a)
dx (b) (1 − t) 2 dt
4x
(a) Rearranging into standard integral form gives:
∫ 2x 3 − 3x
dx
4x
∫ 2x
3
=
4x − 3x ∫ x
2
4x dx = 2 − 3 4 dx
( 1 x
2+1
=
2)
2 + 1 − 3 4 x + c
( 1 x
3
=
2)
3 − 3 4 x + c = 1 6 x3 − 3 4 x + c
∫
(b) Rearranging (1 − t) 2 dt gives:
∫
(1 − 2t + t 2 )dt = t − 2t1+1
1 + 1 + t2+1
2 + 1 + c
= t − 2t2
2 + t3 3 + c
= t − t 2 + 1 3 t3 + c
This problem shows that functions often have to be
rearranged into the standard form of ∫ ax n dx before
it is possible to integrate them.