Chapter 5

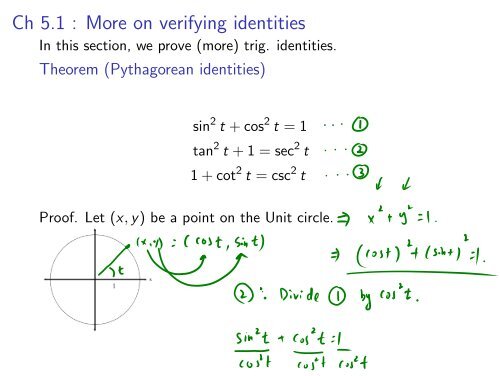
Ch 5.1 : More on verifying identities
In this section, we prove (more) trig. identities.
Theorem (Pythagorean identities)
sin 2 t + cos 2 t = 1
tan 2 t + 1 = sec 2 t
1 + cot 2 t = csc 2 t
Proof. Let (x, y) be a point on the Unit circle.

Verifying symmetry of sine, cosine, and tangent functions
Theorem (Identities due to symmetry)
sin(−t) = − sin(t)
cos(−t) = cos(t)
tan(−t) = − tan(t)
Proof. Let (x, y) be a point on the Unit circle.

Example 1
Prove that
(1 − tan t) 2 = sec 2 (t) + 2 tan(−t)

Example 2
Prove that
sin 2 t · tan 2 t = tan 2 t − sin 2 t

Example 3
Prove that
cos t
1 + sec t
= 1 − cos t
tan 2 t

Example 4
Prove that
sec x
sin x
sin x
−
sec x = tan2 x + cos2 x
tan x

Class Exercises
Verify the following identities.
1−cos x sin x
1. sin x = 1+cos x
1 2. cos2 1 + x sin2 x = csc2 x sec2 x
3.
= csc x
1+cos(−x)
sin x−cos x sin(−x)

5.2: The Sum and Difference Identities
Theorem
sin(α ± β) = sin α cos β ± cos α sin β
Note: ± ⇒ ±
Examples:
◮ sin( π
4
◮ sin( π
4
◮ sin( 5π
12
+ π
6
− π
6
) =
◮ sin(− π
12
) = sin( π
4
) =
) =
) cos( π
6
) + cos( π
4
π ) sin( 6 ) =

5.2: The Sum and Difference Identities
Theorem
cos(α ± β) = cos α cos β ∓ sin α sin β
Note: ± ⇒ ∓
Examples:
◮ cos( π
4
◮ cos( π
4
◮ cos( 5π
12
◮ cos( π
12
+ π
6
− π
6
) =
) =
) = cos( π
4
) =
) cos( π
6
) − sin( π
4
π ) sin( 6 ) =

Cofunction identities
Theorem (The Cofunction Identities)
cos( π
− t) = sin t, sin(π − t) = cos t
2 2
Example) Using the cofunction identities, verify that
cos( π π
6 ) = sin( 3 ).
Proof(s) of theorem.

Sum and difference identities for Tangent
Theorem
Example) tan( π
12 ) =
Proof of theorem:
tan(α ± β) =
tan α ± tan β
1 ∓ tan α tan β

Example 3
Given sin α = 5
13 with the terminal side in the 1st quadrant, and
tan β = − 24
7 with the terminal side in the 2nd quadrant, compute
the value of cos(α + β).

Example 4
Use the sum or difference identity for tangent to find the exact
value of tan 11π
12 .

Example 6
Prove that
sin(α + β) sin(α − β) = sin 2 α − sin 2 β

Class Exercise
Given α and β are acute angles, with cos α = 8
find
1. sin(α + β)
2. cos(α − β)
3. tan(α + β)
17
and sec β = 25
7 ,

Ch 5.3 : The Double-Angle and Half-Angle Identities
Theorem (The Double-Angle Identities)
◮ cos(2α) = cos 2 α − sin 2 α = 1 − 2 sin 2 α = 2 cos 2 α − 1
◮ sin(2α) = 2 sin α cos α
◮ tan(2α) =
2 tan α
1−tan 2 α
Let’s verify these identities.

Example 2 : Combining sum and double-angle identities
Prove that
Proof:
cos 3θ = 4 cos 3 θ − 3 cos θ

Example 3 : Using double-angle identity
Find the exact value of sin π π
8 cos 8 .

The power reduction identities
Theorem
cos 2 α = 1+cos(2α)
2 , sin 2 α = 1−cos(2α)
2 , tan 2 α = 1−cos(2α)
1+cos(2α)
Proof.

Example 4
Prove that
8 sin 4 x = 3 − 4 cos(2x) + cos(4x)

Half-Angle Identities
Theorem
◮ cos( u
2
◮ sin( u
2
1+cos u
) = ±
2
1−cos u
) = ± 2
◮ tan( u
1−cos u
2 ) = ± 1+cos u
Proof.
= 1−cos u
sin u
= sin u
1+cos u

Example 5
Use the half-angle identities to find exact values for a) sin π
12 and
b) tan π
12 .

Example 6
For cos θ = − 7
25
sin θ
2
and b) cos θ
2 .
, and θ in the 3rd quadrant, find exact values of a)

Ch 5.4 : The Product-to-Sum Identities
Proof.
cos α cos β = 1
[cos(α + β) + cos(α − β)]
2
sin α sin β = 1
[cos(α − β) − cos(α + β)]
2

The Product-to-Sum Identities
Proof.
sin α cos β = 1
[sin(α + β) + sin(α − β)]
2
cos α sin β = 1
[sin(α + β) − sin(α − β)]
2

Example
Rewrite the following product as a sum using the product-to-sum
identities.
2 sin(3x + y) cos(3x − y)

The Sum-to-Product Identities (for cosines)
Proof.
u + v u − v
cos u + cos v = 2 cos cos
2
2
u + v u − v
cos u − cos v = −2 sin sin
2
2

The Sum-to-Product Identities (for sines)
Proof.
u + v u − v
sin u + sin v = 2 sin cos
2
2
u + v u − v
sin u − sin v = 2 cos sin
2
2

Example
Write the sum as a product using a sum-to-product identity.
sin(14k) + sin(41k)

Class Exercise
Verify the following identity.
sin(m) + sin(n) m + n
= tan
cos(m) + cos(n) 2