Extrasolar Moons as Gravitational Microlenses Christine Liebig

CHAPTER 4. CHOICE OF SCENARIOS 35
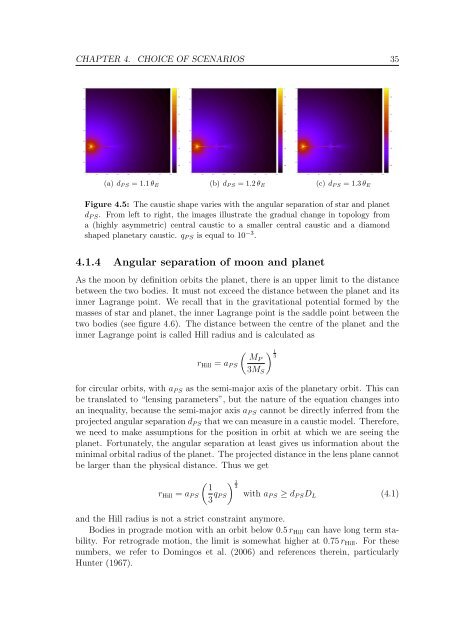
(a) dP S = 1.1 θE (b) dP S = 1.2 θE (c) dP S = 1.3 θE
Figure 4.5: The caustic shape varies with the angular separation of star and planet
dP S. From left to right, the images illustrate the gradual change in topology from
a (highly asymmetric) central caustic to a smaller central caustic and a diamond
shaped planetary caustic. qP S is equal to 10 −3 .
4.1.4 Angular separation of moon and planet
As the moon by definition orbits the planet, there is an upper limit to the distance
between the two bodies. It must not exceed the distance between the planet and its
inner Lagrange point. We recall that in the gravitational potential formed by the
masses of star and planet, the inner Lagrange point is the saddle point between the
two bodies (see figure 4.6). The distance between the centre of the planet and the
inner Lagrange point is called Hill radius and is calculated as
rHill = aP S
MP
3MS
for circular orbits, with aP S as the semi-major axis of the planetary orbit. This can
be translated to “lensing parameters”, but the nature of the equation changes into
an inequality, because the semi-major axis aP S cannot be directly inferred from the
projected angular separation dP S that we can measure in a caustic model. Therefore,
we need to make assumptions for the position in orbit at which we are seeing the
planet. Fortunately, the angular separation at least gives us information about the
minimal orbital radius of the planet. The projected distance in the lens plane cannot
be larger than the physical distance. Thus we get
rHill = aP S
1
3 qP
1
3
S
1
3
with aP S ≥ dP SDL
(4.1)
and the Hill radius is not a strict constraint anymore.
Bodies in prograde motion with an orbit below 0.5 rHill can have long term stability.
For retrograde motion, the limit is somewhat higher at 0.75 rHill. For these
numbers, we refer to Domingos et al. (2006) and references therein, particularly
Hunter (1967).