x - MathnMind

Your Notes 

9.1 

Apply the Distance and 

Midpoint Formulas 

Goal p Find the length and midpoint of a line segment. 

VOCABULARY 

Distance formula 

Midpoint formula 

THE DISTANCE FORMULA 

The distance d between (x 1 , y 1 ) and (x 2 , y 2 ) is 

d 5 Ï }}} 

( ) 2 1 ( ) 2 . 

Example 1 Find the distance between two points 

Find the distance between (25, 23) and (3, 6). 

Let (x1 , y1 ) 5 (25, 23) and (x2 , y2 ) 5 (3, 6). 

d 5 Ï }}} 

(x 2 2 x 1 ) 2 1 (y 2 2 y 1 ) 2 

5 Ï }}} 

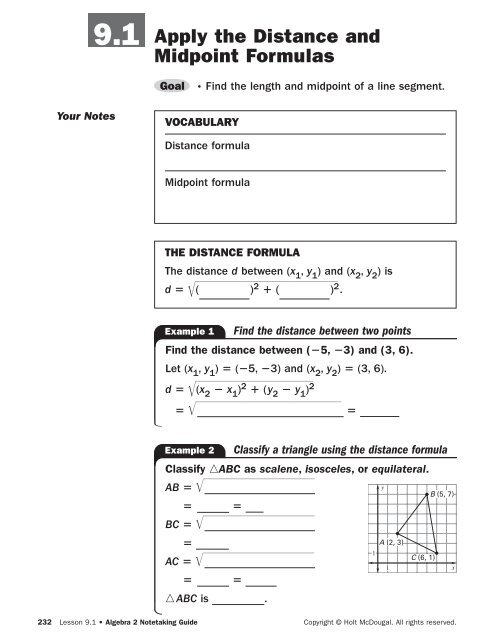
Example 2 Classify a triangle using the distance formula 

Classify nABC as scalene, isosceles, or equilateral. 

AB 5 Ï }} 

y 

5 5 

BC 5 Ï }} 

5 

AC 5 Ï }} 

5 5 

nABC is . 

5 

1 

A (2, 3) 

1 

C (6, 1) 

B (5, 7) 

232 Lesson 9.1 Algebra 2 Notetaking Guide Copyright © Holt McDougal. All rights reserved. 

x

Your Notes 

9.1 

Apply the Distance and 

Midpoint Formulas 

Goal p Find the length and midpoint of a line segment. 

VOCABULARY 

Distance formula The formula used to find the 

distance between two points A(x 1 , y 1 ) and B(x 2 , y 2 ) 

Midpoint formula The formula that describes the 

midpoint of the line segment joining A(x 1 , y 1 ) and 

B(x 2 , y 2 ) 

THE DISTANCE FORMULA 

The distance d between (x1 , y1 ) and (x2 , y2 ) is 

d 5 Ï }}} 

( x2 2 x1 ) 2 1 ( y2 2 y1 ) 2 . 

Example 1 Find the distance between two points 

Find the distance between (25, 23) and (3, 6). 

Let (x1 , y1 ) 5 (25, 23) and (x2 , y2 ) 5 (3, 6). 

d 5 Ï }}} 

(x 2 2 x 1 ) 2 1 (y 2 2 y 1 ) 2 

5 Ï }}} 

(3 2 (25)) 2 1 (6 2 (23)) 2 5 Ï } 

145 

Example 2 Classify a triangle using the distance formula 

Classify nABC as scalene, isosceles, or equilateral. 

AB 5 Ï }} 

(5 2 2) 2 1 (7 2 3) 2 

y 

5 Ï } 

25 5 5 

BC 5 Ï }} 

(6 2 5) 2 1 (1 2 7) 2 

5 Ï } 

37 

AC 5 Ï }} 

(6 2 2) 2 1 (1 2 3) 2 

5 Ï } 

20 5 2 Ï } 

5 

nABC is scalene . 

1 

A (2, 3) 

1 

C (6, 1) 

B (5, 7) 


x

Your Notes 

Checkpoint Complete the following exercises. 

1. Find the distance between (27, 3) and (5, 22). 

2. The vertices of a triangle are T(2, 1), U(4, 6), and 

V(7, 3). Classify nTUV as scalene, isosceles, or 

equilateral. 

THE MIDPOINT FORMULA 

A line segment’s midpoint is from the 

segment’s endpoints. The midpoint formula describes 

the of a line segment joining A(x1 , y1 ) and 

B(x2 , y2 ) as follows: 

M 1 x1 1 x2 } 

2 , y1 1 y2 } 

2 2 

In words, each coordinate of M is the of the 

corresponding coordinates of A and B. 

Example 3 Find the midpoint of a line segment 

Find the midpoint of the line 

segment joining (26, 5) and 

(2, 23). 

Let (x1 , y1 ) 5 (26, 5) and 

(x2 , y2 ) 5 ( 2, 23). 

1 x1 1 x2 } 

2 , y1 1 y2 } 

Midpoint M 

(−6, 5) 

2 2 5 1 , 2 

5 ( , ) 

2 

y 

2 

(2, −3) 

Copyright © Holt McDougal. All rights reserved. Lesson 9.1 Algebra 2 Notetaking Guide 233 

x

Your Notes 


1. Find the distance between (27, 3) and (5, 22). 

13 

2. The vertices of a triangle are T(2, 1), U(4, 6), and 

V(7, 3). Classify nTUV as scalene, isosceles, or 

equilateral. 

isosceles 

THE MIDPOINT FORMULA 

A line segment’s midpoint is equidistant from the 

segment’s endpoints. The midpoint formula describes 

the midpoint of a line segment joining A(x1 , y1 ) and 

B(x2 , y2 ) as follows: 

M 1 x1 1 x2 } 

2 , y1 1 y2 } 

2 2 

In words, each coordinate of M is the mean of the 

corresponding coordinates of A and B. 

Example 3 Find the midpoint of a line segment 

Find the midpoint of the line 

segment joining (26, 5) and 

(2, 23). 

Let (x1 , y1 ) 5 (26, 5) and 

(x2 , y2 ) 5 ( 2, 23). 

1 x1 1 x2 } 

2 , y1 1 y2 } 

2 2 5 1 

26 1 2 

} 

2 

5 ( 22 , 1 ) 

Midpoint M 

(−6, 5) 

, 5 1 (23) 

} 

2 2 

2 

y 

2 

(2, −3) 


x

Your Notes 

Homework 

Example 4 Find a perpendicular bisector 

Write an equation for the perpendicular bisector of the 

line segment joining A(24, 1) and B(2, 3). 

Solution 

1. Find the midpoint of the line segment. 

1 x1 1 x2 } 

2 , y1 1 y2 } 

2 2 5 1 

2. Calculate the slope of 

, 2 5 ( , ) 

} 

AB . 

m 5 y 2 2 y 1 

} 

x 2 2 x 1 5 5 5 

3. Find the slope of the perpendicular bisector. 

2 

1 

} 5 5 

m 

4. Use point-slope form: y 2 5 (x 2 ( )) or 

y 5 . 

An equation for the perpendicular bisector of AB is 

y 5 . 


3. Find the midpoint of the line segment joining (26, 5) 

and (1, 1). 

4. Write an equation for the perpendicular bisector of 

the line segment joining A(25, 6) and (3, 22). 

234 Lesson 9.1 Algebra 2 Notetaking Guide Copyright © Holt McDougal. All rights reserved.

Your Notes 

Homework 

Example 4 Find a perpendicular bisector 

Write an equation for the perpendicular bisector of the 

line segment joining A(24, 1) and B(2, 3). 

Solution 

1. Find the midpoint of the line segment. 

1 x1 1 x2 } 

2 , y1 1 y2 24 1 2 1 1 3 

} 

2 2 5 1 } , } 

2 2 2 5 ( 21 , 2 ) 

2. Calculate the slope of } 

AB . 

m 5 y 2 2 y 1 

} 

x 2 2 x 1 5 

3 2 1 

} 

2 2 (24) 

2 

5 } 5 

6 1 

} 

3 

3. Find the slope of the perpendicular bisector. 

2 

1 

} 5 2 

m 1 

1 

} 

} 

3 

5 23 

4. Use point-slope form: y 2 2 5 23 (x 2 ( 21 )) or 

y 5 23x 2 1 . 

An equation for the perpendicular bisector of AB is 

y 5 23x 2 1 . 


3. Find the midpoint of the line segment joining (26, 5) 

and (1, 1). 

1 2 

5 

} , 3 

2 2 

4. Write an equation for the perpendicular bisector of 

the line segment joining A(25, 6) and (3, 22). 

y 5 x 1 3 


Your Notes 

9.2 Graph and Write Equations of 

Parabolas 

Goal p Graph and write equations of parabolas that open 

left or right. 

VOCABULARY 

Focus 

Directrix 

STANDARD EQUATION OF A PARABOLA WITH VERTEX 

AT THE ORIGIN 

The standard form of the equation of a parabola with 

vertex at (0, 0) is as follows: 

Equation Focus Directrix Axis of Symmetry 

x2 5 4py (0, p) y 5 Vertical ( ) 

y2 5 4px (p, 0) x 5 Horizontal ( ) 

Copyright © Holt McDougal. All rights reserved. Lesson 9.2 Algebra 2 Notetaking Guide 235

Your Notes 


Parabolas 

Goal p Graph and write equations of parabolas that open 

left or right. 

VOCABULARY 

Focus A fixed point that lies on the axis of 

symmetry of a parabola 

Directrix A line that is perpendicular to the axis of 

symmetry of a parabola 

STANDARD EQUATION OF A PARABOLA WITH VERTEX 

AT THE ORIGIN 

The standard form of the equation of a parabola with 

vertex at (0, 0) is as follows: 

Equation Focus Directrix Axis of Symmetry 

x2 5 4py (0, p) y 5 2p Vertical ( x 5 0 ) 

y2 5 4px (p, 0) x 5 2p Horizontal ( y 5 0 ) 


Your Notes 

Example 1 Graph an equation of a parabola 

Graph x 5 1 

} 

2 y 2 . Identify the focus, directrix, and axis of 

symmetry. 

1. Rewrite the equation in standard form. 

x 5 1 

} 

2 y 2 Write original equation. 

5 Multiply each side by . 

2. Identify the focus, directrix, and axis of symmetry. The 

equation has the form y2 5 4px where p 5 . The 

focus is (p, 0), or 1 , 0 2 . The directrix is x 5 2p, or 

x 5 . Because y is squared, the axis of symmetry 

is the . 

y 

3. Draw the parabola by making 

a table of values and plotting 

1 

points. Because p 0, the 

parabola opens to the . 

1 

x 

So, use only x-values. 

x 1 2 3 4 5 

y 

Checkpoint Complete the following exercise. 

1. Graph y 5 2 1 

} x 

4 2 . Identify the focus, directrix, and 

axis of symmetry. 


1 

y 

1 

x

Your Notes 

Example 1 Graph an equation of a parabola 

Graph x 5 1 

} 

2 y 2 . Identify the focus, directrix, and axis of 

symmetry. 


x 5 1 

} y 

2 2 Write original equation. 

2x 5 y2 Multiply each side by 2 . 

2. Identify the focus, directrix, and axis of symmetry. The 

equation has the form y2 5 4px where p 5 1 

} . The 

2 

focus is (p, 0), or 1 1 

} , 0 

2 2 . The directrix is x 5 2p, or 

x 5 2 

1 

} . Because y is squared, the axis of symmetry 

2 

is the x-axis . 

y 

3. Draw the parabola by making 

a table of values and plotting 

points. Because p > 0, the 

parabola opens to the right . 

So, use only positive x-values. 

(0, 0) 

x 5 − 1 

2 

1 1 

2 , 0 

1 

( ) 

x 1 2 3 4 5 

y 61.41 62 62.45 62.83 63.16 


1. Graph y 5 2 1 

} x 

4 2 . Identify the focus, directrix, and 

axis of symmetry. 

focus: (0, 21); directrix: 

y 5 1; axis of symmetry: 

y-axis, x 5 0 

(0, −1) 

y 

y 5 1 

1 

(0, 0) 


1 

x 

x

Your Notes 

Homework 

Example 2 Write an equation of a parabola 

Write an equation of the parabola shown. 

(0, 0) 

1 

y 

Directrix 

1 


x 

Solution 

The graph shows that the vertex is and the 

directrix is y 5 2p 5 . Substitute for p in the 

standard form of the equation of a parabola. 

Standard form, axis of symmetry 

Substitute for p. 

Simplify. 


2. Write the standard form of the equation of the 

parabola with vertex at (0, 0) and the directrix 

x 5 2 3 

} . 

4

Your Notes 

Homework 

Example 2 Write an equation of a parabola 

Write an equation of the parabola shown. 

(0, 0) 

1 

y 

Directrix 

1 


x 

Solution 

The graph shows that the vertex is (0, 0) and the 

directrix is y 5 2p 5 3 . Substitute 23 for p in the 

standard form of the equation of a parabola. 

x 2 5 4py Standard form, vertical axis of symmetry 

x 2 5 4(23)y Substitute for p. 

x 2 5 212y Simplify. 


2. Write the standard form of the equation of the 

parabola with vertex at (0, 0) and the directrix 

x 5 2 3 

} . 

4 

y 2 5 3x

Your Notes 

9.3 Graph and Write Equations 

of Circles 

Goal p Graph and write equations of circles. 

VOCABULARY 

Circle 

Center 

Radius 

STANDARD EQUATION OF A CIRCLE WITH CENTER AT 

THE ORIGIN 

The standard form of the equation of a circle with 

center at (0, 0) and radius r is as follows: 

x2 1 y2 5 

Example 1 Graph an equation of a circle 

Graph y 2 5 2x 2 1 16. Identify the radius of the circle. 

Solution 

1. Rewrite the equation y2 5 2x2 1 16 in standard form 

as . 

y 

2. Identify the center and radius. 

From the equation, the graph is 

a circle centered at the origin 

1 

with radius r 5 5 . 

1 

x 

3. Draw the circle. First plot several 

convenient points that are 4 units 

from the origin, such as (0, ), 

(4, ), (0, ), and (24, ). Then draw the circle 

that passes through the points. 


Your Notes 


of Circles 

Goal p Graph and write equations of circles. 

VOCABULARY 

Circle The set of all points (x, y) that are 

equidistant from a fixed point 

Center The fixed point that is equidistant from all 

the points on a circle 

Radius The distance r between the center and any 

point (x, y) on a circle 

STANDARD EQUATION OF A CIRCLE WITH CENTER AT 

THE ORIGIN 

The standard form of the equation of a circle with 

center at (0, 0) and radius r is as follows: 

x2 1 y2 5 r2 Example 1 Graph an equation of a circle 

Graph y 2 5 2x 2 1 16. Identify the radius of the circle. 

Solution 

1. Rewrite the equation y2 5 2x2 1 16 in standard form 

as x2 1 y2 5 16 . 

y 

2. Identify the center and radius. 

From the equation, the graph is 

a circle centered at the origin 

1 

with radius r 5 Ï 1 

x 

} 

16 5 4 . 

3. Draw the circle. First plot several 

convenient points that are 4 units 

from the origin, such as (0, 4 ), 

(4, 0 ), (0, 24 ), and (24, 0 ). Then draw the circle 

that passes through the points. 


Your Notes 

Checkpoint Graph the equation. Identify the radius. 

1. x 2 5 4 2 y 2 

Example 2 Write an equation of a circle 

The point (23, 4) lies on a circle whose center is the 

origin. Write the standard form of the equation of the 

circle. 

The circle’s radius r must be the distance between the 

center and (23, 4). Use the distance formula. 

r 5 Ï }}} 

( ) 2 1 ( ) 2 

5 Ï } 

5 Ï } 

5 

Use the standard form with r 5 

of the circle. 

to write an equation 

x2 1 y2 5 r2 Standard form 

x2 1 y2 5 2 Substitute for r. 

x2 1 y2 5 Simplify. 

Example 3 Find a tangent line 

Write an equation of the line tangent to the circle 

x2 1 y2 5 17 at (4, 21). 

A line tangent to a circle and the radius to the point of 

tangency are perpendicular. The radius with endpoint 

(4, 21) has slope m 5 5 , so the slope 

of the tangent line at (4, 21) is the negative reciprocal 

of , or . An equation of the tangent line is as 

follows: 

y 1 5 (x 2 ) Point-slope form 

y 5 Solve for y. 


1 

y 

1 

x

Your Notes 

Checkpoint Graph the equation. Identify the radius. 

1. x2 5 4 2 y2 r 5 2 

Example 2 Write an equation of a circle 

The point (23, 4) lies on a circle whose center is the 

origin. Write the standard form of the equation of the 

circle. 

The circle’s radius r must be the distance between the 

center and (23, 4). Use the distance formula. 

r 5 Ï }}} 

( 23 2 0 ) 2 1 ( 4 2 0 ) 2 

5 Ï } 

9 1 16 5 Ï } 

25 5 5 

Use the standard form with r 5 5 to write an equation 

of the circle. 

x2 1 y2 5 r2 Standard form 

x2 1 y2 5 5 2 Substitute for r. 

x2 1 y2 5 25 Simplify. 

Example 3 Find a tangent line 

Write an equation of the line tangent to the circle 

x2 1 y2 5 17 at (4, 21). 

A line tangent to a circle and the radius to the point of 

tangency are perpendicular. The radius with endpoint 

21 2 0 1 

(4, 21) has slope m 5 } 5 2 } , so the slope 

4 2 0 4 

of the tangent line at (4, 21) is the negative reciprocal 

of 2 

1 

} , or 4 . An equation of the tangent line is as 

4 

follows: 

y 1 1 5 4 (x 2 4 ) Point-slope form 

y 5 4x 2 17 Solve for y. 


1 

y 

1 

x

Your Notes 

Homework 

Example 4 Write a circular model 

Lighthouse The beam from Oak Island Lighthouse in North 

Carolina can be seen for up to 24 miles. You are 18 miles 

east and 9 miles south of the lighthouse. Can you see the 

lighthouse beam? 

Solution 

1. Write an inequality for the region lit by the beam. 

This region is all the points that satisfy the following 

inequality: x2 1 y2 < 2 

2. Substitute the coordinates (18, 9) into the inequality. 

x 2 1 y 2 < 2 Inequality 

< 2 Substitute for x and y. 

The inequality is . 

You see the lighthouse beam. 


2. Write the standard form of the equation of the circle 

with center at the origin that passes through the 

point (6, 23). 

3. Write an equation of the line tangent to the circle 

x 2 1 y 2 5 34 at (23, 25). 

4. From Example 4, suppose you are 16 miles east and 

19 miles south of the lighthouse. Can you see the 



Your Notes 

Homework 

Example 4 Write a circular model 

Lighthouse The beam from Oak Island Lighthouse in North 

Carolina can be seen for up to 24 miles. You are 18 miles 

east and 9 miles south of the lighthouse. Can you see the 


Solution 

1. Write an inequality for the region lit by the beam. 

This region is all the points that satisfy the following 

inequality: x2 1 y2 < 24 2 

2. Substitute the coordinates (18, 9) into the inequality. 

x2 1 y2 < 24 2 Inequality 

182 1 92 < 24 2 Substitute for x and y. 

405 < 576 The inequality is true . 

You can see the lighthouse beam. 


2. Write the standard form of the equation of the circle 

with center at the origin that passes through the 

point (6, 23). 

x 2 1 y 2 5 45 

3. Write an equation of the line tangent to the circle 

x 2 1 y 2 5 34 at (23, 25). 

y 5 2 3 

} x 2 

5 34 

} 

5 

4. From Example 4, suppose you are 16 miles east and 

19 miles south of the lighthouse. Can you see the 


no 


Your Notes 


Ellipses 

Goal p Graph and write equations of ellipses. 

VOCABULARY 

Ellipse 

Foci 

Vertices 

Major axis 

Center 

Co-vertices 

Minor axis 

STANDARD EQUATION OF AN ELLIPSE WITH CENTER 

AT THE ORIGIN 

Equation Major Axis Vertices Co-Vertices 

x2 y2 

} 1 } 

2 

a 

x2 y2 

} 1 } 

2 

5 1 Horizontal (6 , 0) (0, 6 ) 

b2 5 1 

b a2 Vertical (0, 6 ) (6 , 0) 

The major and minor axes are of lengths 2a and 2b, 

respectively, where a > b > 0. The foci of the ellipse 

lie on the major axis at a distance of c units from the 

center, where c2 5 . 


Your Notes 


Ellipses 

Goal p Graph and write equations of ellipses. 

VOCABULARY 

Ellipse The set of all points P such that the sum 

of the distances between P and two fixed points, 

called the foci, is a constant 

Foci Two fixed points in an ellipse 

Vertices The points at which the line through the 

foci intersect the ellipse 

Major axis The line segment that joins the vertices 

Center The midpoint of the major axis 

Co-vertices The points of intersection of an ellipse 

and the line perpendicular to the major axis at the 

center 

Minor axis The line segment that joins the 

co-vertices 

STANDARD EQUATION OF AN ELLIPSE WITH CENTER 

AT THE ORIGIN 

Equation Major Axis Vertices Co-Vertices 

x2 y2 

} 1 } 

2 

a 

x2 y2 

} 1 } 

2 

5 1 Horizontal (6 a , 0) (0, 6 b ) 

b2 5 1 Vertical (0, 6 a ) (6 b , 0) 

b a2 The major and minor axes are of lengths 2a and 2b, 

respectively, where a > b > 0. The foci of the ellipse 

lie on the major axis at a distance of c units from the 

center, where c2 5 a2 2 b2 . 


Your Notes 

Example 1 Graph an equation of an ellipse 

Graph the equation 9x2 1 36y2 5 324. Identify the 

vertices, co-vertices, and foci of the ellipse. 


9x2 1 36y2 5 324 Write original equation. 

1 5 Divide each side by . 

1 5 1 Simplify. 

2. Identify the vertices, co-vertices, 

and foci. Note that a 2 5 

and b 2 5 , so a 5 and 

b 5 . The denominator of the 

x 2 -term is that 

of the y 2 -term, so the major axis 

is . The vertices of 

the ellipse are at (6a, 0) 5 (6 , 0). The co-vertices 

are at (0, 6b) 5 (0, 6 ). Find the foci. 

c2 5 a2 2 b2 5 5 , so c 5 Ï } 

. 

The foci are at (6 Ï } 

, 0), or about (6 , 0). 

3. Draw the ellipse that passes through each vertex and 

co-vertex. 

Example 2 Write an equation given a vertex and a co-vertex 

Write an equation of the ellipse that has a vertex at 

(0, 7), a co-vertex at (24, 0), and center at (0, 0). 

Sketch the ellipse as a check for your 

final equation. By symmetry, the 

y 

ellipse must also have a vertex at 

(0, ) and a co-vertex at ( , 0). 

Because the vertex is on the 

2 

2 

x 

and the co-vertex is on the , 

the major axis is with 

a 5 , and the minor axis is with b 5 . 

An equation is 5 1, or 5 1. 


2 

y 

2 

x

Your Notes 

Example 1 Graph an equation of an ellipse 

Graph the equation 9x2 1 36y2 5 324. Identify the 

vertices, co-vertices, and foci of the ellipse. 


9x2 1 36y2 5 324 Write original equation. 

9x2 

} 

342 

x2 

} 

36 

1 36y2 

} 

324 

1 y2 

} 

9 

5 324 

} 

324 

5 1 Simplify. 

2. Identify the vertices, co-vertices, 

and foci. Note that a 2 5 36 

and b 2 5 9 , so a 5 6 and 

b 5 3 . The denominator of the 

x 2 -term is greater than that 

of the y 2 -term, so the major axis 

is horizontal . The vertices of 

Divide each side by 324 . 

(− 27, 0) 

(−6, 0) 

2 

(0, −3) 

y 

(0, 3) 

2 

( 27, 0) 

the ellipse are at (6a, 0) 5 (6 6 , 0). The co-vertices 

are at (0, 6b) 5 (0, 6 3 ). Find the foci. 

c2 5 a2 2 b2 5 62 2 32 5 27 , so c 5 Ï } 

27 . 

The foci are at (6 Ï } 

27 , 0), or about (6 5.2 , 0). 

x 

(6, 0) 

3. Draw the ellipse that passes through each vertex and 

co-vertex. 

Example 2 Write an equation given a vertex and a co-vertex 


(0, 7), a co-vertex at (24, 0), and center at (0, 0). 

Sketch the ellipse as a check for your 

final equation. By symmetry, the 

(0, 7) 

y 

ellipse must also have a vertex at 

(0, 27 ) and a co-vertex at ( 4 , 0). 

Because the vertex is on the y-axis 

(4, −0) 

2 

2 

(4, 0) 

x 

and the co-vertex is on the x-axis , 

the major axis is vertical with 

(0, −7) 

a 5 7 , and the minor axis is horizontal with b 5 4 . 

An equation is x2 

} 

4 

y2 

x2 

1 } 5 1, or } 

2 2 

7 

16 

1 y2 

} 

49 

5 1. 


Your Notes 

Homework 

Example 3 Write an equation given a vertex and a focus 


(26, 0) and a focus at (5, 0). 

Solution 

Make a sketch of the ellipse. 

Because the vertex and focus lie 

on the , the major axis is 

, with a 5 and 

c 5 . To find b, use the equation 

c 2 5 a 2 2 b 2 . 

5 2 b 2 

b 2 5 2 5 

b 5 

An equation is 5 1, or 5 1. 

Checkpoint Graph the equation. Identify the vertices, 

co-vertices, and foci of the ellipse. 

1. x2 1 y2 

} 5 1 

25 

Checkpoint Write an equation of the ellipse with the 

given characteristics and center at (0, 0). 

2. Vertex: (29, 0) 3. Vertex: (0, 7) 

Co-vertex: (0, 4) Focus: (0, 23) 


2 

2 

y 

y 

2 

2 

x 

x

Your Notes 

Homework 

Example 3 Write an equation given a vertex and a focus 


(26, 0) and a focus at (5, 0). 

Solution 

Make a sketch of the ellipse. 

Because the vertex and focus lie 

on the x-axis , the major axis is 

horizontal , with a 5 6 and 

c 5 5 . To find b, use the equation 

c 2 5 a 2 2 b 2 . 

5 2 5 6 2 2 b 2 

b2 5 62 2 52 5 11 

b 5 Ï } 

11 

An equation is x2 

} 

6 

1. x2 1 y2 

} 5 1 

25 

(−6, 0) 

y2 

x2 

1 } 5 1, or } 

2 2 

( Ï } 


11 ) 

2 

y 

2 

(6, 0) 

x 

(0, 5) (0, −5) 

36 

1 y2 

} 

11 

( 0, 24) 

2 

(−1, 0) 

5 1. 


co-vertices, and foci of the ellipse. 

vertices: (0, 65) 

co-vertices: (61, 0) 

foci: (0, 64.90) 

( 0, − 24) 

y 

(0, 5) 

(1, 0) 

2 

(0, −5) 

Checkpoint Write an equation of the ellipse with the 

given characteristics and center at (0, 0). 

2. Vertex: (29, 0) 3. Vertex: (0, 7) 

Co-vertex: (0, 4) Focus: (0, 23) 

x2 

} 

81 

1 y2 

} 

16 

5 1 x2 

} 

40 

y2 

1 } 5 1 

49 

x

Your Notes 


of Hyperbolas 

Goal p Graph and write equations of hyperbolas. 

VOCABULARY 

Hyperbola 

Foci 

Vertices 

Transverse Axis 

Center 

STANDARD EQUATION OF A HYPERBOLA WITH 

CENTER AT THE ORIGIN 

Equation Transverse Axis Asymptotes Vertices 

x2 y2 

} 2 } 

2 

a 

y2 x2 

2 } 

2 

5 1 Horizontal y 5 6 x (6 , 0) 

b2 } 5 1 

a b2 Vertical y 5 6 x (0, 6 ) 

The foci lie on the transverse axis, c units from the 

center, where c2 5 . 


Your Notes 


of Hyperbolas 

Goal p Graph and write equations of hyperbolas. 

VOCABULARY 

Hyperbola The set of all points P such that the 

difference of the distances between P and two 

fixed points, called the foci, is a constant 

Foci Two fixed points in a hyperbola 

Vertices The points of intersection of a hyperbola 

and the line through the foci 

Transverse Axis The line segment that connects the 

vertices of a hyperbola 

Center The midpoint of the transverse axis 

STANDARD EQUATION OF A HYPERBOLA WITH 

CENTER AT THE ORIGIN 

Equation Transverse Axis Asymptotes Vertices 

x2 y2 

} 2 } 

2 

a 

b 

5 1 Horizontal y 5 6 } x (6 a , 0) 

b2 a 

y2 x2 

a 

} 2 } 5 1 Vertical y 5 6 } x (0, 6 a ) 

a2 b2 b 

The foci lie on the transverse axis, c units from the 

center, where c2 5 a2 1 b2 . 


Your Notes 

Example 1 Graph an equation of a hyperbola 

Graph 36y 2 2 9x 2 5 324. Identify the vertices, foci, 

and asymptotes of the hyperbola. 

Solution 

1. Rewrite the equation in standard 

form. 

5 1 

2. Identify the vertices, foci, and 

asymptotes. Note that a2 5 

and b2 5 , so a 5 and b 5 . The y2-term is , so the transverse axis is and 

the vertices are (0, 6 ). Find the foci. 

c2 5 a2 1 b2 5 5 , so c 5 Ï } 

The foci are at (0, 6 Ï } 

) ø (0, 66.7). 

The asymptotes are y 5 6 

a 

} x, or y 5 

b 

. 

3. Draw the hyperbola. Draw a rectangle centered at the 

origin that is 2a 5 units high and 2b 5 units 

wide. The asymptotes pass through opposite corners 

of the rectangle. Then, draw the hyperbola passing 

through the vertices and approaching the asymptotes. 


foci, and asymptotes of the hyperbola. 

1. x2 

} 

49 

2 y2 

} 

9 5 1 


2 

y 

2 

2 

y 

2 

x 

x

Your Notes 

Example 1 Graph an equation of a hyperbola 

Graph 36y 2 2 9x 2 5 324. Identify the vertices, foci, 

and asymptotes of the hyperbola. 

Solution 

1. Rewrite the equation in standard 

form. 

y2 

} 

9 

2 x2 

} 

36 

5 1 

2. Identify the vertices, foci, and 

asymptotes. Note that a 2 5 9 

( 0, 45) 


2 

(0, −3) 

y 

(0, 3) 

2 

( 0, − 45) 

and b2 5 36 , so a 5 3 and b 5 6 . The y2-term is positive , so the transverse axis is vertical and 

the vertices are (0, 6 3 ). Find the foci. 

c2 5 a2 1 b2 5 32 1 62 5 45 , so c 5 Ï } 

45 

The foci are at (0, 6 Ï } 

45 ) ø (0, 66.7). 

The asymptotes are y 5 6 

a 

} x, or y 5 6 

b 1 

} x . 

2 

3. Draw the hyperbola. Draw a rectangle centered at the 

origin that is 2a 5 6 units high and 2b 5 12 units 

wide. The asymptotes pass through opposite corners 

of the rectangle. Then, draw the hyperbola passing 

through the vertices and approaching the asymptotes. 


foci, and asymptotes of the hyperbola. 

1. x2 

} 

49 

2 y2 

} 

9 5 1 

vertices: (67, 0) 

foci: (67.62, 0) 

asymptotes: y 5 6 3 

} x 

7 

(−7.62, 0) 

(−7, 0) 

2 

y 

2 

(7, 0) 

x 

(7.62, 0) 

x

Your Notes 

Example 2 Write an equation of a hyperbola 

Write an equation of the 

y 

hyperbola with foci at 

(25, 0) and (5, 0) and 

(−4, 0) (4, 0) 

vertices at (24, 0) and 

(−5, 0) 

2 

(5, 0) 

(4, 0). 

The foci and vertices lie on the 

-axis equidistant from the 

origin, so the transverse axis is 

6 x 

and the center is the origin. The foci are 

each units from the center, so c 5 . The vertices 

are each units from the center, so a 5 . 

Because c2 5 a2 1 b2 , you have b2 5 c2 2 a2 . Find b2 . 

b2 5 c2 2 a2 5 5 

Because the transverse axis is horizontal, the standard 

form of the equation is as follows: 

5 1 Substitute 4 for a and 9 for b 2 . 

5 1 Simplify. 

Checkpoint Write an equation of the hyperbola with 

the given foci and vertices. 

2. Foci: (0, 28), (0, 8) 

Vertices: (0, 25), (0, 5) 


Your Notes 

Example 2 Write an equation of a hyperbola 

Write an equation of the 

y 

hyperbola with foci at 

(25, 0) and (5, 0) and 

(−4, 0) (4, 0) 

vertices at (24, 0) and 

(−5, 0) 

2 

(5, 0) 

(4, 0). 

The foci and vertices lie on the 

x -axis equidistant from the 

origin, so the transverse axis is 

6 x 

horizontal and the center is the origin. The foci are 

each 5 units from the center, so c 5 5 . The vertices 

are each 4 units from the center, so a 5 4 . 

Because c2 5 a2 1 b2 , you have b2 5 c2 2 a2 . Find b2 . 

b2 5 c2 2 a2 5 52 2 42 5 9 

Because the transverse axis is horizontal, the standard 

form of the equation is as follows: 

x2 y2 

2 } 

2 

} 

4 

x2 

} 

16 

2 y2 

} 

9 

9 5 1 Substitute 4 for a and 9 for b2 . 

5 1 Simplify. 

Checkpoint Write an equation of the hyperbola with 

the given foci and vertices. 

2. Foci: (0, 28), (0, 8) 

Vertices: (0, 25), (0, 5) 

y2 

} 

25 

x2 

2 } 5 1 

39 


Your Notes 

Homework 

Example 3 Solve a multi-step problem 

Lamp The diagram shows the hyperbolic cross section 

of a lamp. Write an equation for the cross section of the 

lamp. The lamp is 10 inches high. How wide is the base? 

Solution 

1. From the diagram, a 5 

and b 5 . 

Because the transverse axis 

is , an equation 

for the cross section of 

the lamp 

is 5 1, 

or 5 1. 

(−2, 0) 


3. Write an equation for the hyperbolic cross section of 

the lamp in Example 3 if the vertices are at (63, 0) 

and the foci are at (65, 0). If the lamp is 15 inches 

high, how wide is the base? 


(0, 4) 

(0, −4) 

2. Find the x-coordinate at the lamp’s bottom edge. 

Because the lamp is 10 inches tall, substitute 

y 5 into the equation and solve. 

x 2 5 

5 1 

x ø 

So, the lamp has a width of 2x or 

2( ) 5 inches. 

y 

(2, 0) 

x

Your Notes 

Homework 

Example 3 Solve a multi-step problem 

Lamp The diagram shows the hyperbolic cross section 

of a lamp. Write an equation for the cross section of the 

lamp. The lamp is 10 inches high. How wide is the base? 

Solution 

1. From the diagram, a 5 2 

and b 5 4 . 

Because the transverse axis 

is horizontal , an equation 

for the cross section of 

the lamp 

is x2 y2 

} 2 } 5 1, 

22 42 or x2 

} 

4 


3. Write an equation for the hyperbolic cross section of 

the lamp in Example 3 if the vertices are at (63, 0) 

and the foci are at (65, 0). If the lamp is 15 inches 

high, how wide is the base? 

x2 

} 

9 

y2 

2 } 5 1 

16 

12.75 in. 

2 y2 

} 

16 

5 1. 

(−2, 0) 


(0, 4) 

(0, −4) 

2. Find the x-coordinate at the lamp’s bottom edge. 

Because the lamp is 10 inches tall, substitute 

y 5 5 into the equation and solve. 

x2 

} 

4 

2 52 

} 

16 

5 1 

x 2 5 10.25 

x ø 3.20 

So, the lamp has a width of 2x or 

2( 3.20 ) 5 6.40 inches. 

y 

(2, 0) 

x

Your Notes 

9.6 Translate and Classify Conic 

Sections 

Goal p Translate conic sections. 

VOCABULARY 

Conic sections 

General second-degree equation 

Discriminant 

STANDARD FORM OF EQUATIONS OF TRANSLATED 

CONICS 

In the following equations, the point (h, k) is the vertex 

of the parabola and the center of the other conics. 

Circle (x 2 h) 2 1 (y 2 k) 2 5 r2 Parabola (y 2 k) 2 5 4p(x 2 h) Horizontal axis 

(x 2 h) 2 5 4p(y 2 k) Vertical axis 

Ellipse 

Hyperbola 

(x 2 h)2 

} 

a 

(x 2 h)2 

} 

b 

(y 2 k)2 

1 } 

2 

(y 2 k)2 

1 } 

2 

(x 2 h)2 

} 

a 

(y 2 k)2 

} 

a 

(y 2 k)2 

2 } 

2 

(x 2 h)2 

2 } 

2 

5 1 Horizontal axis 

b2 5 1 Vertical axis 

a2 5 1 Horizontal axis 


b2 248 Lesson 9.6 Algebra 2 Notetaking Guide Copyright © Holt McDougal. All rights reserved.

Your Notes 

9.6 Translate and Classify Conic 

Sections 

Goal p Translate conic sections. 

VOCABULARY 

Conic sections The intersection of a plane and a 

double-napped cone 

General second-degree equation An equation of the 

form Ax 2 1 Bxy 1 Cy 2 1 Dx 1 Ey 1 F 5 0 

Discriminant The expression B 2 2 4AC for the 

equation Ax 2 1 Bxy 1 Cy 2 1 Dx 1 Ey 1 F 5 0, 

used to identify a conic section 

STANDARD FORM OF EQUATIONS OF TRANSLATED 

CONICS 

In the following equations, the point (h, k) is the vertex 

of the parabola and the center of the other conics. 

Circle (x 2 h) 2 1 (y 2 k) 2 5 r2 Parabola (y 2 k) 2 5 4p(x 2 h) Horizontal axis 

(x 2 h) 2 5 4p(y 2 k) Vertical axis 

Ellipse 

Hyperbola 

(x 2 h)2 

} 

a 

(x 2 h)2 

} 

b 

(y 2 k)2 

1 } 

2 

(y 2 k)2 

1 } 

2 

(x 2 h)2 

} 

a 

(y 2 k)2 

} 

a 

(y 2 k)2 

2 } 

2 

(x 2 h)2 

2 } 

2 

5 1 Horizontal axis 


a2 5 1 Horizontal axis 


b2 248 Lesson 9.6 Algebra 2 Notetaking Guide Copyright © Holt McDougal. All rights reserved.

Your Notes 

Example 1 Graph the equation of a translated circle 

Graph (x 1 3) 2 1 (y 2 2) 2 5 4. 

1. Compare the given equation to 

the standard form of an equation 

of a circle. The graph is a circle 

with center at (h, k) 5 ( , ) 

and radius r 5 Ï } 

5 . 

2. Plot the center. Then plot several 

points that are each units 

from the center: 


5 

5 

5 

5 

3. Draw a circle through the points. 

Example 2 Graph the equation of a translated hyperbola 

Graph 

(y 1 2)2 

} 

16 

2 (x 2 1)2 

} 

4 

5 1. 

1. Compare the given equation to the standard forms 

of equations of hyperbolas. The graph is a hyperbola 

with a transverse axis. The center is at 

(h, k) 5 ( , ). Because a2 5 and 

b2 5 , you know that a 5 and b 5 . 

2. Plot the center, vertices, and foci. 

y 

The vertices lie a 5 units 

above and below the center, at 

2 

( , ) and ( , ). 

2 

x 

Because c2 5 a2 1 b2 5 

the foci lie c 5 Ï 

, 

} 

ø 

units above and below the center, 

at ( , ) and ( , ). 

3. Draw the hyperbola. Draw a rectangle centered at 

( , ) that is 2a 5 units high and 2b 5 

units wide. Draw the asymptotes through the opposite 

corners of the rectangle. Then draw the hyperbola so 

that it passes through the vertices and approaches the 

asymptotes. 

1 

y 

1 x

Your Notes 

Example 1 Graph the equation of a translated circle 

Graph (x 1 3) 2 1 (y 2 2) 2 5 4. 

1. Compare the given equation to 

the standard form of an equation 

of a circle. The graph is a circle 

with center at (h, k) 5 ( 23 , 2 ) 

and radius r 5 Ï } 

4 5 2 . 

2. Plot the center. Then plot several 

points that are each 2 units 

from the center: 

(23 1 2, 2) 5 (21, 2) 

(23 2 2, 2) 5 (25, 2) 

(23, 2 1 2) 5 (23, 4) 

(23, 2 2 2) 5 (23, 0) 

3. Draw a circle through the points. 

Graph 

(y 1 2)2 

} 

16 

2 (x 2 1)2 

} 

4 

5 1. 

(−5, 2) 

(−3, 4) 

(−3, 2) 

(−3, 0) 

(−1, 2) 

Example 2 Graph the equation of a translated hyperbola 

1. Compare the given equation to the standard forms 

of equations of hyperbolas. The graph is a hyperbola 

with a vertical transverse axis. The center is at 

(h, k) 5 ( 1 , 22 ). Because a2 5 16 and 

b2 5 4 , you know that a 5 4 and b 5 2 . 

2. Plot the center, vertices, and foci. 

y 

(1, 2) 

The vertices lie a 5 4 units 

above and below the center, at 

2 

( 1 , 2 ) and ( 1 , 26 ). 

2 

x 

Because c (1, −2) 

(1, −6) 

2 5 a2 1 b2 5 20 , 

the foci lie c 5 Ï } 

20 ø 4.47 

units above and below the center, 

at ( 1 , 2.47 ) and ( 1 , 26.47 ). 

3. Draw the hyperbola. Draw a rectangle centered at 

( 1 , 22 ) that is 2a 5 8 units high and 2b 5 4 

units wide. Draw the asymptotes through the opposite 

corners of the rectangle. Then draw the hyperbola so 

that it passes through the vertices and approaches the 

asymptotes. 


1 

y 

1 x

Your Notes 

Write an equation of the parabola whose vertex is at 

(2, 1) and whose focus is at (5, 1). 

1. Determine the form of the 

equation. Sketch the parabola. 

The parabola opens to the 

y 

and has the form 

2 

(y 2 k) 2 

x 

2 Example 3 Write an equation of a translated parabola 

5 4p(x 2 h) where 

p 0. 

2. Identify h and k. The vertex is at 

(2, 1), so h 5 and k 5 . 

3. Find p. The vertex (2, 1) and focus (5, 1) both lie on 

the line , so the distance between them is 

⏐p⏐ 5 ⏐ ⏐ 5 , and p 5 or p 5 . 

Because p 0, it follows that p 5 , so 4p 5 . 

The equation is . 


1. Graph (x 2 2) 2 1 (y 1 3) 2 5 9. 

Identify the center and radius. 

2. Graph 

(x 1 3)2 

} 

9 

(y 2 1)2 

2 } 5 1. 

25 

3. Write an equation of the parabola whose vertex is at 



1 

y 

1 

2 

y 

2 

x 

x

Your Notes 

Example 3 Write an equation of a translated parabola 

Write an equation of the parabola whose vertex is at 



equation. Sketch the parabola. 

The parabola opens to the 

right and has the form 

(y 2 k) 2 5 4p(x 2 h) where 

p > 0. 

2. Identify h and k. The vertex is at 

(2, 1), so h 5 2 and k 5 1 . 

2 

y 

2 

(2, 1) 

(5, 1) 

3. Find p. The vertex (2, 1) and focus (5, 1) both lie on 

the line y 5 1 , so the distance between them is 

⏐p⏐ 5 ⏐ 5 2 2 ⏐ 5 3 , and p 5 3 or p 5 23 . 

Because p > 0, it follows that p 5 3 , so 4p 5 12 . 

The equation is (y 2 1) 2 5 12(x 2 2) . 


1. Graph (x 2 2) 2 1 (y 1 3) 2 5 9. 

Identify the center and radius. 

center: (2, 23) 

radius: 3 

2. Graph 

(x 1 3)2 

} 

9 

(y 2 1)2 

2 } 5 1. 

25 

1 

y 

1 

(2, −3) 

3. Write an equation of the parabola whose vertex is at 


(x 1 3) 2 5 8(y 1 1) 


2 

y 

2 

x 

x 

x

Your Notes 

Example 4 Write an equation of a translated ellipse 

Write an equation of the ellipse with foci at (22, 3) and 

(4, 3) and co-vertices at (1, 4) and (1, 2). 


equation. First sketch the 

ellipse. The foci lie on the 

major axis, so the axis is 

y 

. The equation 

1 

has the form: 

1 

x 

(x 2 h)2 (y 2 k)2 

} 1 } 5 1 

a2 b2 2. Identify h and k by finding the center, which is halfway 

between the foci (or the co-vertices). 

(h, k) 5 1 , 2 5 ( , ) 

3. Find b, the distance between a co-vertex and the 

center, and c, the distance between a focus and 

the center: 

b 5 ⏐ ⏐ 5 , c 5 ⏐ ⏐ 5 3. 

4. Find a. For an ellipse, 

a2 5 b2 1 c2 5 1 5 , so a 5 Ï } 

. 

The standard form of the equation is 



. 

4. Write an equation of the ellipse with foci at (23, 0) 

and (23, 26) and co-vertices at (21, 23) and 

(25, 23).

Your Notes 

Example 4 Write an equation of a translated ellipse 

Write an equation of the ellipse with foci at (22, 3) and 

(4, 3) and co-vertices at (1, 4) and (1, 2). 


y 

equation. First sketch the 

ellipse. The foci lie on the 

major axis, so the axis is 

(−2, 3) 

(1, 4) 

(4, 3) 

horizontal . The equation 

(1, 2) 

1 

has the form: 

1 

x 

(x 2 h)2 (y 2 k)2 

} 1 } 5 1 

a2 b2 2. Identify h and k by finding the center, which is halfway 

between the foci (or the co-vertices). 

22 1 4 3 1 3 

(h, k) 5 1 } , } 

2 2 2 5 ( 1 , 3 ) 

3. Find b, the distance between a co-vertex and the 

center, and c, the distance between a focus and 

the center: 

b 5 ⏐ 4 2 3 ⏐ 5 1 , c 5 ⏐ 22 2 1 ⏐ 5 3. 

4. Find a. For an ellipse, 

a2 5 b2 1 c2 5 12 1 32 5 10 , so a 5 Ï } 

10 . 

The standard form of the equation is 

(x 2 1)2 

} 

10 

(y 2 3)2 

1 } 5 1 . 

1 


4. Write an equation of the ellipse with foci at (23, 0) 

and (23, 26) and co-vertices at (21, 23) and 

(25, 23). 

(x 1 3)2 

} 

4 

(y 1 3)2 

1 } 5 1 

13 


Your Notes 

Homework 

CLASSIFYING CONICS USING THEIR EQUATIONS 

Any conic can be described by a general second-degree 

equation in x and y: 

Ax2 1 Bxy 1 Cy2 1 Dx 1 Ey 1 F 5 0. 

The expression B2 2 4AC is the discriminant of the 

conic equation and can be used to identify it. 

Discriminant Type of Conic 

B2 2 4AC 0, B 5 0, and A 5 C Circle 

B2 2 4AC 0 and either B Þ 0 or A Þ C Ellipse 

B2 2 4AC 0 Parabola 

B2 2 4AC 0 Hyperbola 

If B 

vertical. 

0, each axis of the conic is horizontal or 

Example 5 Classify a conic 

Classify the conic given by 

2x 2 1 2y 2 2 5x 1 3y 2 1 5 0. 

Solution 

Note that A 5 , B 5 , and C 5 , so the value of 

the discriminant is: B2 2 4AC 5 5 . 

Because B2 2 4AC 0 and A C, the conic is 

a . 

Checkpoint Classify the conic. 

5. 5x 2 2 3y 2 2 10x 2 12y 2 22 5 0 


Your Notes 

Homework 

CLASSIFYING CONICS USING THEIR EQUATIONS 

Any conic can be described by a general second-degree 

equation in x and y: 

Ax2 1 Bxy 1 Cy2 1 Dx 1 Ey 1 F 5 0. 

The expression B2 2 4AC is the discriminant of the 

conic equation and can be used to identify it. 

Discriminant Type of Conic 

B2 2 4AC < 0, B 5 0, and A 5 C Circle 

B2 2 4AC < 0 and either B Þ 0 or A Þ C Ellipse 

B2 2 4AC 5 0 Parabola 

B2 2 4AC > 0 Hyperbola 

If B 5 0, each axis of the conic is horizontal or 

vertical. 

Example 5 Classify a conic 

Classify the conic given by 

2x 2 1 2y 2 2 5x 1 3y 2 1 5 0. 

Solution 

Note that A 5 2 , B 5 0 , and C 5 2 , so the value of 

the discriminant is: B2 2 4AC 5 02 2 4(2)(2) 5 216 . 

Because B2 2 4AC < 0 and A 5 C, the conic is 

a circle . 

Checkpoint Classify the conic. 

5. 5x2 2 3y2 2 10x 2 12y 2 22 5 0 

hyperbola 


Your Notes 

9.7 Solve Quadratic Systems 

Goal p Solve quadratic systems. 

VOCABULARY 

Quadratic system 

Example 1 Solve a linear-quadratic system by graphing 

Solve the system using a graphing calculator. 

y2 2 3x 2 1 5 0 Equation 1 

2x 2 y 5 6 Equation 2 

1. Solve each equation for y. 

Equation 1 Equation 2 

y 2 2 3x 2 1 5 0 2x 2 y 5 6 

y 2 5 2y 5 

y 5 y 5 

2. Graph the equations. Use the 

calculator’s intersect feature to 

find the coordinates of the 

intersection points. The graphs 

of y 5 and 

y 5 intersect at 

Intersection 

X=5 Y=4 

( , ). The graphs of y 5 and 

y 5 intersect at ( , ). 

The solutions are ( , ) and ( , ). Check 

the solutions by substituting the coordinates of the points 

into each of the original equations. 

Checkpoint Use a graphing calculator to solve system. 

1. x 2 1 y 2 5 18 Equation 1 

y 5 2x 1 6 Equation 2 


Your Notes 

9.7 Solve Quadratic Systems 

Goal p Solve quadratic systems. 

VOCABULARY 

Quadratic system A system that includes one or 

more equations of conics 

Example 1 Solve a linear-quadratic system by graphing 

Solve the system using a graphing calculator. 

y2 2 3x 2 1 5 0 Equation 1 

2x 2 y 5 6 Equation 2 

1. Solve each equation for y. 

Equation 1 Equation 2 

y2 2 3x 2 1 5 0 2x 2 y 5 6 

y2 5 3x 1 1 2y 5 22x 1 6 

y 5 6 Ï } 

3x 1 1 y 5 2x 2 6 

2. Graph the equations. Use the 

calculator’s intersect feature to 

find the coordinates of the 

intersection points. The graphs 

Intersection 

X=5 Y=4 

of y 5 2 Ï } 

3x 1 1 and 

y 5 2x 2 6 intersect at 

( 1.75 , 22.5 ). The graphs of y 5 Ï } 

3x 1 1 and 

y 5 2x 2 6 intersect at ( 5 , 4 ). 

The solutions are ( 1.75 , 22.5) and ( 5 , 4 ). Check 

the solutions by substituting the coordinates of the points 

into each of the original equations. 

Checkpoint Use a graphing calculator to solve system. 

1. x 2 1 y 2 5 18 Equation 1 

y 5 2x 1 6 Equation 2 

(3, 3) 


Your Notes 

Example 2 Solve a linear-quadratic system by substitution 

Solve the system using substitution. 

x2 1 y2 5 13 Equation 1 

y 5 x 1 5 Equation 2 

Solution 

Substitute x 1 5 for y in Equation 1 and solve for x. 


x2 1 ( ) 2 5 13 Substitute for y. 

x2 1 5 13 Expand the power. 

5 0 Combine like terms. 

5 0 Divide each side by . 

5 0 Factor. 

x 5 or x 5 Zero product property 

The corresponding y-values are y 5 5 and 

y 5 5 . The solutions are ( , ) and 

( , ). 

Checkpoint Solve the system using substitution. 

2. y 2 1 6x 2 3 5 0 

y 5 2x 2 1 


Your Notes 

Example 2 Solve a linear-quadratic system by substitution 

Solve the system using substitution. 


y 5 x 1 5 Equation 2 

Solution 

Substitute x 1 5 for y in Equation 1 and solve for x. 


x2 1 ( x 1 5 ) 2 5 13 Substitute for y. 

x2 1 x2 1 10x 1 25 5 13 Expand the power. 

2x2 1 10x 1 12 5 0 Combine like terms. 

x2 1 5x 1 6 5 0 Divide each side by 2 . 

(x 1 2)(x 1 3) 5 0 Factor. 

x 5 22 or x 5 23 Zero product property 

The corresponding y-values are y 5 22 1 5 5 3 and 

y 5 23 1 5 5 2 . The solutions are ( 22 , 3 ) and 

( 23 , 2 ). 

Checkpoint Solve the system using substitution. 

2. y 2 1 6x 2 3 5 0 

y 5 2x 2 1 

(0.5, 0), (21, 23) 


Your Notes 

Example 3 Solve a quadratic system by elimination 

Solve the system by elimination. 

5x2 1 y2 1 48x 1 99 5 0 Equation 1 

x2 2 y2 2 9 5 0 Equation 2 

Solution 

Add the equations to eliminate the y2-term and obtain a 

quadratic equation in x. 

5x2 1 y2 1 48x 1 99 5 0 

x 2 2 y 2 2 9 5 0 

5 Add. 

5 Divide each side by . 

5 Factor. 

x 5 or x 5 Zero product property 

When x 5 , y 5 . When x 5 , y 5 . 

The solutions are , , and . 

Checkpoint Solve the system by elimination. 

3. 2x 2 1 2y 2 2 26 5 0 

2x 2 1 y 1 7 5 0 


Your Notes 

Example 3 Solve a quadratic system by elimination 

Solve the system by elimination. 

5x2 1 y2 1 48x 1 99 5 0 Equation 1 

x2 2 y2 2 9 5 0 Equation 2 

Solution 

Add the equations to eliminate the y2-term and obtain a 

quadratic equation in x. 

5x2 1 y2 1 48x 1 99 5 0 

x 2 2 y 2 2 9 5 0 

6x 2 1 48x 1 90 5 0 Add. 

x2 1 8x 1 15 5 0 Divide each side by 6 . 

(x 1 3)(x 1 5) 5 0 Factor. 

x 5 23 or x 5 25 Zero product property 

When x 5 23 , y 5 0 . When x 5 25 , y 5 64 . 

The solutions are (23, 0) , (25, 4) , and (25, 24) . 

Checkpoint Solve the system by elimination. 

3. 2x 2 1 2y 2 2 26 5 0 

2x2 1 y 1 7 5 0 

(3, 2), (23, 2), (2, 23), (22, 23) 


Your Notes 

Homework 

Example 4 Solve a real-life quadratic system 

Treasure Hunt The class is having a treasure hunt that 

begins at the school. 

Clue 1: The treasure is 3 km from the school. 

Clue 2: The treasure is 5 km from the post office. 

(The post office is 4 km west and 6 km north 

of the school.) 

Clue 3: The treasure is 1 km from the town square. (The 

town square is 2 km north of the school.) 

Solution 

Let each unit represent 1 km. If the school is at (0, 0), 

then the post office is at and the town square 

is at . Write equations of circles that represent the 

possible locations of the treasure. The intersection of the 

circles is the location of the treasure. 

Clue 1: 

Clue 2: 

Clue 3: 

Expand the equation from Clue 3, subtract Clue 1 from 

Clue 3, and solve for y. 

2 ( ) 

y 5 

Use y 5 and the equation from Clue 1 to find 

x 5 . The treasure is of the school. 

Checkpoint Find the location of the treasure. 

4. Clue 1: The treasure is 4 blocks from the school. 

Clue 2: The treasure is 5 blocks from the library. (The 

library is 3 blocks north of the school.) 

Clue 3: The treasure is 2 blocks from the science 

center. (The science center is 2 blocks south 

and 4 blocks east of the school.) 


Your Notes 

Homework 

Example 4 Solve a real-life quadratic system 

Treasure Hunt The class is having a treasure hunt that 

begins at the school. 

Clue 1: The treasure is 3 km from the school. 

Clue 2: The treasure is 5 km from the post office. 

(The post office is 4 km west and 6 km north 

of the school.) 

Clue 3: The treasure is 1 km from the town square. (The 

town square is 2 km north of the school.) 

Solution 

Let each unit represent 1 km. If the school is at (0, 0), 

then the post office is at (24, 6) and the town square 

is at (0, 2) . Write equations of circles that represent the 

possible locations of the treasure. The intersection of the 

circles is the location of the treasure. 

Clue 1: x2 1 y2 5 9 

Clue 2: (x 1 4) 2 1 (y 2 6) 2 5 25 

Clue 3: x 2 1 (y 2 2) 2 5 1 

Expand the equation from Clue 3, subtract Clue 1 from 

Clue 3, and solve for y. 

x 2 1 y 2 2 4y 1 4 5 1 

2 ( x 2 1 y 2 5 9 ) 

24y 1 4 5 28 y 5 3 

Use y 5 3 and the equation from Clue 1 to find 

x 5 0 . The treasure is 3 km north of the school. 

Checkpoint Find the location of the treasure. 

4. Clue 1: The treasure is 4 blocks from the school. 

Clue 2: The treasure is 5 blocks from the library. (The 

library is 3 blocks north of the school.) 

Clue 3: The treasure is 2 blocks from the science 

center. (The science center is 2 blocks south 

and 4 blocks east of the school.) 

4 blocks east of the school 


Focus On 

Graphing 

Use after Lesson 9.7 

Your Notes 

Determine Eccentricities 

of Conic Sections 

Goal p Find and apply the eccentricity of a conic section. 

VOCABULARY 

Eccentricity 

ECCENTRICITY OF CONIC SECTIONS 

The eccentricity, e, is defined below. For an ellipse or 

hyperbola, c is the distance from each focus to the center, 

and a is the distance from each vertex to the center. 

Circle: e 5 Parabola: e 5 

Ellipse: e 5 c 

} 

a , and , e , Hyperbola: e 5 c 

a 

} , 

and e . 

Example 1 Find eccentricity 

Find the eccentricity of the conic section represented by 

the equation. 

a. (x 1 2) 2 (x 2 2)2 (y 2 1)2 

5 16y b. } 2 } 

121 25 51 

Solution 

a. For a the eccentricity is e 5 . 

b. This equation represents a with 

a 5 5 , b 5 5 , and 

c 5 Ï } 

a 2 +b 2 5 . The eccentricity is 

e 5 c 

} 

a 5 

≈ . 

Checkpoint Find the eccentricity of the conic section. 

1. (x 1 4) 2 1 (y 1 1) 2 5 4 2. 

(x 1 3)2 

} 

81 

(y 2 4)2 

1 } 5 1 

9 

Copyright © Holt McDougal. All rights reserved. 9.7 Focus On Graphing Algebra 2 Notetaking Guide 257

Focus On 

Graphing 

Use after Lesson 9.7 

Your Notes 

Determine Eccentricities 

of Conic Sections 

Goal p Find and apply the eccentricity of a conic section. 

VOCABULARY 

Eccentricity The distance from a focus to the center 

divided by the distance from a vertex to the center. 

ECCENTRICITY OF CONIC SECTIONS 

The eccentricity, e, is defined below. For an ellipse or 

hyperbola, c is the distance from each focus to the center, 

and a is the distance from each vertex to the center. 

Circle: e 5 0 Parabola: e 5 1 

Ellipse: e 5 c 

} 

a , and 0 , e , 1 Hyperbola: e 5 c 

a 

} , 

and e . 1 

Example 1 Find eccentricity 

Find the eccentricity of the conic section represented by 

the equation. 

a. (x 1 2) 2 (x 2 2)2 (y 2 1)2 

5 16y b. } 2 } 

121 25 51 

Solution 

a. For a parabola, the eccentricity is e 5 1 . 

b. This equation represents a hyperbola with 

a 5 Î} 121 5 11 , b 5 Ï } 

25 5 5 , and 

c 5 Ï } 

a2 +b2 } 

5 Ï 146 . The eccentricity is 

e 5 c Î 

} 

a 5 } 

146 

} ≈ 1.098 . 

11 

Checkpoint Find the eccentricity of the conic section. 

1. (x 1 4) 2 1 (y 1 1) 2 5 4 2. 

(x 1 3)2 

} 

81 

(y 2 4)2 

1 } 5 1 

9 

2 Ï} 2 

e 5 0 e 5 } ≈ 0.943 

3 

Copyright © Holt McDougal. All rights reserved. 9.7 Focus On Graphing Algebra 2 Notetaking Guide 257

Your Notes 

Homework 

Example 2 Use eccentricity to write an equation 

Write an equation of a hyperbola with center (21, 3), 

vertex (3, 3), and e 5 3. 

Solution 

Use the form (x2h)2 (y2k)2 

} 2 } 5 1. The vertex lies 

a2 b2 3 2 5 units from the center, so a 5 . 

Because e 5 c 

} 

a 5 3, you know that c 

} 5 3, or c 5 . 

So, b 2 5 c 2 2a 2 5 2 5 . 

The equation is 

Example 3 Use eccentricity to write a model 

An asteroid orbits the sun in an elliptical path with 

the sun at one focus. The eccentricity of the orbit 

is e 5 0.229 and the length of the major axis is 9.0 

astronomical units (A.U). Find an equation of the 

orbit. (Assume that the major axis is horizontal.) 

Solution 

The equation of the orbit has the form x2 y2 

} 1 } 5 1. You 

a2 b2 know that 2a 5 , or a 5 . 

e 5 c 

} 

a , so 0.229 5 c 

} , or c ≈ 1.03. For an ellipse, 

c 2 = a 2 2 b 2 , so b = Ï } 

So, an equation for the asteroid’s orbit is 

258 9.7 Focus On Graphing Algebra 2 Notetaking Guide Copyright © Holt McDougal. All rights reserved. 

. 

5 Ï }} 

≈ 

x2 y2 x2 y2 

} 1 } 5 1 or } 1 } 5 1, where x and y are 

( ) 2 ( ) 2 20 19 

measured in A.U. 


3. For another asteroid, the eccentricity of the orbit is 

e = 0.459 and the length of the major axis 

(horizontal) is 14.8 A.U. Find an equation of the 

asteroid’s orbit.

Your Notes 

Homework 

Example 2 Use eccentricity to write an equation 

Write an equation of a hyperbola with center (21, 3), 

vertex (3, 3), and e 5 3. 

Solution 

Use the form (x2h)2 (y2k)2 

} 2 } 5 1. The vertex lies 

a2 b2 3 2 (21) 5 4 units from the center, so a 5 4 . 

Because e 5 c 

} 

a 5 3, you know that c 

} 5 3, or c 5 12 . 

4 

So, b2 5 c22a2 5 144 2 16 5 128 . 

The equation is 

(x 1 1)2 

} 

16 

2 (y 2 3)2 

} 

128 

5 1. 

Example 3 Use eccentricity to write a model 

An asteroid orbits the sun in an elliptical path with 

the sun at one focus. The eccentricity of the orbit 

is e 5 0.229 and the length of the major axis is 9.0 

astronomical units (A.U). Find an equation of the 

orbit. (Assume that the major axis is horizontal.) 

Solution 

The equation of the orbit has the form x2 y2 

} 1 } 5 1. You 

a2 b2 know that 2a 5 9.0 , or a 5 4.5 . 

e 5 c 

} 

a , so 0.229 5 c 

} , or c ≈ 1.03. For an ellipse, 

4.5 

c2 = a22 b2 , so b = Ï } 

a2 2c2 5 Ï }} 

(4.5) 2 2 (1.03) 2 ≈ 4.4 

So, an equation for the asteroid’s orbit is 

x2 y2 x2 y2 

} 1 } 5 1 or } 1 } 5 1, where x and y are 

(4.5) 2 (4.4) 2 20 19 

measured in A.U. 


3. For another asteroid, the eccentricity of the orbit is 

e = 0.459 and the length of the major axis 

(horizontal) is 14.8 A.U. Find an equation of the 

asteroid’s orbit. x2 y2 

} 1 } 5 1 

55 43 

258 9.7 Focus On Graphing Algebra 2 Notetaking Guide Copyright © Holt McDougal. All rights reserved.

Words to Review 

Give an example of the vocabulary word. 


Focus, foci 

Circle 

Radius 

1 

Vertices 

y 

1 

x 


Directrix 

Center 

Ellipse 

Copyright © Holt McDougal. All rights reserved. Words to Review Algebra 2 Notetaking Guide 259 

1 

y 

1 

Major axis 

x

Words to Review 

Give an example of the vocabulary word. 


The distance between 

(26, 3) and (2, 5) is 

(5 2 3) 2 1 (2 1 6) 2 

d 5 Ï }} 

68 . 

5 Ï } 

Focus, foci 

The focus for the 

parabola x 2 5 24y is 

(0, 21). 

Circle 

1 

y 

1 

x 2 1 y 2 5 4 

Radius 

The radius of the circle 

x2 1 y2 5 4 is 

r 5 Ï } 

4 5 2. 

Vertices 

The vertices of the 

ellipse x2 

} 

9 

(63, 0). 

x 

y2 

1 } 5 1 are 

4 


The midpoint of the 

line from (26, 3) to 

(2, 5) is 

26 1 2 3 1 5 

1 } , } 

2 2 2 5 (22, 4). 

Directrix 

The directrix for the 

parabola x 2 5 24y is 

y 5 1. 

Center 

The center of the circle 

x 2 1 y 2 5 4 is (0, 0). 

Ellipse 

Copyright © Holt McDougal. All rights reserved. Words to Review Algebra 2 Notetaking Guide 259 

x2 

} 

9 

1 

y 

1 

y2 

1 } 5 1 

4 

Major axis 

The major axis for 

x2 y2 

} 1 } 5 1 is 

9 4 

horizontal (y 5 0). 

x

Co-vertices 

Hyperbola 

2 


1 

y 

y 

2 

1 

Discriminant 

Eccentricity 

x 

x 

Minor axis 

Transverse axis 

General second-degree 

equation 


Review your notes and Chapter 9 by using the 

Chapter Review on pages 666–669 of your textbook. 

260 Words to Review Algebra 2 Notetaking Guide Copyright © Holt McDougal. All rights reserved.

Co-vertices 

The co-vertices for 

x2 

} 

9 

y2 

1 } 5 1 are (0, 62). 

4 

Hyperbola 

y2 

} 

16 

2 

y 

2 

x2 

2 } 5 1 

9 


1 

y 

1 

The circle x 2 1 y 2 5 1 

is a conic section. 

Discriminant 

The discriminant of 

3x 2 1 y 2 1 2x 2 3y 

1 15 5 0 is 

B 2 2 4AC 5 0 2 2 4(3)(1) 

5 212. 

Eccentricity 

x 

x 

The eccentricity of an ellipse with equation 

(x23)2 

} 

49 1 

(y22)2 

} 

9 

5 1 is e 5 c 

} 

a = 

Minor axis 

The minor axis for 

x2 

} 

5 1 is vertical 

9 4 

(x 5 0). 

1 y2 

} 

Transverse axis 

The transverse axis for 

y2 

} 

5 1 is vertical 

16 9 

(x 5 0). 

2 x2 

} 

General second-degree 

equation 

3x 2 1 y 2 1 2x 2 3y 

1 152 0 


x2 1 y2 5 5 

y 5 2x 

2 Ï} 10 

} ≈ 0.904. 

7 

Review your notes and Chapter 9 by using the 

Chapter Review on pages 666–669 of your textbook. 

260 Words to Review Algebra 2 Notetaking Guide Copyright © Holt McDougal. All rights reserved.

x - MathnMind

Create successful ePaper yourself

Delete template?

Save as template?