Frobenius monads and pseudomonoids Introduction - ResearchGate

More documents

Recommendations

Info

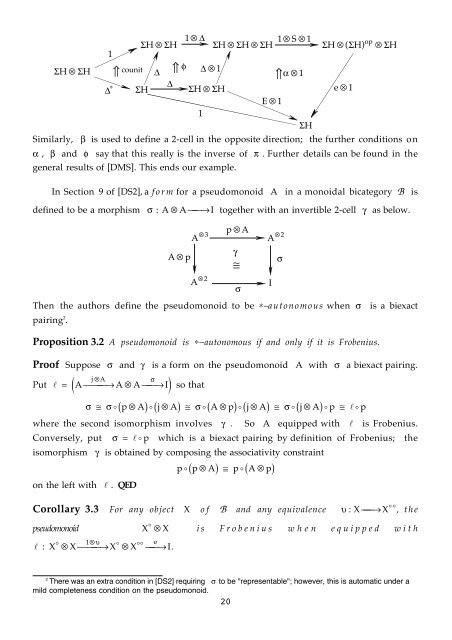
SH ƒ SH 1 D * › counit SH ƒ SH SH D D › f 1 ƒD D ƒ1 SH ƒ SH 1 SH ƒ SH ƒ SH E ƒ 1 1ƒSƒ1 › a ƒ1 SH op SH ƒ( SH) ƒ SH Similarly, b is used to define a 2-cell in the opposite direction; the further conditions on a , b and f say that this really is the inverse of p . Further details can be found in the general results of [DMS]. This ends our example. e ƒ 1 In Section 9 of [DS2], a form for a pseudomonoid A in a monoidal bicategory B is defined to be a morphism s :A ƒA æ æÆItogether with an invertible 2-cell g as below. 1A A ƒ p A ƒ3 p A A ƒ2 Then the authors define the pseudomonoid to be *-autonomous when s is a biexact pairing 2 . ƒ A1 Proposition 3.2 A pseudomonoid is *-autonomous if and only if it is Frobenius. Proof Suppose s and g is a form on the pseudomonoid A with s a biexact pairing. j ƒA s ( ) Put l = A æææÆA ƒA æ æÆI so that g @ s A ƒ2 ( ) ( ƒ ) @ ( ƒ ) ( ƒ ) @ ( ƒ ) @ s @ so p ƒA o j A so A p o j A so j A op lop where the second isomorphism involves g . So A equipped with l is Frobenius. Conversely, put s = lop which is a biexact pairing by definition of Frobenius; the isomorphism g is obtained by composing the associativity constraint on the left with l . QED ( ) @ ( ƒ ) po p A po A p ƒ Corollary 3.3 For any object X of B and any equivalence u :X æÆ æ X oo , the p s e u d o m o n o i d X o ƒ X i s F r o b e n i u s w h e n e q u i p p e d w i t h l o 1ƒ u :X X X o X oo e ƒ æææÆ ƒ æ æÆI. 2 There was an extra condition in [DS2] requiring s to be "representable"; however, this is automatic under a mild completeness condition on the pseudomonoid. 20 I s
Example Star-autonomous monoidal enriched categories A V-category A is monoidal when it is equipped with the structure of pseudomonoid in V - Cat; we write #:A ƒA æ æÆA for the "multiplication" V-functor (to distinguish it from the tensor product ƒ of V ) and J for the unit object. We say that A is *- autonomous when it is equipped with an equivalence of V-categories S V-natural family of isomorphisms ( ) @ ( ). A A# B, J A B, SA op : A æÆ æ A and a This is a straightforward enrichment of the concept due to Barr [Ba3] and considered more generally in [DS2] in connection with quantum groupoids. Here we want to emphasize the Frobenius aspect. In order to obtain an autonomous monoidal bicategory, we need to move from V - Cat to V -Mod. Because of the way we have defined V -Mod, it is better to consider A op rather than A; to say one is monoidal is the same as saying the other is. For simplicity, we also suppose A is Cauchy complete (see Section 5); then any equivalence S A æÆ æ Aop in V -Mod is automatically of the form S * for an equivalence op : A æÆ æ A in V - Cat. As a consequence of Proposition 3.2 we have that: the monoidal V-category A is *-autonomous if and only if A op is Frobenius in V -Mod. If we write ⁄ op op S : A ƒA æ æÆI for the module defined by isomorphism defining *-autonomy is precisely of [DS2] that, for any V-category X, the monoidal V-category §4. Projective equivalences * ⁄ SAB ( , ) A B, SA, we see that the = ( ) J o # @ S. Corollary 3.3 implies a result * ⁄ op X ƒ X is *-autonomous. This section was inspired to a large extent by the discussion of "weak monoidal Morita equivalence" in [Mü1] and [Mü2]. It is well known what it means for a morphism to be an equivalence in any bicategory and that every equivalence can be made an adjoint equivalence. We wish to discuss a more general notion of equivalence. For this we need to clarify a concept of scalar. A scalar for a bicategory D is a modification w : 11 æÆ æ 11 : 1 1 : D D D æ æÆ D D æ ÆD . That is, w assigns to each object A of D a 2-cell w A A A morphisms f: A æÆ æ B, :1 æÆ æ 1 such that, for all f wA = wB f . Scalars form a commutative monoid under composition. By abuse of language, 2-cell 21
Page 1 and 2: Frobenius monads and pseudomonoids
Page 3 and 4: the tensor product), an object I of
Page 5 and 6: qo( koa) qo ( kov) of qo ko( vof) q
Page 7 and 8: only be equivalences rather than is
Page 9 and 10: 2 r :1æÆ æ T satisfying the equa
Page 11 and 12: that m and d are mates as required
Page 13 and 14: Frobenius monad (see pages 151 and
Page 15 and 16: (see [ML] for Yoneda-Day-Kelly inte
Page 17 and 18: n: I æÆ æ A ƒA o and counit In
Page 19: algebra is a quasibialgebra H toget
Page 23 and 24: e¢ o ( u¢ e f¢ ) o ( u¢ hf¢ )
Page 25 and 26: that say t is a monad). Notice that
Page 27: [Law1] F.W. Lawvere, Ordinal sums a

Frobenius monads and pseudomonoids Introduction - ResearchGate

Create successful ePaper yourself

Delete template?

Save as template?