Linear Algebra II (pdf, 500 kB)

14
5.6. Application. One important application of the Jordan Normal Form Theorem
is to the explicit solution of systems of linear first-order differential equations
with constant coefficients. Such a system can be written
d
y(t) = A · y(t) ,
dt
where y is a vector-valued function and A is a matrix. One can then show (Exercise)
that there is a unique solution with y(0) = y0 for any specified initial value y0,
and it is given by
y(t) = exp(tA) · y0
with the matrix exponential
exp(tA) =
∞
n=0
t n
n! An .
If A is in Jordan Normal Form, the exponental can be easily determined. In
general, A can be transformed into Jordan Normal Form, the exponential can be
evaluated for the transformed matrix, then we can transform it back — note that
exp(tP −1 AP ) = P −1 exp(tA)P .
5.7. Remark. What can we do when the characteristic polynomial does not split
into linear factors (which is possible when the field F is not algebraically closed)?
In this case, we have to use a weaker notion than that of diagonalizability. Define
the endomorphism f : V → V to be semi-simple if every f-invariant subspace
U ⊂ V has an f-invariant complementary subspace in V . One can show (Exercise)
that if the characteristic polynomial of f splits into linear factors, then f is semisimple
if and only if it is diagonalizable. The general version of the Jordan Normal
Form Theorem then is as follows.
Let V be a finite-dimensional vector space, f : V → V an endomorphism. Then
f = s + n with endomorphisms s and n of V such that s is semi-simple, n is
nilpotent, and s ◦ n = n ◦ s.
Unfortunately, we do not have the means and time to prove this result here.
However, we can state the result we get over F = R.
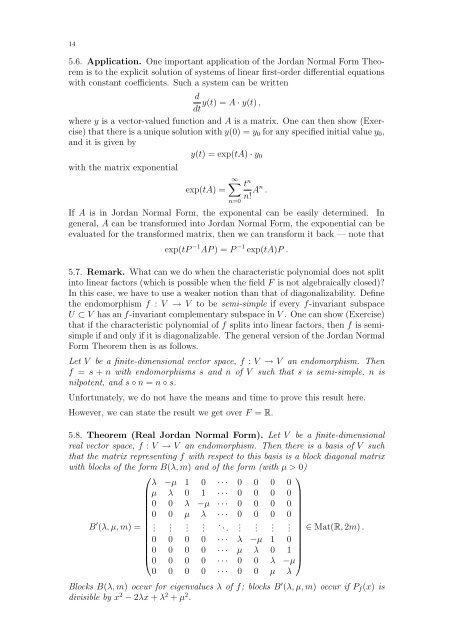
5.8. Theorem (Real Jordan Normal Form). Let V be a finite-dimensional
real vector space, f : V → V an endomorphism. Then there is a basis of V such
that the matrix representing f with respect to this basis is a block diagonal matrix
with blocks of the form B(λ, m) and of the form (with µ > 0)
B ′ ⎛
λ −µ 1 0 · · · 0 0 0
⎞
0
⎜
µ
⎜0
⎜
⎜0
⎜
(λ, µ, m) = ⎜ .
⎜
⎜0
⎜
⎜0
⎝0
λ
0
0
.
0
0
0
0
λ
µ
.
0
0
0
1
−µ
λ
.
0
0
0
· · ·
· · ·
· · ·
...
· · ·
· · ·
· · ·
0
0
0
.
λ
µ
0
0
0
0
.
−µ
λ
0
0
0
0
.
1
0
λ
0 ⎟
0 ⎟
0 ⎟
.
⎟ ∈ Mat(R, 2m) .
0
⎟
1 ⎟
−µ ⎠
0 0 0 0 · · · 0 0 µ λ
Blocks B(λ, m) occur for eigenvalues λ of f; blocks B ′ (λ, µ, m) occur if Pf(x) is
divisible by x 2 − 2λx + λ 2 + µ 2 .