b - NTNU

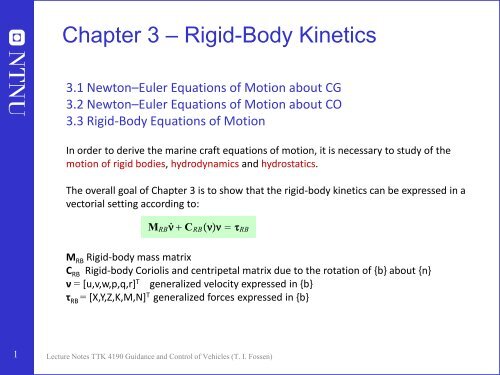
Chapter 3 – Rigid-Body Kinetics
3.1 Newton–Euler Equations of Motion about CG
3.2 Newton–Euler Equations of Motion about CO
3.3 Rigid-Body Equations of Motion
In order to derive the marine craft equations of motion, it is necessary to study of the
motion of rigid bodies, hydrodynamics and hydrostatics.
The overall goal of Chapter 3 is to show that the rigid-body kinetics can be expressed in a
vectorial setting according to:
M RB C RB RB #
M RB Rigid-body mass matrix
C RB Rigid-body Coriolis and centripetal matrix due to the rotation of {b} about {n}
ν = [u,v,w,p,q,r] T generalized velocity expressed in {b}
τ RB = [X,Y,Z,K,M,N] T generalized forces expressed in {b}
1
Lecture Notes TTK 4190 Guidance and Control of Vehicles (T. I. Fossen)

Chapter 3 – Rigid-Body Kinetics
The equations of motion will be represented in two body-fixed reference points:
1) Center of gravity (CG), subscript g
2) Origin o b of {b}, subscript b
These points coincides if the vector
r g 0 CO CG
Time differentiation of a vector
in a moving reference frame {b} satisfies
i d
dt a b d
dt a b/i a
Time differentiation in {b} is denoted as
a : b d
dt a
{b}
r b/i
r g/i
r g
2
{i}={n}
inertial frame

3.1 Newton-Euler Equations of Motion
about CG
Coordinate-free vector: A vector v b/n , velocity of {b} with respect to {n}, is defined by its
magnitude and direction but without reference to a coordinate frame.
i
Coordinate vector: A vector v b/n
decomposed in the inertial reference frame is denoted by v b/n
Newton-Euler Formulation
Newton's Second Law relates mass m, acceleration v g/i and force f g according to:
mv g/i fg
Isaac Newton (1642-1726)
where the subscript g denotes the center of gravity (CG).
Euler's First and Second Axioms
Euler suggested to express Newton's Second Law in terms of conservation
of both linear momentum p g and angular momentum h according to:
g
i
d
dt p g Leonhard Euler (1707-1783)
fg
p g mv g/i #
f g and m g are forces/moments about CG
i
d
is the angular velocity of frame b relative frame i
dt h g m g h g I g b/i # b/i
I g is the inertia dyadic about the body's CG
3
Lecture Notes TTK 4190 Guidance and Control of Vehicles (T. I. Fossen)

3.1.1 Translational Motion about CG
When deriving the equations of motion it will be assumed that:
(1) The vessel is rigid
(2) The NED frame is inertial—that is, {n} ≈ {i}
The first assumption eliminates the consideration of forces acting between individual
elements of mass while the second eliminates forces due to the Earth's motion
relative to a star-fixed inertial reference system such that:
v g/i v g/n
b/i b/n
For guidance and navigation applications in space it is usual to use a star-fixed reference
frame or a reference frame rotating with the Earth. Marine crafts are, on the other
hand, usually related to the NED reference frame. This is a good assumption since
forces on a marine craft due to the Earth's rotation:
e/i 7.2921 10 5 rad/s
are quite small compared to the hydrodynamic forces.
4
Lecture Notes TTK 4190 Guidance and Control of Vehicles (T. I. Fossen)

3.1.1 Translational Motion about CG
r g/n r b/n r g #
{n} is inertial
r g/i r b/i r g #
Time differentiation of
reference frame {b} gives
v g/n v b/n
For a rigid body, CG satisfies
b
d
dt
r g 0 #
r g/n
in a moving
b
d
dt
r g b/n r g #
v g/n v b/n b/n r g #
f g i d
dt
mv g/i
i d
dt
mv g/n
b d mv dt g/n m b/n mv g/n
mv g/n b/n v g/n # b
mv g/n
r g
CO
r b/i
r g/i
{i}={n} inertial frame
{b}
Body-fixed reference frame {b} is fixed in
the point CO and rotating with respect
to the inertial frame {i}
Translational Motion about CG Expressed in {b}
S b
b/n
v b b
g/n f g
#
CG
5
Lecture Notes TTK 4190 Guidance and Control of Vehicles (T. I. Fossen)

3.1.2 Rotational Motion about CG
The derivation starts with the Euler’s 2nd axiom:
m g i d
dt
I g b/i
i d
dt
I g b/n
b d
dt
I g b/n b/n I g b/n
I g b/n I g b/n b/n #
Rotational Motion about CG Expressed in {b}
b
I g b/n
SI g b b
b/n b/n
m g
b
where I g is the inertia matrix
where I x , I y , and I z are the moments of inertia about {b} and I xy =I yx , I xz =I zx and I yz =I zy are the
products of inertia defined as:
I g :
I x I xy I xz
I yx I y I yz , I g I g 0 #
I zx I zy I z
I x V
y 2 z 2 m dV;
I y V
x 2 z 2 m dV;
I z V
x 2 y 2 m dV;
I xy V
xy m dV V
yx m dV I yx
I xz V
xz m dV V
zx m dV I zx
I yz V
yz m dV V
zy m dV I zy
6
Lecture Notes TTK 4190 Guidance and Control of Vehicles (T. I. Fossen)

3.1.3 Equations of Motion about CG
The Newton-Euler equations can be represented in matrix form according to:
CG
M RB
b
v g/n
b
b/n
CG
C RB
b
v g/n
b
b/n
f g
b
m g
b
#
Expanding the matrices give: Isaac Newton (1642-1726)
Leonhard Euler (1707-1783)
mI 33
0 33
b
v g/n
b
b/n
mS b b/n 0 33
0 33 SI g b
b/n
b
v g/n
b
b/n
f g
b
m g
b
#
0 33 I g
CG
M RB
CG
C RB
7
Lecture Notes TTK 4190 Guidance and Control of Vehicles (T. I. Fossen)

3.2 Newton-Euler Equations of Motion
about CO
For marine craft it is desirable to derive the equations of motion
for an arbitrary origin CO to take advantage of the craft's
geometric properties. Since the hydrodynamic forces and
moments often are computed in CO, Newton's laws will be
formulated in CO as well.
Transform the equations of motion from CG to CO
using a coordinate transformation based on:
b
v g/n
b
v b/n
b
v b/n
b
v b/n
b
b/n
r g
b
b
r gb b/n
S b
r gb b/n #
b
v g/n
b
b/n
Hr gb
b
v b/n
b
b/n
#
Transformation matrix:
I 33 S r b
Hr gb :
g
, H r gb
0 33 I 33
I 33
0 33
Sr gb I 33
#
8
Lecture Notes TTK 4190 Guidance and Control of Vehicles (T. I. Fossen)

3.2 Newton-Euler Equations of Motion
about CO
Newton-Euler equations in matrix form (about CG)
CG
M RB
b
v g/n
b
b/n
CG
C RB
b
v g/n
b
b/n
f g
b
m g
b
#
b
v g/n
b
b/n
Hr gb
b
v b/n
b
b/n
#
Expanding the matrices
mI 33
0 33
b
v g/n
b
b/n
mS b b/n 0 33
0 33 SI g b
b/n
b
v g/n
b
b/n
f g
b
m g
b
#
0 33 I g
CG
M RB
CG
C RB
Newton-Euler equations in matrix form (about CO)
H r gb M CG RB Hr gb
b
v b/n
b
b/n
H r gb C CG RB Hr gb
b
v b/n
b
b/n
H r gb
f g
b
m g
b
#
9
Lecture Notes TTK 4190 Guidance and Control of Vehicles (T. I. Fossen)

3.2 Newton-Euler Equations of Motion
about CO
H r gb M CG RB Hr gb
b
v b/n
b
b/n
H r gb C CG RB Hr gb
b
v b/n
b
b/n
H r gb
f g
b
m g
b
#
The mass and Coriolis-centripetal matrices in CO are defined as:
M CO RB : H r gb M CG RB Hr gb
C CO RB : H r gb C CG RB Hr gb
Expanding the matrices
#
#
M CO RB
mI 33
mSr gb
mSr gb
I g mS 2 r gb
#
C CO RB
mS b/n mS b/n Sr gb
mSr gb S b/n SI g mS 2 r gb b/n
#
10
Lecture Notes TTK 4190 Guidance and Control of Vehicles (T. I. Fossen)

3.2.1 Translational Motion about CO
Translational Motion about CO Expressed in {b}
b
mv b/n
S b
b/n
r gb S b b
b/n v b/n
S 2 b
b
b/n r gb f b
#
An alternative representation using vector cross products is:
b
mv b/n
b
b/n
r gb b b
b/n v b/n
b
b/n
b
b
b/n r gb f b
#
11
Copyright © Bjarne Stenberg/NTNU
Lecture Notes TTK 4190 Guidance and Control of Vehicles (T. I. Fossen)

3.2.2 Rotational Motion about CO
Rotational Motion about CO Expressed in {b}
b
I b b/n
S b b
b
b/n I b b/n mSr gb v b/n
mSr gb S b b b
b/n v b/n m b #
An alternative representation using vector cross products is:
b
I b b/n
b
b/n
b
I b b/n
b
mr gb v b/n
b
b/n
v b b
b/n m b #
Theorem 3.1 (Parallel Axes or Huygens-Steiner Theorem)
The inertia matrix I b about an arbitrary origin o b is given by:
I g mSr gb S r gb I g mS 2 r gb
I b #
where I g is the inertia matrix about the body's center of gravity.
Christian Huygens (1629-1695)
Jakob Steiner (1796-1863)
12
Lecture Notes TTK 4190 Guidance and Control of Vehicles (T. I. Fossen)

3.3 Rigid-Body Equations of Motion
f b
b
X,Y,Z - force through o b expressed in b
m b
b
K,M,N - moment about o b expressed in b
b
v b/n u,v,w - linear velocity of o b relative o n expressed in b
b
b/n p,q,r - angular velocity of b relative to n expressed in b
r g
b
x g ,y g ,z g - vector from o b to CG expressed in b
Component form (SNAME 1950)
mu vr wq x g q 2 r 2 y g pq r z g pr q X
mv wp ur y g r 2 p 2 z g qr p x g qp r Y
mw uq vp z g p 2 q 2 x g rp q y g rq p Z
I x p I z I y qr r pqI xz r 2 q 2 I yz pr q I xy
my g w uq vp z g v wp ur K
I y q I x I z rp p qrI xy p 2 r 2 I zx qp rI yz
mz g u vr wq x g w uq vp M
I z r I y I x pq q rpI yz q 2 p 2 I xy rq p I zx
mx g v wp ur y g u vr wq N
13
Lecture Notes TTK 4190 Guidance and Control of Vehicles (T. I. Fossen)

3.3.1 Nonlinear 6-DOF Rigid-Body
Equations of Motion
Matrix-Vector Form (Fossen 1991)
M RB C RB RB
u, v, w, p, q, r
generalized velocity
Property 3.1 (Rigid-Body System Inertia Matrix)
M RB M RB 0, M RB 0 66
M RB
mI 33
mSr g b
mSr g b
I o
I 33 is the identity matrix
m 0 0 0 mzg my g
0 m 0 mzg 0 mxg
I b I b 0 is the inertia
matrix about CO
0 0 m my g mxg 0
0 mzg my g Ix Ixy Ixz
#
Sr b g is the matrix cross
product operator
mzg 0 mxg Iyx Iy Iyz
my g mxg 0 Izx Izy Iz
14
Lecture Notes TTK 4190 Guidance and Control of Vehicles (T. I. Fossen)

3.3.1 Nonlinear 6-DOF Rigid-Body
Equations of Motion
Theorem 3.2 (Coriolis-Centripetal Matrix from System Inertia Matrix)
Let M be a 6×6 system inertia matrix defined as:
M M
M 11 M 12
M 21 M 22
0
where M 21 = M 12T . Then the Coriolis-centripetal matrix can always be parameterized such that
C C
C
0 33 SM 11 1 M 12 2
SM 11 1 M 12 2
SM 21 1 M 22 2
where
1 u, v, w , 2 p, q, r
Proof: Sagatun and Fossen (1991).
15
Lecture Notes TTK 4190 Guidance and Control of Vehicles (T. I. Fossen)

3.3.1 Nonlinear 6-DOF Rigid-Body
Equations of Motion
Property 3.2 (Rigid-Body Coriolis and Centripetal Matrix)
The rigid-body Coriolis and centripetal matrix
that
C RB
is skew-symmetric, that is
C RB C RB , 6
C RB
can always be represented such
The skew-symmetric property is very useful when designing nonlinear motion control
system since the quadratic form ν T C RB (ν)ν ≡ 0.
This is exploited in energy-based designs where Lyapunov functions play a key role. The
same property is also used in nonlinear observer design.
There exists several parameterizations that satisfies Property 3.2. Two of them are
presented on the forthcoming pages:
16
Lecture Notes TTK 4190 Guidance and Control of Vehicles (T. I. Fossen)

3.3.1 Nonlinear 6-DOF Rigid-Body
Equations of Motion
Lagrangian Parameterization:
Application of the Theorem 3.2 with M = M RB yields the following expression:
C RB
0 33 mS 1 mSS 2 r g b
mS 1 mSS 2 r g b
mSS 1 r g b SI o 2
which can be rewritten according to (Fossen and Fjellstad 1995):
C RB
0 33 mS 1 mS 2 Sr gb
mS 1 mSr gb S 2
SI b 2
#
Joseph-Louis Lagrange (1736-1813)
In order to ensure that C RB (ν) = -C RB (ν) T , it is necessary to use S(ν₁)ν₁ = 0
and include S(ν₁) in C RB
{21}
.
17
Lecture Notes TTK 4190 Guidance and Control of Vehicles (T. I. Fossen)

3.3.1 Nonlinear 6-DOF Rigid-Body
Equations of Motion
Linear Velocity Independent Parameterization:
By using the cross-product property S(ν₁)ν₂ = -S(ν₂)ν₁, it is possible to move S(ν₁)ν₂ from
C RB
{12}
to C RB
{11}
. This gives an expression for C RB (ν) that is independent of linear velocity ν₁
(Fossen and Fjellstad 1995):
C RB
mS 2
mSr gb S 2
mS 2 Sr gb
SI b 2
#
Remark 1: This expression is useful when ocean currents enter the equations of motion.
The main reason for this is that C RB (ν) does not depend on linear velocity ν₁. This can be
further exploited when considering a marine craft exposed to irrotational ocean currents.
According to Property 8.1 in Section 8.3:
M RB C RB M RB r C RB r r #
if the relative velocity vector ν r = ν-ν c is defined such that only linear ocean current
velocities are used:
: u c ,v c ,w c ,0,0,0 #
18
Lecture Notes TTK 4190 Guidance and Control of Vehicles (T. I. Fossen)

3.3.2 Linearized 6-DOF Rigid-Body
Equations of Motion
The nonlinear rigid-body equations of motion
M RB C RB RB #
can be linearized about ν₀ = [U,0,0,0,0,0] T for a marine craft moving at forward speed U.
M RB C RB RB #
0 0 0 0 0 0
C RB M RB LU #
The linearized Coriolis and centripetal forces
are recognized as:
L
0 0 0 0 0 1
0 0 0 0 1 0
0 0 0 0 0 0
0 0 0 0 0 0
0 0 0 0 0 0
#
0
mUr
f c
C
RB
mUq
my g Uq mz g Ur
#
mx g Uq
mx g Ur
19
Lecture Notes TTK 4190 Guidance and Control of Vehicles (T. I. Fossen)

3.3.2 Linearized 6 DOF Rigid-Body
Equations of Motion
Simplified 6-DOF Rigid-Body Equations of Motion
(1) Origin CO coincides with the CG
This implies that
r gb 0,0,0 , I b I g
such that
M RB
mI 33
0 33
0 33 I g
#
A further simplification is obtained when the body axes (x b ,y b, z b ) coincide with the principal axes of
inertia. This implies that
I g diagIx cg ,Iy cg ,Iz cg
20
Lecture Notes TTK 4190 Guidance and Control of Vehicles (T. I. Fossen)

3.3.2 Linearized 6-DOF Rigid-Body
Equations of Motion
(2) Translation of the origin CO such that I b becomes diagonal:
The body-fixed frame (x b ,y b, z b ) can be chosen such that:
I b diagI x ,I y ,I z
If I g is a full matrix, the parallel-axes theorem:
gives
I b I g mS 2 r gb I g mr gb r gb r gb r gb I 33 #
I x Ix cg my g2 z g2
I y Iy cg mx g2 z g2
I z Iz cg mx g2 y g2
#
where x g , y g and z g must be chosen such that:
mIyz
cg x g2 Ixy
cg cg
Ixz
mIxz
cg y g2 Ixy
cg cg
Iyz
mIxy
cg z g2 Ixz
cg cg
Iyz
#
21
Lecture Notes TTK 4190 Guidance and Control of Vehicles (T. I. Fossen)