Avoidable structures, II: finite distributive lattices and nicely ...

More documents

Recommendations

Info

24 W. DZIOBIAK, J. JEŽEK, AND R. MCKENZIE M(6N +12N) = 18N ·M . Proof of Proposition 5.8 and Theorem 5.7. The proposition follows immediately by induction, from Lemmas 5.9 and 5.11. Thus, by this proposition, where k = w(P), we get a ((18N) k−1 )-system S k−1 for P where each divided interval (if any) is a critical interval of depth k −1. By Lemma 4.5, each of these divided intervals I[b,c] has width at most 1; i.e., the interval is a chain. Thus the procedure followed in the proof of Lemma 5.10 now produces a (5 · (18N) k−1 )-system S for P that is nice (has no divided intervals). By Lemma 3.4 (3), we have k ≤ 6N +5. This proves Theorem 5.7. • 6. Avoiding {J k : k ≥ N}, III: conclusion Theorem 6.1. For each N ≥ 1 the class A N is well-quasi-orderd by embeddability. Proof. We can assume that N ≥ 3. Assume that this theorem is false. Choose, by Theorem 1.1, a minimal bad sequence 〈L n : n < ω〉 in A N . By removing finitely many terms from the sequence, we can assume that every L n is + o -indecomposable. Let P n = L ∂ n for n < ω. The P n are primitive. By Theorem 5.7, P n is nicely (M)- structured by a system S n , M = 5(18N) 6N+4 . By successively cutting down to subsequences, we can assume that: • There is a basic skeleton S b = (X;b 0 ,...,b m−1 ,c 0 ,...,c m−1 ), such that every S n is an expansion (X,Y 0 ,Y 1 ;b 0 ,...,b m−1 ;c 0 ,...,c m−1 ) of S b . • We have P n = S n (Q n 0,...,Q n m−1) where Q n j are convex subsets of P n with Q n j ∂ < Pn ∂ ∼ = L n . • The characters (¯c n 0,¯c n 1) of S n satisfy (¯c i 0,¯c i 1) ≤ (¯c j 0 ,¯cj 1 ) whenever i < j < ω. Since Q n r ∂ < L n , then the collection {Q n r ∂ : 0 ≤ r < m,0 ≤ n < ω} is well-quasiordered. Thus by successive further cutting down to infinite subsequences, we can assume that • When i < j we have Q i r∂ ≤ Q j r ∂ for all 0 ≤ r < m. But now, by Theorem 5.6, P0 ∂ ≤ P1 ∂ , equivalently, L 0 ≤ L 1 . This is a contradiction, and it concludes our proof of the theorem. • Theorem 6.2. The unavoidable members of 〈D,≤〉 are precisely the lattices isomorphic to a proper sublattice of some J n . Proof. Since {J n : n < ω} is an anti-chain, any unavoidable finite distributive lattice must be isomorphic to a proper sublattice of some J n . But we immediately see that if L is isomorphic to a proper sublattice of J n , then L < J n+m for all m ≥ 0. Thus in this case, {K ∈ D : L ≰ K} is contained in A n−1 and, by Theorem 6.1, is well-quasi-ordered. This means that L is unavoidable. •
AVOIDABLE STRUCTURES, II 25 Theorem 6.3. Let A be any order-ideal in 〈D,≤〉. Then 〈A,≤〉 is well-quasiordered iff A ⊆ A N for some N. If 〈L n : n < ω〉 is any minimal bad sequence in 〈D,≤〉, then all but finitely many L n are among the terms of the sequence 〈J n : n < ω〉. Proof. This is an immediate consequence of Theorem 6.1 and the fact that {J n : n < ω} is an anti-chain. • Corollary 6.4. A universal class K of distributive lattices has uncountably many universal subclasses if and only if it contains infinitely many members of the sequence 〈J n : n < ω〉. Proof. Every subset of an infinite set of pairwise incomparable finite distributive lattices in K generates a different order-ideal of 〈K ∩ D,≤〉. If K contains only finitely many members of 〈J n : n < ω〉, then 〈K ∩ D,≤〉 is well-quasi-ordered; by Theorem 6.3, every order-ideal I in 〈K ∩ D,≤〉 is uniquely determined by the set of minimal elements of 〈(K ∩ D) − I,≤〉, and the set of these minimal elements is finite (up to isomorphism); thus there are only countably many order-ideals in 〈K∩D,≤〉. 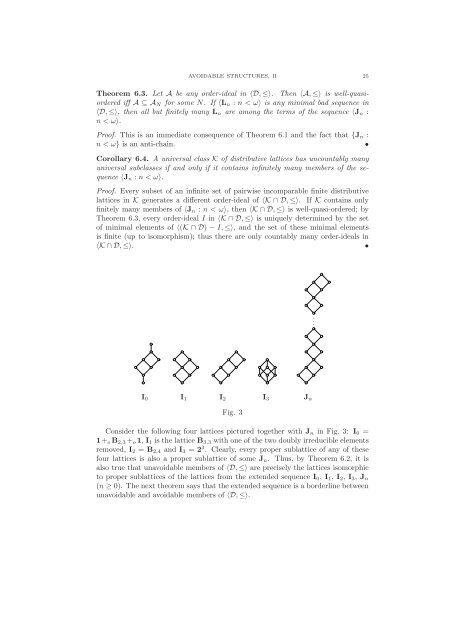• I 0 I 1 I 2 I 3 J n Fig. 3 Consider the following four lattices pictured together with J n in Fig. 3: I 0 = 1+ o B 2,3 + o 1, I 1 is the lattice B 3,3 with one of the two doubly irreducible elements removed, I 2 = B 2,4 and I 3 = 2 3 . Clearly, every proper sublattice of any of these four lattices is also a proper sublattice of some J n . Thus, by Theorem 6.2, it is also true that unavoidable members of 〈D,≤〉 are precisely the lattices isomorphic to proper sublattices of the lattices from the extended sequence I 0 , I 1 , I 2 , I 3 , J n (n ≥ 0). The next theorem says that the extended sequence is a borderline between unavoidable and avoidable members of 〈D,≤〉.
Page 1 and 2: Avoidable structures, II: finite di
Page 3 and 4: AVOIDABLE STRUCTURES, II 3 with n c
Page 5 and 6: AVOIDABLE STRUCTURES, II 5 to D 2 ,
Page 7 and 8: AVOIDABLE STRUCTURES, II 7 Lemma 3.
Page 9 and 10: AVOIDABLE STRUCTURES, II 9 Lemma 3.
Page 11 and 12: AVOIDABLE STRUCTURES, II 11 proved)
Page 13 and 14: AVOIDABLE STRUCTURES, II 13 Conside
Page 15 and 16: AVOIDABLE STRUCTURES, II 15 Proof.
Page 17 and 18: AVOIDABLE STRUCTURES, II 17 I[b 0 ,
Page 19 and 20: AVOIDABLE STRUCTURES, II 19 Definit
Page 21 and 22: AVOIDABLE STRUCTURES, II 21 Assume
Page 23: AVOIDABLE STRUCTURES, II 23 for the
Page 27 and 28: AVOIDABLE STRUCTURES, II 27 7. Comp
Page 29 and 30: AVOIDABLE STRUCTURES, II 29 Claim 4

Avoidable structures, II: finite distributive lattices and nicely ...

You also want an ePaper? Increase the reach of your titles

Delete template?

Save as template?