Chapter 7 Local properties of plane algebraic curves - RISC
Chapter 7 Local properties of plane algebraic curves - RISC
Chapter 7 Local properties of plane algebraic curves - RISC
You also want an ePaper? Increase the reach of your titles
YUMPU automatically turns print PDFs into web optimized ePapers that Google loves.
factor D(z) intersection point multipl. <strong>of</strong> intersection<br />
x 4 (2z − 1) 2 P 1 = (0 : 2 : 1) 4<br />
y z + 1/3 P 2 = (−3 : 0 : 1) 1<br />
3x + y z − 1 P 3 = (1 : −3 : 1) 1<br />
x + 2y z + 1 P 4 = (−2 : 1 : 1) 1<br />
x + y z + 1 P 5 = (−1 : 1 : 1) 1<br />
x − y z − 1 P 6 = (1 : 1 : 1) 1<br />
Table 7.2.<br />
4<br />
y<br />
2<br />
–4 –2 0 2 x 4<br />
–2<br />
–4<br />
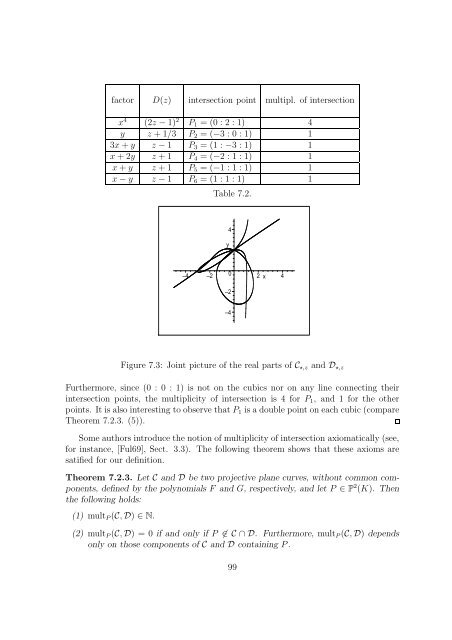
Figure 7.3: Joint picture <strong>of</strong> the real parts <strong>of</strong> C ⋆,z and D ⋆,z<br />
Furthermore, since (0 : 0 : 1) is not on the cubics nor on any line connecting their<br />
intersection points, the multiplicity <strong>of</strong> intersection is 4 for P 1 , and 1 for the other<br />
points. It is also interesting to observe that P 1 is a double point on each cubic (compare<br />
Theorem 7.2.3. (5)).<br />
Some authors introduce the notion <strong>of</strong> multiplicity <strong>of</strong> intersection axiomatically (see,<br />
for instance, [Ful69], Sect. 3.3). The following theorem shows that these axioms are<br />
satified for our definition.<br />
Theorem 7.2.3. Let C and D be two projective <strong>plane</strong> <strong>curves</strong>, without common components,<br />
defined by the polynomials F and G, respectively, and let P ∈ P 2 (K). Then<br />
the following holds:<br />
(1) mult P (C, D) ∈ N.<br />
(2) mult P (C, D) = 0 if and only if P ∉ C ∩ D. Furthermore, mult P (C, D) depends<br />
only on those components <strong>of</strong> C and D containing P.<br />
99