Chapter 7 Local properties of plane algebraic curves - RISC
Chapter 7 Local properties of plane algebraic curves - RISC
Chapter 7 Local properties of plane algebraic curves - RISC
Create successful ePaper yourself
Turn your PDF publications into a flip-book with our unique Google optimized e-Paper software.
Solving them one gets that the linear system is defined by:<br />
H(x, y, z) = a 3 y 3 z 2 − 2a 3 y 4 z + a 3 y 5 + (−a 9 − a 10 ) xy 2 z 2 + a 9 xy 3 z + a 10 xy 4 +<br />
(−a 13 − a 14 − a 15 − a 16 − a 17 − a 18 − a 19 − a 20 ) x 2 yz 2 + a 13 x 2 y 2 z+<br />
a 14 x 2 y 3 + a 15 x 3 z 2 + a 16 x 3 yz + a 17 x 3 y 2 + a 18 x 4 z + a 19 x 4 y + a 20 x 5<br />
4<br />
3<br />
y2<br />
1<br />
–4 –3 –2 –1 0 1 2 3 4<br />
x<br />
–1<br />
–2<br />
–3<br />
–4<br />
4<br />
y<br />
2<br />
–4 –2 0 2 x 4<br />
–2<br />
–4<br />
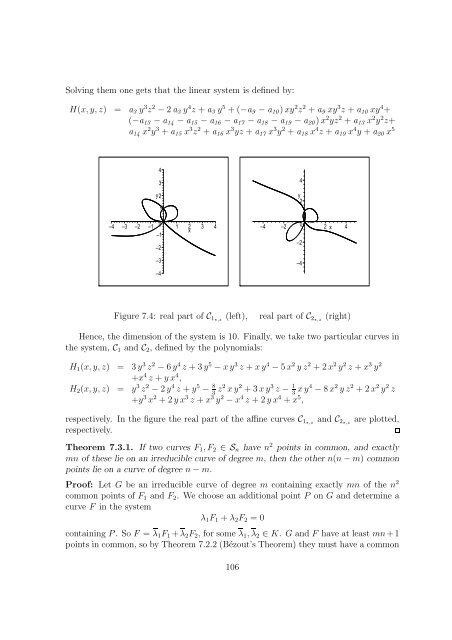
Figure 7.4: real part <strong>of</strong> C 1⋆,z (left),<br />
real part <strong>of</strong> C 2⋆,z (right)<br />
Hence, the dimension <strong>of</strong> the system is 10. Finally, we take two particular <strong>curves</strong> in<br />
the system, C 1 and C 2 , defined by the polynomials:<br />
H 1 (x, y, z) = 3 y 3 z 2 − 6 y 4 z + 3 y 5 − xy 3 z + xy 4 − 5 x 2 y z 2 + 2 x 2 y 2 z + x 3 y 2<br />
+x 4 z + y x 4 ,<br />
H 2 (x, y, z) = y 3 z 2 − 2 y 4 z + y 5 − 8 3 z2 xy 2 + 3 xy 3 z − 1 3 xy4 − 8 x 2 y z 2 + 2 x 2 y 2 z<br />
+y 3 x 2 + 2 y x 3 z + x 3 y 2 − x 4 z + 2 y x 4 + x 5 ,<br />
respectively. In the figure the real part <strong>of</strong> the affine <strong>curves</strong> C 1⋆,z and C 2⋆,z are plotted,<br />
respectively.<br />
Theorem 7.3.1. If two <strong>curves</strong> F 1 , F 2 ∈ S n have n 2 points in common, and exactly<br />
mn <strong>of</strong> these lie on an irreducible curve <strong>of</strong> degree m, then the other n(n − m) common<br />
points lie on a curve <strong>of</strong> degree n − m.<br />
Pro<strong>of</strong>: Let G be an irreducible curve <strong>of</strong> degree m containing exactly mn <strong>of</strong> the n 2<br />
common points <strong>of</strong> F 1 and F 2 . We choose an additional point P on G and determine a<br />
curve F in the system<br />
λ 1 F 1 + λ 2 F 2 = 0<br />
containing P. So F = λ 1 F 1 +λ 2 F 2 , for some λ 1 , λ 2 ∈ K. G and F have at least mn+1<br />
points in common, so by Theorem 7.2.2 (Bézout’s Theorem) they must have a common<br />
106