INFLUENCE OF A NON-STANDARD GEOMETRY ... - Dunarea de Jos

12
THE ANNALS OF UNIVERSITY “DUNĂREA DE JOS “ OF GALAŢI
FASCICLE VIII, 2004, ISSN 1221-4590
TRIBOLOGY
2. CURVED FACE WIDTH
SPUR GEARS WITH MODIFIED
GEOMETRY
The curved face width gear geometry is produced
using both the traditional conjugate surface generation
theory [3] and solid modelling techniques [4]. The
simulation of the non-standard gear generation, using
solid modelling, was based on the following
assumptions:
- the virtual gear blank is a cylindrical primitive that
is rotated about its axis and is provided with
translation motion, tangential to the base circle of the
gear, in order to get the rolling motion required for the
involute tooth form generation;
- the virtual tools, for the gear tooth concave and
convex flank generations, are conical solid primitives
that substitute the rotational motion of the imaginary
rack-cutter flank, with zero pressure angle, about its
inclined axis. The modified gear tooth geometry is
defined by the tool axis inclination β, and by the
radius of the “generating circle”.
Fig. 1. Tri-dimensional representation of curved face
width spur gear teeth
Figure 1 shows the representation of three
generated teeth as solid model. The tooth geometry,
numerically identified in several sections along the
gear face width, is used for further investigations.
3. THE THEORETICAL SLIDING
VELOCITY
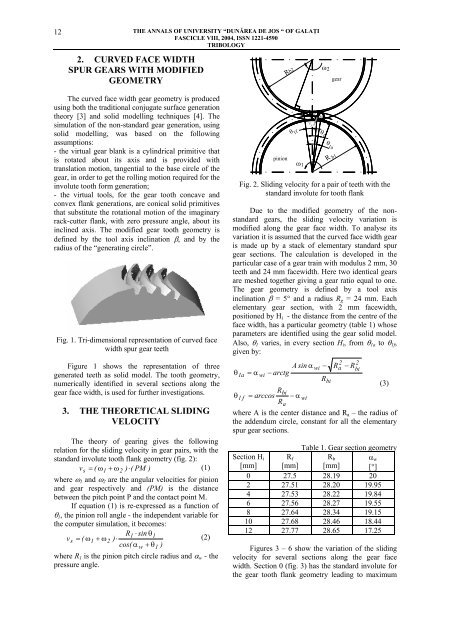
The theory of gearing gives the following
relation for the sliding velocity in gear pairs, with the
standard involute tooth flank geometry (fig. 2):
vs
= ( ω1
+ ω2
) ⋅(
PM )
(1)
where ω 1 and ω 2 are the angular velocities for pinion
and gear respectively and (PM) is the distance
between the pitch point P and the contact point M.
If equation (1) is re-expressed as a function of
θ 1 , the pinion roll angle - the independent variable for
the computer simulation, it becomes:
R1
⋅ sin θ1
vs
= ( ω1
+ ω2
) ⋅
(2)
cos( α w + θ1
)
where R 1 is the pinion pitch circle radius and α w - the
pressure angle.
pinion
Rb2
ω 2
gear
P M
v s
ω 1
θ 1f
θ
1
θ 1a
R b1
Fig. 2. Sliding velocity for a pair of teeth with the
standard involute for tooth flank
Due to the modified geometry of the nonstandard
gears, the sliding velocity variation is
modified along the gear face width. To analyse its
variation it is assumed that the curved face width gear
is made up by a stack of elementary standard spur
gear sections. The calculation is developed in the
particular case of a gear train with modulus 2 mm, 30
teeth and 24 mm facewidth. Here two identical gears
are meshed together giving a gear ratio equal to one.
The gear geometry is defined by a tool axis
inclination β = 5° and a radius R g = 24 mm. Each
elementary gear section, with 2 mm facewidth,
positioned by H i - the distance from the centre of the
face width, has a particular geometry (table 1) whose
parameters are identified using the gear solid model.
Also, θ 1 varies, in every section H i , from θ 1a to θ 1f ,
given by:
2 2
A sin α wi − Ra
− Rbi
θ1a
= α wi − arctg
Rbi
Rbi
θ1 f = arccos − α wi
Ra
(3)
where A is the center distance and R a – the radius of
the addendum circle, constant for all the elementary
spur gear sections.
Section H i
[mm]
R f
Table 1. Gear section geometry
R b α w
[mm] [°]
[mm]
0 27.5 28.19 20
2 27.51 28.20 19.95
4 27.53 28.22 19.84
6 27.56 28.27 19.55
8 27.64 28.34 19.15
10 27.68 28.46 18.44
12 27.77 28.65 17.25
Figures 3 – 6 show the variation of the sliding
velocity for several sections along the gear face
width. Section 0 (fig. 3) has the standard involute for
the gear tooth flank geometry leading to maximum