Universal role of correlation entropy in critical phenomena
Universal role of correlation entropy in critical phenomena
Universal role of correlation entropy in critical phenomena
Create successful ePaper yourself
Turn your PDF publications into a flip-book with our unique Google optimized e-Paper software.
J. Phys. A: Math. Theor. 41 (2008) 025002 S-J Gu et al<br />
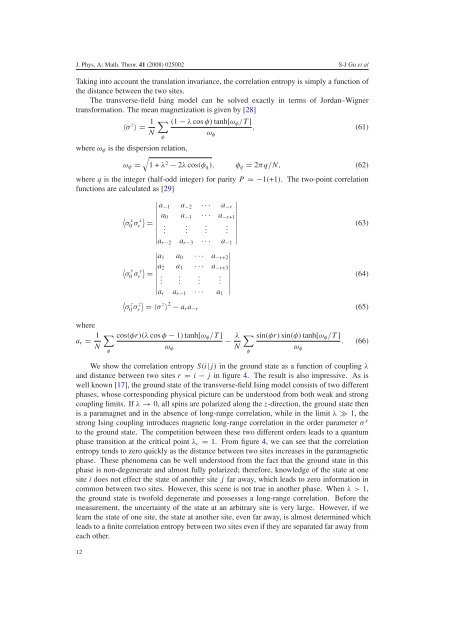
Tak<strong>in</strong>g <strong>in</strong>to account the translation <strong>in</strong>variance, the <strong>correlation</strong> <strong>entropy</strong> is simply a function <strong>of</strong><br />
the distance between the two sites.<br />
The transverse-field Is<strong>in</strong>g model can be solved exactly <strong>in</strong> terms <strong>of</strong> Jordan–Wigner<br />
transformation. The mean magnetization is given by [28]<br />
〈σ z 〉= 1 ∑ (1 − λ cos φ)tanh[ω φ /T]<br />
, (61)<br />
N<br />
ω φ<br />
φ<br />
where ω φ is the dispersion relation,<br />
√<br />
ω φ = 1+λ 2 − 2λ cos(φ q ), φ q = 2πq/N, (62)<br />
where q is the <strong>in</strong>teger (half-odd <strong>in</strong>teger) for parity P =−1(+1). The two-po<strong>in</strong>t <strong>correlation</strong><br />
functions are calculated as [29]<br />
where<br />
a r = 1 ∑<br />
N<br />
φ<br />
∣ ∣ ∣∣∣∣∣∣∣∣ a −1 a −2 ··· a −r ∣∣∣∣∣∣∣∣<br />
〈<br />
σ<br />
x<br />
0 σr<br />
x 〉 a 0 a −1 ··· a −r+1<br />
= (63)<br />
. . . .<br />
a r−2 a r−3 ··· a −1<br />
〈<br />
σ<br />
y<br />
0 σ y r<br />
〈<br />
σ<br />
z<br />
0 σ z r<br />
∣ ∣ ∣∣∣∣∣∣∣∣ a 1 a 0 ··· a −r+2∣∣∣∣∣∣∣∣<br />
〉 a 2 a 1 ··· a −r+3<br />
= .<br />
.<br />
.<br />
.<br />
a r a r−1 ··· a 1<br />
(64)<br />
〉<br />
=〈σ z 〉 2 − a r a −r (65)<br />
cos(φr)(λ cos φ − 1) tanh[ω φ /T]<br />
ω φ<br />
− λ ∑<br />
N<br />
φ<br />
s<strong>in</strong>(φr) s<strong>in</strong>(φ) tanh[ω φ /T]<br />
ω φ<br />
. (66)<br />
We show the <strong>correlation</strong> <strong>entropy</strong> S(i|j) <strong>in</strong> the ground state as a function <strong>of</strong> coupl<strong>in</strong>g λ<br />
and distance between two sites r = i − j <strong>in</strong> figure 4. The result is also impressive. As is<br />
well known [17], the ground state <strong>of</strong> the transverse-field Is<strong>in</strong>g model consists <strong>of</strong> two different<br />
phases, whose correspond<strong>in</strong>g physical picture can be understood from both weak and strong<br />
coupl<strong>in</strong>g limits. If λ → 0, all sp<strong>in</strong>s are polarized along the z-direction, the ground state then<br />
is a paramagnet and <strong>in</strong> the absence <strong>of</strong> long-range <strong>correlation</strong>, while <strong>in</strong> the limit λ ≫ 1, the<br />
strong Is<strong>in</strong>g coupl<strong>in</strong>g <strong>in</strong>troduces magnetic long-range <strong>correlation</strong> <strong>in</strong> the order parameter σ x<br />
to the ground state. The competition between these two different orders leads to a quantum<br />
phase transition at the <strong>critical</strong> po<strong>in</strong>t λ c = 1. From figure 4, we can see that the <strong>correlation</strong><br />
<strong>entropy</strong> tends to zero quickly as the distance between two sites <strong>in</strong>creases <strong>in</strong> the paramagnetic<br />
phase. These <strong>phenomena</strong> can be well understood from the fact that the ground state <strong>in</strong> this<br />
phase is non-degenerate and almost fully polarized; therefore, knowledge <strong>of</strong> the state at one<br />
site i does not effect the state <strong>of</strong> another site j far away, which leads to zero <strong>in</strong>formation <strong>in</strong><br />
common between two sites. However, this scene is not true <strong>in</strong> another phase. When λ>1,<br />
the ground state is tw<strong>of</strong>old degenerate and possesses a long-range <strong>correlation</strong>. Before the<br />
measurement, the uncerta<strong>in</strong>ty <strong>of</strong> the state at an arbitrary site is very large. However, if we<br />
learn the state <strong>of</strong> one site, the state at another site, even far away, is almost determ<strong>in</strong>ed which<br />
leads to a f<strong>in</strong>ite <strong>correlation</strong> <strong>entropy</strong> between two sites even if they are separated far away from<br />
each other.<br />
12