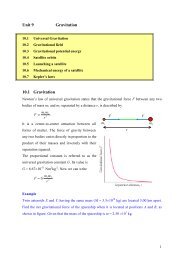
Chapter 2 Stellar Structure Equations 1 Mass conservation equation

With our LTE assumption, matter in the star is in thermal equilibrium.
Thus, the gas inside a star will not expand or contract; the work done by the
gas is zero. Let L(r) denotes the luminosity at the spherical shell with radius
r centered at the core of the star. (That is, L(r) is the energy per unit time
moves out of the spherical shell of radius r.) Energy conservation demands
that
dL
dr = 4πr2 ρ(r)ϵ(r) , (51)
where ϵ is the rate of energy production per unit mass of material at the
radius r from the stellar center. Note that ϵ is an implicit function of density
ρ, temperature T and chemical composition. (Note: a few authors use the
notation q instead of ϵ.)
Using Eq. (2), the above energy production equation can be written as
dL
dm
= ϵ(r) . (52)
Note that L(0) = 0 and L(R) = L where L is the luminosity of the star.
Besides, the region with ϵ(r) > 0 is the region where nuclear fusion takes
place.
6 Some important timescales
• The free-fall / dynamical timescale: For a star with mass M and
radius R, the escape velocity or free fall velocity is of order of √ GM/R.
The free-fall timescale is, therefore, of the order of τ ff ≈ R/ √ GM/R =
√
R3 /GM ≈ 1/ √ G¯ρ where ¯ρ denotes the mean density of the star.
For our Sun, the free-fall timescale is of order of an hour. In short, any
force that is unbalanced inside a star occurs at the free-fall timescale.
Thus, if we are interested in the processes of a stable star over a time
span which is much longer than the free-fall timescale, then we are safe
to assume that the star is in mechanical equilibrium.
• The Kelvin-Helmholtz timescale: This is the timescale τ KH for a
star to radiate its thermal energy away at constant luminosity. By
virial theorem,i.e. KE=PE/2 or KE (the thermal energy) ∼ GM 2 /R,
therefore τ KH ≈ GM 2 /RL. For our Sun, τ KH is about 3 × 10 7 yr. For a
time much longer than the Kelvin-Helmholtz timescale, we may safely
assume that the star is in thermal equilibrium.
12