Chapter 2 Stellar Structure Equations 1 Mass conservation equation

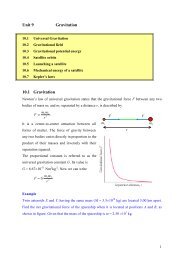
Note that the R.H.S. above is Ω/3 where Ω is the gravitational potential
energy of the entire star. Using integration by parts, the L.H.S. above equals
− ∫ V (R)
P dV = − ∫ M
P/ρ dm. Hence, we arrive at
0 0
Ω = −3
∫ M
0
P
ρ
dm . (8)
This equation is one form of the virial theorem (sometimes known as the
global form of the virial theorem).
We can use the non-relativistic classical ideal gas to obtain the usual form
of virial theorem. The kinetic energy density of an ideal gas ε is simply given
by ε = N 3
kT and the pressure of an ideal gas is P= N kT= 2 ε. Substituting
V 2 V 3
this relation into above equation we get
∫ M
∫
P
V (R) ∫ V (R)
3
0 ρ dm = 3 P dV = 2 εdV = 2U = −Ω (9)
0
Thus, virial theorem tells us that 2U + Ω = 0 where U is the thermal energy
of a star making up of non-relativistic classical ideal gas. Consequently, the
total energy of the star is U + Ω < 0. In other words, the star is in a bound
state.
One consequence of the virial theorem is that upon gravitational contraction,
the star becomes hotter, more tightly bound and have to radiate some
energy to space.
In contrast, if the star is made up of extremely relativistic particles, the
pressure equals one-third the energy density. Hence, in this case, virial theorem
implies that U + Ω = 0. In other words, a star making up of extremely
relativistic particles can be in LTE only when its total energy is 0. So, such
a star is not bounded.
Another consequence of the virial theorem concerns the conversation law.
Consider a star making up of non-relativistic classical ideal gas. Suppose
further that the timescale concerned is small enough that the total energy of
the star is roughly a constant. Since 2U + Ω = 0, the total energy of the star
can be expressed as a function of U (or Ω) alone. Thus, the thermal energy
and the gravitational potential energy of the star are also conserved. In turns
out that this consequence of the virial theorem is useful to understand several
properties of late stage stellar evolution.
3
0