Deformation Techniques for Efficient Polynomial Equation ... - RISC

DEFORMATION TECHNIQUES
85
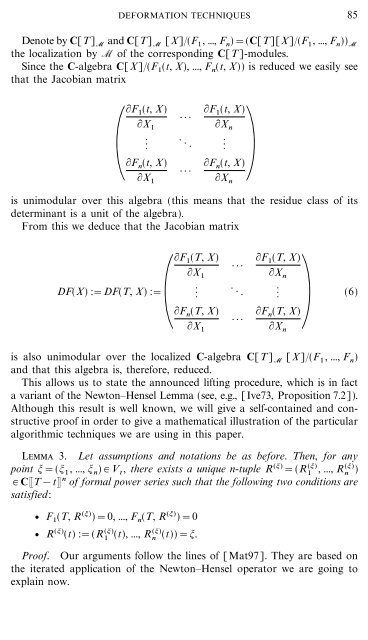
Denote by C[T] M and C[T] M [X](F 1 , ..., F n )=(C[T][X](F 1 , ..., F n )) M
the localization by M of the corresponding C[T]-modules.
Since the C-algebra C[X](F 1 (t, X), ..., F n (t, X)) is reduced we easily see
that the Jacobian matrix
F 1 (t, X) F
}}}
1 (t, X)
\
X 1 X n
.
b . . b
+
F n (t, X) F
}}}
n (t, X)
X 1 X n
is unimodular over this algebra (this means that the residue class of its
determinant is a unit of the algebra).
From this we deduce that the Jacobian matrix
F 1 (T, X) F 1 (T, X)
}}}
X 1 X n
.
DF(X) :=DF(T, b
X):=\
. . b
+
(6)
F n (T, X) F n (T, X)
}}}
X 1 X n
is also unimodular over the localized C-algebra C[T] M [X](F 1 , ..., F n )
and that this algebra is, therefore, reduced.
This allows us to state the announced lifting procedure, which is in fact
a variant of the NewtonHensel Lemma (see, e.g., [Ive73, Proposition 7.2]).
Although this result is well known, we will give a self-contained and constructive
proof in order to give a mathematical illustration of the particular
algorithmic techniques we are using in this paper.
Lemma 3. Let assumptions and notations be as before. Then, for any
point !=(! 1 , ..., ! n )#V t , there exists a unique n-tuple R (!) =(R (!)
1
, ..., R(!) ) n
# CT&t n of formal power series such that the following two conditions are
satisfied:
v F 1 (T, R (!) )=0, ..., F n (T, R (!) )=0
v R (!) (t) :=(R (!)
1
(t), ..., R(!) (t))=!.
n
Proof. Our arguments follow the lines of [Mat97]. They are based on
the iterated application of the NewtonHensel operator we are going to
explain now.