Multifolded torus chaotic attractors: Design and implementation

013118-8 Yu, Lu, and Chen Chaos 17, 013118 2007
For N2, we can transform the multifolded torus system
1 with 3 into two basic linear systems 9 and 11 by
using a simple linear transformation. In the following, we
only select N=5 as an example to explain how to construct
the linear transformation. When N=5, construct the following
linear transformation:
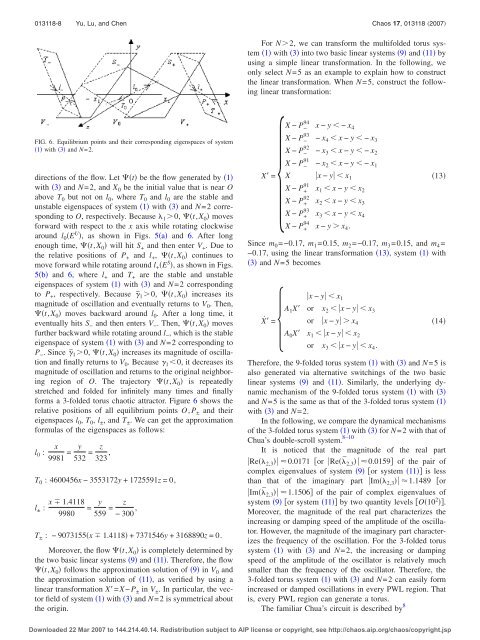
FIG. 6. Equilibrium points and their corresponding eigenspaces of system
1 with 3 and N=2.
directions of the flow. Let t be the flow generated by 1
with 3 and N=2, and X 0 be the initial value that is near O
above T 0 but not on l 0 , where T 0 and l 0 are the stable and
unstable eigenspaces of system 1 with 3 and N=2 corresponding
to O, respectively. Because 1 0, t,X 0 moves
forward with respect to the x axis while rotating clockwise
around l 0 E U , as shown in Figs. 5a and 6. After long
enough time, t,X 0 will hit S + and then enter V + . Due to
the relative positions of P + and l + , t,X 0 continues to
move forward while rotating around l + E S , as shown in Figs.
5b and 6, where l + and T + are the stable and unstable
eigenspaces of system 1 with 3 and N=2 corresponding
to P + , respectively. Because ¯ 10, t,X 0 increases its
magnitude of oscillation and eventually returns to V 0 . Then,
t,X 0 moves backward around l 0 . After a long time, it
eventually hits S − and then enters V − . Then, t,X 0 moves
further backward while rotating around l − , which is the stable
eigenspace of system 1 with 3 and N=2 corresponding to
P − . Since ¯ 10, t,X 0 increases its magnitude of oscillation
and finally returns to V 0 . Because 1 0, it decreases its
magnitude of oscillation and returns to the original neighboring
region of O. The trajectory t,X 0 is repeatedly
stretched and folded for infinitely many times and finally
forms a 3-folded torus chaotic attractor. Figure 6 shows the
relative positions of all equilibrium points O, P ± and their
eigenspaces l 0 , T 0 , l ± , and T ± . We can get the approximation
formulas of the eigenspaces as follows:
l 0 :
x
9981 = y
532 = z
323 ,
T 0 : 4600456x − 3553172y + 1725591z =0,
l ± : x 1.4118
9980
= y
559 = z
− 300 ,
T ± : − 9073155x 1.4118 + 7371546y + 3168890z =0.
Moreover, the flow t,X 0 is completely determined by
the two basic linear systems 9 and 11. Therefore, the flow
t,X 0 follows the approximation solution of 9 in V 0 and
the approximation solution of 11, as verified by using a
linear transformation X=X− P ± in V ± . In particular, the vector
field of system 1 with 3 and N=2 is symmetrical about
the origin.
94
− P − x − y − x 4
93
X − P − − x 4 x − y − x 3
92
X − P − − x 3 x − y − x 2
91
X − P − − x 2 x − y − x 1
X =X X x − y x 1
91
X − P + x 1 x − y x 2
92
X − P + x 2 x − y x 3
93
X − P + x 3 x − y x 4
94
X − P + x − y x 4 .
13
Since m 0 =−0.17, m 1 =0.15, m 2 =−0.17, m 3 =0.15, and m 4 =
−0.17, using the linear transformation 13, system 1 with
3 and N=5 becomes
=
x − y x 1
A 1 X or x 2 x − y x 3
Ẋ or x − y x 4
A 0 X x 1 x − y x 2
or x 3 x − y x 4 .
14
Therefore, the 9-folded torus system 1 with 3 and N=5 is
also generated via alternative switchings of the two basic
linear systems 9 and 11. Similarly, the underlying dynamic
mechanism of the 9-folded torus system 1 with 3
and N=5 is the same as that of the 3-folded torus system 1
with 3 and N=2.
In the following, we compare the dynamical mechanisms
of the 3-folded torus system 1 with 3 for N=2 with that of
Chua’s double-scroll system. 8–10
It is noticed that the magnitude of the real part
Re 2,3 0.0171 or Re¯ 2,30.0159 of the pair of
complex eigenvalues of system 9 or system 11 is less
than that of the imaginary part Im 2,3 1.1489 or
Im¯ 2,31.1506 of the pair of complex eigenvalues of
system 9 or system 11 by two quantity levels O10 2 .
Moreover, the magnitude of the real part characterizes the
increasing or damping speed of the amplitude of the oscillator.
However, the magnitude of the imaginary part characterizes
the frequency of the oscillation. For the 3-folded torus
system 1 with 3 and N=2, the increasing or damping
speed of the amplitude of the oscillator is relatively much
smaller than the frequency of the oscillator. Therefore, the
3-folded torus system 1 with 3 and N=2 can easily form
increased or damped oscillations in every PWL region. That
is, every PWL region can generate a torus.
The familiar Chua’s circuit is described by 8
Downloaded 22 Mar 2007 to 144.214.40.14. Redistribution subject to AIP license or copyright, see http://chaos.aip.org/chaos/copyright.jsp