Bishop's qc-folding and wandering domains in Eremenko ... - ICMS

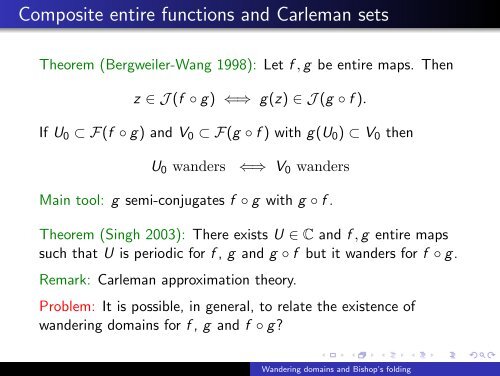
Composite entire functions and Carleman sets
Theorem (Bergweiler-Wang 1998): Let f , g be entire maps. Then
z ∈ J (f ◦ g) ⇐⇒ g(z) ∈ J (g ◦ f ).
If U 0 ⊂ F(f ◦ g) and V 0 ⊂ F(g ◦ f ) with g(U 0 ) ⊂ V 0 then
U 0 wanders
⇐⇒ V 0 wanders
Main tool: g semi-conjugates f ◦ g with g ◦ f .
Theorem (Singh 2003): There exists U ∈ C and f , g entire maps
such that U is periodic for f , g and g ◦ f but it wanders for f ◦ g.
Remark: Carleman approximation theory.
Problem: It is possible, in general, to relate the existence of
wandering domains for f , g and f ◦ g
Wandering domains and Bishop’s folding