MATHEMATICS REVISION OF FORMULAE AND RESULTS
MATHEMATICS REVISION OF FORMULAE AND RESULTS
MATHEMATICS REVISION OF FORMULAE AND RESULTS
You also want an ePaper? Increase the reach of your titles
YUMPU automatically turns print PDFs into web optimized ePapers that Google loves.
<strong>MATHEMATICS</strong> <strong>REVISION</strong> <strong>OF</strong> <strong>FORMULAE</strong> <strong>AND</strong> <strong>RESULTS</strong><br />
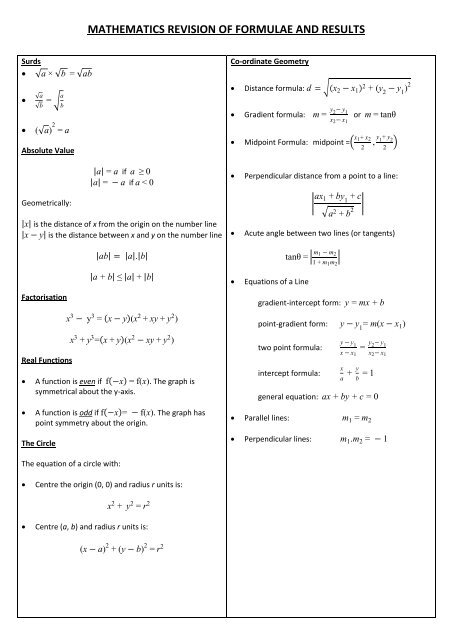
Surds<br />
a × b = ab<br />
<br />
<br />
a<br />
b =<br />
a<br />
b<br />
( a) 2 = a<br />
Absolute Value<br />
Co-ordinate Geometry<br />
Distance formula: d = x 2 − x 1 2 + (y 2<br />
− y 1<br />
) 2<br />
Gradient formula: m = y 2 − y 1<br />
x 2 − x 1<br />
or m = tanθ<br />
Midpoint Formula: midpoint = x 1+ x 2<br />
, y 1 + y 2<br />
2 2<br />
Geometrically:<br />
a = a if a ≥ 0<br />
a = − a if a < 0<br />
x is the distance of x from the origin on the number line<br />
x − y is the distance between x and y on the number line<br />
Factorisation<br />
Real Functions<br />
<br />
ab = a . b<br />
a + b ≤ a + b<br />
x 3 − y 3 = x − y (x 2 + xy + y 2 )<br />
x 3 + y 3 = x + y (x 2 − xy + y 2 )<br />
A function is even if f −x = f(x). The graph is<br />
symmetrical about the y-axis.<br />
<br />
<br />
<br />
Perpendicular distance from a point to a line:<br />
ax 1 + by 1<br />
+ c<br />
a 2 + b 2<br />
Acute angle between two lines (or tangents)<br />
Equations of a Line<br />
tanθ = m 1 − m 2<br />
1 + m 1 m 2<br />
gradient-intercept form: y = mx + b<br />
point-gradient form: y − y 1<br />
= m(x − x 1 )<br />
two point formula:<br />
intercept formula:<br />
y − y 1<br />
x − x 1<br />
= y 2 − y 1<br />
x 2 − x 1<br />
x<br />
a + y b = 1<br />
general equation: ax + by + c = 0<br />
<br />
A function is odd if f −x = − f(x). The graph has<br />
point symmetry about the origin.<br />
Parallel lines: m 1 = m 2<br />
The Circle<br />
Perpendicular lines: m 1 .m 2 = − 1<br />
The equation of a circle with:<br />
<br />
Centre the origin (0, 0) and radius r units is:<br />
x 2 + y 2 = r 2<br />
<br />
Centre (a, b) and radius r units is:<br />
(x − a) 2 + (y − b) 2 = r 2
Trigonometric Results<br />
The Quadratic Polynomial<br />
sinθ =<br />
opposite<br />
hypotenuse<br />
(SOH)<br />
<br />
The general quadratics is: y = ax 2 + bx + c<br />
cosθ =<br />
adjacent<br />
hypotenuse<br />
(CAH)<br />
The quadratic formula is: x = −b ± b2 − 4ac<br />
2a<br />
<br />
The discriminant is: Δ = b 2 − 4ac<br />
<br />
<br />
<br />
tanθ = opposite<br />
adjacent<br />
Complementary ratios:<br />
Pythagorean Identities<br />
(TOA)<br />
sin 90° − θ = cosθ<br />
cos 90° − θ = sinθ<br />
tan 90° − θ = cotθ<br />
sec 90° − θ = cosecθ<br />
cosec(90° − θ) = secθ<br />
sin 2 θ + cos 2 θ = 1<br />
<br />
If Δ ≥ 0 the roots are real<br />
If Δ < 0 the roots are not real<br />
If Δ = 0 the roots are equal<br />
If Δ is a perfect square, the roots are rational<br />
If α and β are the roots of the quadratic equation<br />
ax 2 + bx + c = 0<br />
then: α + β = − b a and αβ = c a<br />
The axis of symmetry is: x = − b<br />
<br />
If a quadratic function is positive for all values of x, it is<br />
positive definite i.e. Δ < 0 and a > 0<br />
2a<br />
1 + cot 2 θ = cosec 2 θ<br />
tanθ = sinθ<br />
cosθ<br />
tan 2 θ + 1 = sec 2 θ<br />
and cotθ = cosθ<br />
sinθ<br />
<br />
<br />
If a quadratic function is negative for all values of x, it<br />
is negative definite i.e. Δ < 0 and a < 0<br />
If a function is sometimes positive and sometimes<br />
negative, it is indefinite i.e. Δ > 0<br />
<br />
<br />
<br />
The Sine Rule<br />
a<br />
= b<br />
= c<br />
sinA sinB sinC<br />
The Cosine Rule<br />
a 2 = b 2 + c 2 − 2bcCosA<br />
CosA = b2 + c 2 − a 2<br />
2bc<br />
The Area of a Triangle<br />
The Parabola<br />
<br />
The parabola x 2 = 4ay has vertex (0,0), focus (0,a),<br />
focal length ‘a’ units and directrix y = − a<br />
The parabola (x − h) 2 = 4a(y − k) has vertex (h, k)<br />
Area = 1 2 abSinC
Differentiation<br />
Geometrical Applications of Differentiation<br />
<br />
First Principles:<br />
<br />
Stationary points:<br />
dy<br />
dx = 0<br />
f ' (x) =<br />
lim<br />
h → ∞<br />
f (x + h) – f (x)<br />
h<br />
or<br />
<br />
Increasing function:<br />
dy<br />
dx > 0<br />
f ' (c) = lim<br />
x → c<br />
f (x) – f (c)<br />
h<br />
<br />
Decreasing function:<br />
dy<br />
dx < 0<br />
<br />
If y = x n then dy<br />
dx = nxn−1<br />
<br />
Concave up:<br />
d 2 y<br />
dx 2 < 0<br />
<br />
Chain Rule: d dx<br />
f (u) = f ' (u)<br />
du<br />
dx<br />
<br />
Concave down:<br />
d 2 y<br />
dx 2 > 0<br />
<br />
<br />
Product Rule: If y = uv then dy<br />
= u dv<br />
+ v du<br />
dx dx dx<br />
Quotient Rule: If y = u v<br />
then<br />
dy<br />
dx = v du<br />
dx + u dv<br />
dx<br />
v 2<br />
<br />
<br />
Minimum turning point:<br />
Maximum turning point:<br />
dy<br />
= 0 dx and d2 y<br />
> 0 dx 2<br />
dy<br />
= 0 dx and d2 y<br />
< 0 dx 2<br />
<br />
Trigonometric Functions:<br />
d<br />
dx<br />
sinx = cosx<br />
<br />
Points of inflexion: d2 y<br />
dx2 = 0 and concavity changes<br />
about the point.<br />
d<br />
dx<br />
d<br />
dx<br />
cosx = − sinx<br />
tanx = sec2 x<br />
<br />
Horizontal points of inflexion: dy<br />
= 0 and d2 y<br />
= 0 and<br />
dx dx 2<br />
concavity changes about the point.<br />
<br />
Exponential Functions:<br />
d<br />
dx<br />
ef (x) = f ' (x)e f (x)<br />
d<br />
dx<br />
ax = a x .lna<br />
<br />
Logarithmic Functions:<br />
d<br />
dx<br />
log e<br />
f (x) =<br />
f ' (x)<br />
f (x)
Approximation Methods<br />
Sequences and Series<br />
<br />
The Trapezoidal Rule:<br />
<br />
Arithmetic Progression<br />
a<br />
b<br />
f x dx = h 2<br />
y 0 + y n + 2 y 1 + y 2 + y 3 + …+ y n−1<br />
d = U 2 − U 1<br />
U n = a + n − 1 d<br />
<br />
Simpson’s Rule:<br />
S n = n [2a + n − 1 d]<br />
2<br />
b<br />
f x dx = h 3<br />
y 0 + y n + 4 y 1 + y 3 + … + 2 y 2 + y 4 + …<br />
S n = n [a + l] where l is the last term<br />
2<br />
a<br />
<br />
Geometric Progression<br />
In both rules, h = b − a<br />
where n is the number of strips.<br />
n<br />
Integration<br />
<br />
If f (x) ≥ 0 for a ≤ x ≤ b, the area bounded by the<br />
curve y = f (x), the x-axis and x = a and x = b is given<br />
by<br />
b<br />
a<br />
f x dx.<br />
r = U 2<br />
U 1<br />
U n = ar n−1<br />
S n = a rn − 1<br />
r − 1<br />
S ∞ =<br />
a<br />
1 − r<br />
= a 1 − rn<br />
1 − r<br />
<br />
<br />
<br />
<br />
The volume obtained by rotating the curve y = f (x)<br />
about the x-axis between x = a and x = b is given by<br />
b<br />
a<br />
π f x 2<br />
If dx<br />
dx = xn then y = xn+1<br />
n + 1<br />
If dx<br />
= ax + b n then y = ax + b n<br />
dx a(n + 1)<br />
Trigonometric Functions:<br />
The Trigonometric Functions<br />
radians = 180⁰<br />
Length of an arc: l = rθ<br />
Area of a sector: A = 1 2 r2 θ<br />
Area of a segment: A = 1 2 r2 (θ − sinθ)<br />
[In these formulae, is measured in radians.]<br />
sin x dx = − cosx + C<br />
<br />
Small angle results:<br />
cos x dx = sinx + C<br />
sec 2 x dx = tanx + C<br />
sinx → 0<br />
cosx → 1<br />
tanx → 0<br />
lim<br />
x → 0<br />
sinx<br />
x<br />
= lim<br />
x → 0<br />
tanx<br />
x<br />
= 1<br />
<br />
Exponential Functions:<br />
e ax dx = eax<br />
a + C and ax dx =<br />
1<br />
ln a .ax<br />
<br />
<br />
For y = sin nx and y = cos nx the period is 2π<br />
For y = sin nx the period is π n<br />
n<br />
<br />
Logarithmic Functions:<br />
f ' (x)<br />
f (x) dx = log e x + C
Logarithmic and Exponential Functions<br />
<br />
The Index Laws:<br />
a x × a y = a x+y<br />
a x ÷ a y = a x−y<br />
a x y = a xy<br />
a −x = 1 a x<br />
x<br />
y<br />
ay = a x<br />
a 0 = 1<br />
<br />
The logarithmic Laws:<br />
If log a<br />
b = c then a c = b<br />
log a<br />
x + log a<br />
y = log a<br />
xy<br />
log a<br />
x − log a<br />
y = log a x y<br />
log a<br />
x n + nlog a<br />
x<br />
log a<br />
a = 1 and log a<br />
1 = 0<br />
<br />
The Change of Base Result:<br />
log a<br />
b = log e b<br />
= log 10 b<br />
log e a log 10 a