LECTURES ON ZARISKI VAN-KAMPEN THEOREM 1. Introduction ...
LECTURES ON ZARISKI VAN-KAMPEN THEOREM 1. Introduction ...
LECTURES ON ZARISKI VAN-KAMPEN THEOREM 1. Introduction ...
You also want an ePaper? Increase the reach of your titles
YUMPU automatically turns print PDFs into web optimized ePapers that Google loves.
<strong>LECTURES</strong> <strong>ON</strong> <strong>ZARISKI</strong> <strong>VAN</strong>-<strong>KAMPEN</strong> <strong>THEOREM</strong> 9<br />
<br />
<br />
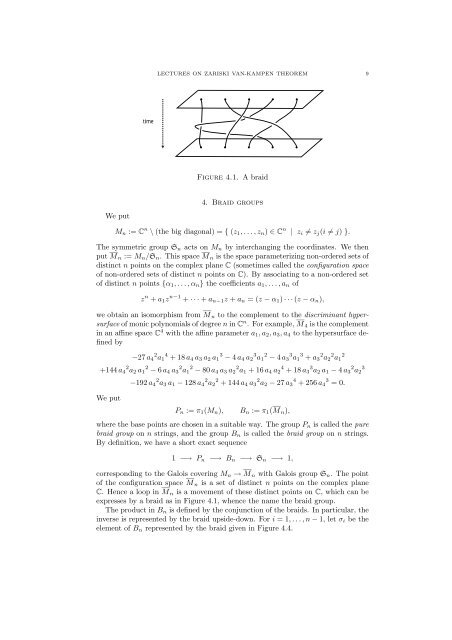
Figure 4.<strong>1.</strong> A braid<br />
We put<br />
4. Braid groups<br />
M n := C n \ (the big diagonal) = { (z 1 ,...,z n ) ∈ C n | z i ≠ z j (i ≠ j) }.<br />
The symmetric group S n acts on M n by interchanging the coordinates. We then<br />
put M n := M n /S n . This space M n is the space parameterizing non-ordered sets of<br />
distinct n points on the complex plane C (sometimes called the configuration space<br />
of non-ordered sets of distinct n points on C). By associating to a non-ordered set<br />
of distinct n points {α 1 ,...,α n } the coefficients a 1 ,...,a n of<br />
z n + a 1 z n−1 + ···+ a n−1 z + a n =(z − α 1 ) ···(z − α n ),<br />
we obtain an isomorphism from M n to the complement to the discriminant hypersurface<br />
of monic polynomials of degree n in C n . For example, M 4 is the complement<br />
in an affine space C 4 with the affine parameter a 1 ,a 2 ,a 3 ,a 4 to the hypersurface defined<br />
by<br />
−27 a 4 2 a 1 4 +18a 4 a 3 a 2 a 1 3 − 4 a 4 a 2 3 a 1 2 − 4 a 3 3 a 1 3 + a 3 2 a 2 2 a 1<br />
2<br />
+144 a 4 2 a 2 a 1 2 − 6 a 4 a 3 2 a 1 2 − 80 a 4 a 3 a 2 2 a 1 +16a 4 a 2 4 +18a 3 3 a 2 a 1 − 4 a 3 2 a 2<br />
3<br />
We put<br />
−192 a 4 2 a 3 a 1 − 128 a 4 2 a 2 2 + 144 a 4 a 3 2 a 2 − 27 a 3 4 + 256 a 4 3 =0.<br />
P n := π 1 (M n ), B n := π 1 (M n ),<br />
where the base points are chosen in a suitable way. The group P n is called the pure<br />
braid group on n strings, and the group B n is called the braid group on n strings.<br />
By definition, we have a short exact sequence<br />
1 −→ P n −→ B n −→ S n −→ 1,<br />
corresponding to the Galois covering M n → M n with Galois group S n . The point<br />
of the configuration space M n is a set of distinct n points on the complex plane<br />
C. Hence a loop in M n is a movement of these distinct points on C, which can be<br />
expresses by a braid as in Figure 4.1, whence the name the braid group.<br />
The product in B n is defined by the conjunction of the braids. In particular, the<br />
inverse is represented by the braid upside-down. For i =1,...,n− 1, let σ i be the<br />
element of B n represented by the braid given in Figure 4.4.