MATH1725 Introduction to Statistics: Worked examples

Joint probabilities p(x,y) are found by summing probabilities for each outcome giving rise to
(X = x,Y = y). Thus p(1,2) = pr{HTT or TTH} = 1/4.
Marginal probabilities are found by forming row or column sum. For example
pr{X = 2} = p(2,1) + p(2,2) + p(2,3) = 3 8 .
(c) If X = 1, then
pr{Y = y|X = 1} = p(1,y)
p X (1) = p(1,y)
3/8 .
Thus
pr{Y = 1|X = 1} = 1/8
2/8
= 1/3, pr{Y = 2|X = 1} = = 2/3, pr{Y = 3|X = 1} = 0.
3/8 3/8
If X = 1, then the outcome is one of HTT, THT, TTH. In one out of these three cases we observe
Y = 1 and in two out of three we observe Y = 2.
Worked Example: Lecture 11
Suppose X and Y are independent continuous random variables which are each uniformly distributed
on the interval (0,1).
(a) Find the probability that 0 < X + Y < z for values z ∈ (0,2).
(b) If Z = X + Y , deduce the form of the probability density function f(z) of Z.
Hints: In (a), think about the area on the x-y plane corresponding to 0 < x + y < z. In (b), first
find the cumulative distribution function F(z) = pr{Z ≤ z}.
Answer: As X and Y are uniformly distributed on the interval [0,1) they have pdf
f X (x) =
{ 1 if 0 < x < 1,
0 otherwise,
f Y (y) =
{ 1 if 0 < y < 1,
0 otherwise.
(a)
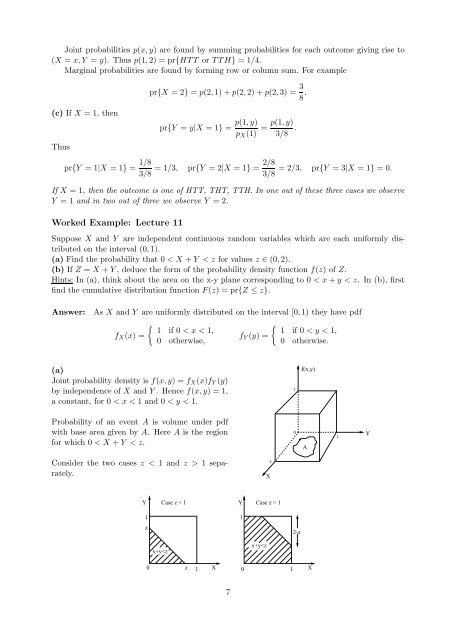
Joint probability density is f(x,y) = f X (x)f Y (y)
by independence of X and Y . Hence f(x,y) = 1,
a constant, for 0 < x < 1 and 0 < y < 1.
1
f(x,y)
Probability of an event A is volume under pdf
with base area given by A. Here A is the region
for which 0 < X + Y < z.
0
A
1
Y
Consider the two cases z < 1 and z > 1 separately.
1
X
Y
1
Case z < 1 Y Case z > 1
1
z
2-z
x+y