Orientation reversal of manifolds - Universität Bonn

2.7 3-manifolds 27
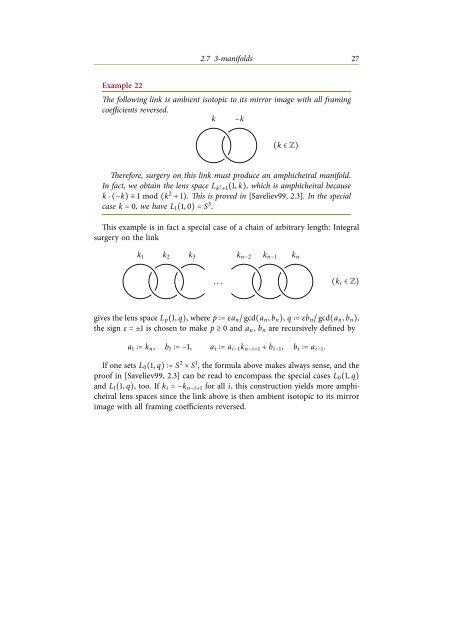
Example 22
The following link is ambient isotopic to its mirror image with all framing
coefficients reversed.
k −k
(k ∈ Z)
Therefore, surgery on this link must produce an amphicheiral manifold.
In fact, we obtain the lens space L k 2 +1(1, k), which is amphicheiral because
k ⋅ (−k) ≡ 1 mod (k 2 + 1). This is proved in [Saveliev99, 2.3]. In the special
case k = 0, we have L 1 (1, 0) = S 3 .
This example is in fact a special case of a chain of arbitrary length: Integral
surgery on the link
k 1 k 2 k 3 k n−2 k n−1 k n
. . .
(k i ∈ Z)
gives the lens space L p (1, q), where p ∶= εa n / gcd(a n , b n ), q ∶= εb n / gcd(a n , b n ),
the sign ε = ±1 is chosen to make p ≥ 0 and a n , b n are recursively defined by
a 1 ∶= k n , b 1 ∶= −1, a i ∶= a i−1 k n−i+1 + b i−1 , b i ∶= a i−1 .
If one sets L 0 (1, q) ∶= S 2 × S 1 , the formula above makes always sense, and the
proof in [Saveliev99, 2.3] can be read to encompass the special cases L 0 (1, q)
and L 1 (1, q), too. If k i = −k n−i+1 for all i, this construction yields more amphicheiral
lens spaces since the link above is then ambient isotopic to its mirror
image with all framing coefficients reversed.