Orientation reversal of manifolds - Universität Bonn
Orientation reversal of manifolds - Universität Bonn
Orientation reversal of manifolds - Universität Bonn
Create successful ePaper yourself
Turn your PDF publications into a flip-book with our unique Google optimized e-Paper software.
34 3 Examples in every dimension ≥ 3<br />
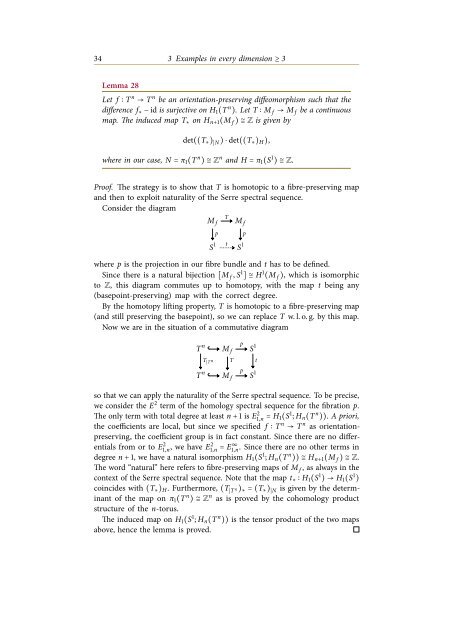
Lemma 28<br />
Let f ∶ T n → T n be an orientation-preserving diffeomorphism such that the<br />
difference f ∗ − id is surjective on H 1 (T n ). Let T ∶ M f → M f be a continuous<br />
map. The induced map T ∗ on H n+1 (M f ) ≅ Z is given by<br />
det((T ∗ ) ∣N ) ⋅ det((T ∗ ) H ),<br />
where in our case, N = π 1 (T n ) ≅ Z n and H = π 1 (S 1 ) ≅ Z.<br />
Pro<strong>of</strong>. The strategy is to show that T is homotopic to a fibre-preserving map<br />
and then to exploit naturality <strong>of</strong> the Serre spectral sequence.<br />
Consider the diagram<br />
M f<br />
p<br />
T<br />
M f<br />
p<br />
S 1 t S 1<br />
where p is the projection in our fibre bundle and t has to be defined.<br />
Since there is a natural bijection [M f , S 1 ] ≅ H 1 (M f ), which is isomorphic<br />
to Z, this diagram commutes up to homotopy, with the map t being any<br />
(basepoint-preserving) map with the correct degree.<br />
By the homotopy lifting property, T is homotopic to a fibre-preserving map<br />
(and still preserving the basepoint), so we can replace T w. l. o. g. by this map.<br />
Now we are in the situation <strong>of</strong> a commutative diagram<br />
T n p<br />
M f<br />
T ∣T n T<br />
T n M f<br />
p<br />
S 1 t<br />
S 1<br />
so that we can apply the naturality <strong>of</strong> the Serre spectral sequence. To be precise,<br />
we consider the E 2 term <strong>of</strong> the homology spectral sequence for the fibration p.<br />
The only term with total degree at least n + 1 is E1,n 2 = H 1(S 1 ; H n (T n )). A priori,<br />
the coefficients are local, but since we specified f ∶ T n → T n as orientationpreserving,<br />
the coefficient group is in fact constant. Since there are no differentials<br />
from or to E1,n 2 , we have E2 1,n = E∞ 1,n . Since there are no other terms in<br />
degree n + 1, we have a natural isomorphism H 1 (S 1 ; H n (T n )) ≅ H n+1 (M f ) ≅ Z.<br />
The word “natural” here refers to fibre-preserving maps <strong>of</strong> M f , as always in the<br />
context <strong>of</strong> the Serre spectral sequence. Note that the map t ∗ ∶ H 1 (S 1 ) → H 1 (S 1 )<br />
coincides with (T ∗ ) H . Furthermore, (T ∣T n) ∗ = (T ∗ ) ∣N is given by the determinant<br />
<strong>of</strong> the map on π 1 (T n ) ≅ Z n as is proved by the cohomology product<br />
structure <strong>of</strong> the n-torus.<br />
The induced map on H 1 (S 1 ; H n (T n )) is the tensor product <strong>of</strong> the two maps<br />
above, hence the lemma is proved.