Orientation reversal of manifolds - Universität Bonn

3.1 Examples in every odd dimension ≥ 3 35
Having chosen a basis for H 1 (T n ) ≅ Z n , every invertible matrix A ∈ SL(n, Z)
can be realised as the induced map on H 1 (T n ) of an orientation-preserving
diffeomorphism f ∶ T n → T n . Hence, we can construct a chiral (n + 1)-manifold
under the following circumstances:
Lemma 29
Suppose there is a matrix A ∈ SL(n, Z) such that
(a) det(A − id) = ±1,
(b) the equation AB = BA has no solution B ∈ GL(n, Z), det B = −1,
(c) the equation A −1 B = BA has no solution B ∈ SL(n, Z).
Then a mapping torus M f with f ∶ T n → T n realising A on H 1 (T n ) ≅ Z n
has no map onto itself with degree −1.
Proof. This is a consequence of Proposition 27 and Lemma 28. For the reader’s
convenience, we list the correspondence between the notations here and in
Proposition 27:
A = f ∗ = ψ(1), A −1 = ψ(−1),
T H (1) = { +1
−1 , T ∣N = B.
For odd n, this method fails because B = −A is a solution for equation (b).
This is the reason why this approach does not yield examples in even dimensions
n + 1. For even n ≥ 2, we construct an example in each dimension, thus
proving Theorem 23.
It is shown that every matrix A ∈ M(n × n; Z), for n even, with characteristic
polynomial
χ A (X) = X n − X + 1
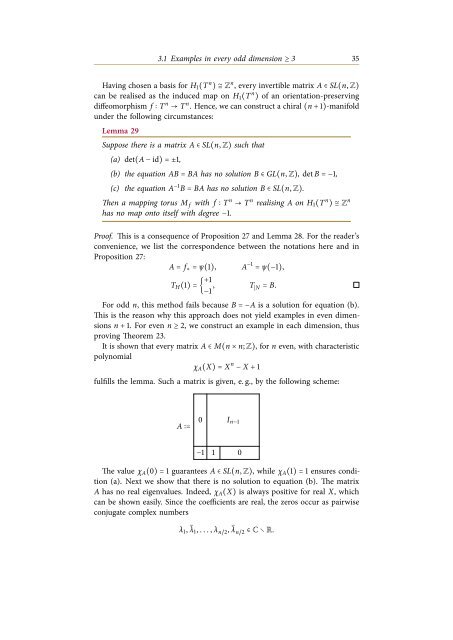
fulfills the lemma. Such a matrix is given, e. g., by the following scheme:
A ∶=
0 I n−1
−1 1 0
The value χ A (0) = 1 guarantees A ∈ SL(n, Z), while χ A (1) = 1 ensures condition
(a). Next we show that there is no solution to equation (b). The matrix
A has no real eigenvalues. Indeed, χ A (X) is always positive for real X, which
can be shown easily. Since the coefficients are real, the zeros occur as pairwise
conjugate complex numbers
λ 1 , ̅λ 1 , . . . , λ n/2 , ̅λ n/2 ∈ C ∖ R.