Orientation reversal of manifolds - Universität Bonn

30 3 Examples in every dimension ≥ 3
For the chirality of the even-dimensional examples, we use the cup product
structure in cohomology in a way which cannot be reduced to the intersection
or linking form as in the simpler examples of Chapter 2.
3.1
Examples in every odd dimension ≥ 3
Theorem 23
In every odd dimension ≥ 3, there is a closed orientable manifold that does
not admit a continuous map to itself with degree −1.
Examples of such manifolds will be provided by mapping tori of n-dimensional
tori T n = S 1 × . . . × S 1 . Although the base space S 1 and the fibre T n
are amphicheiral, the fibration is twisted in a way that makes orientation reversal
impossible. We can exclude orientation-reversing maps by studying
the endomorphisms of the fundamental group. Therefore, we first work out
some properties of the kind of groups which we will encounter as fundamental
groups.
Lemma 24
Abelianisation is a right exact functor.
Although abelianisation as a functor is left adjoint to the inclusion of abelian
groups into all groups, the simple category-theoretic argument “left adjoint
functors are right exact” only applies to abelian categories. Therefore, the
exactness property is checked ad hoc.
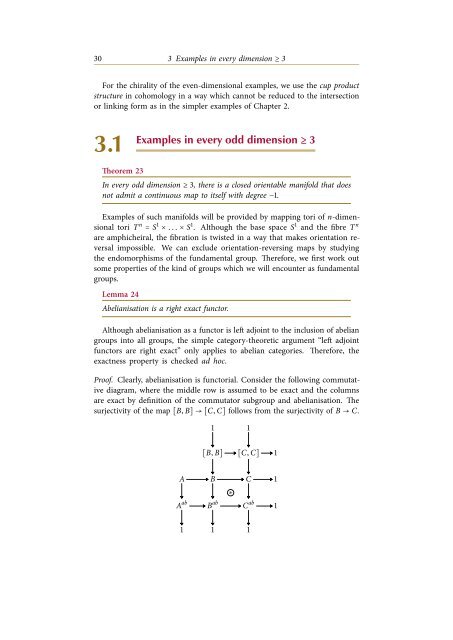
Proof. Clearly, abelianisation is functorial. Consider the following commutative
diagram, where the middle row is assumed to be exact and the columns
are exact by definition of the commutator subgroup and abelianisation. The
surjectivity of the map [B, B] → [C, C] follows from the surjectivity of B → C.
1 1
[B, B] [C, C] 1
A B C 1
∗
A ab B ab C ab 1
1 1 1