University of Paderborn Department of Mathematics Diploma Thesis ...

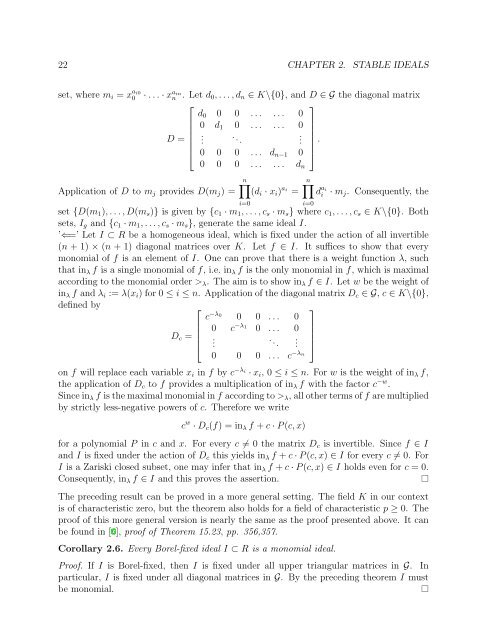
22 CHAPTER 2. STABLE IDEALSset, where m i = x a i00 · . . . · x a inn . Let d 0 , . . . , d n ∈ K\{0}, and D ∈ G the diagonal matrix⎡⎤d 0 0 0 . . . . . . 00 d 1 0 . . . . . . 0D =⎢... . ..⎥⎣ 0 0 0 . . . d n−1 0 ⎦0 0 0 . . . . . . d nApplication of D to m j provides D(m j ) =n∏(d i · x i ) a i=i=0n∏i=0d a ii· m j . Consequently, theset {D(m 1 ), . . . , D(m s )} is given by {c 1 · m 1 , . . . , c s · m s } where c 1 , . . . , c s ∈ K\{0}. Bothsets, I g and {c 1 · m 1 , . . . , c s · m s }, generate the same ideal I.’⇐=’ Let I ⊂ R be a homogeneous ideal, which is fixed under the action of all invertible(n + 1) × (n + 1) diagonal matrices over K. Let f ∈ I. It suffices to show that everymonomial of f is an element of I. One can prove that there is a weight function λ, suchthat in λ f is a single monomial of f, i.e. in λ f is the only monomial in f, which is maximalaccording to the monomial order > λ . The aim is to show in λ f ∈ I. Let w be the weight ofin λ f and λ i := λ(x i ) for 0 ≤ i ≤ n. Application of the diagonal matrix D c ∈ G, c ∈ K\{0},defined by⎡⎤c −λ 00 0 . . . 00 c −λ 10 . . . 0D c = ⎢⎣....⎥ . ⎦0 0 0 . . . c −λnon f will replace each variable x i in f by c −λi · x i , 0 ≤ i ≤ n. For w is the weight of in λ f,the application of D c to f provides a multiplication of in λ f with the factor c −w .Since in λ f is the maximal monomial in f according to > λ , all other terms of f are multipliedby strictly less-negative powers of c. Therefore we writec w · D c (f) = in λ f + c · P (c, x)for a polynomial P in c and x. For every c ≠ 0 the matrix D c is invertible. Since f ∈ Iand I is fixed under the action of D c this yields in λ f + c · P (c, x) ∈ I for every c ≠ 0. ForI is a Zariski closed subset, one may infer that in λ f + c · P (c, x) ∈ I holds even for c = 0.Consequently, in λ f ∈ I and this proves the assertion.The preceding result can be proved in a more general setting. The field K in our contextis of characteristic zero, but the theorem also holds for a field of characteristic p ≥ 0. Theproof of this more general version is nearly the same as the proof presented above. It canbe found in [6], proof of Theorem 15.23, pp. 356,357.Corollary 2.6. Every Borel-fixed ideal I ⊂ R is a monomial ideal.Proof. If I is Borel-fixed, then I is fixed under all upper triangular matrices in G. Inparticular, I is fixed under all diagonal matrices in G. By the preceding theorem I mustbe monomial.