MEMOIRS

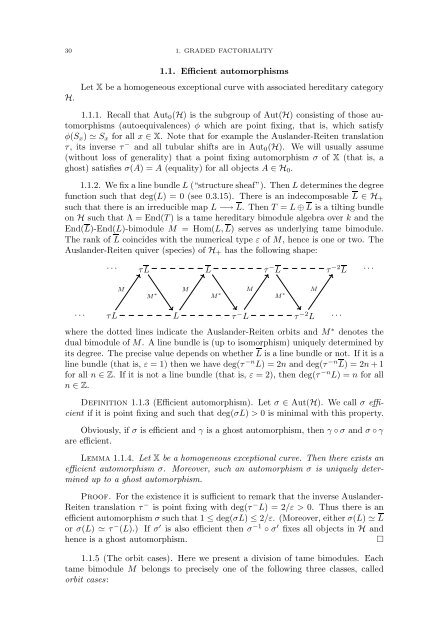
30 1. GRADED FACTORIALITYH.1.1. Efficient automorphismsLet X be a homogeneous exceptional curve with associated hereditary category1.1.1. Recall that Aut 0 (H) is the subgroup of Aut(H) consisting of those automorphisms(autoequivalences) φ which are point fixing, that is, which satisfyφ(S x ) ≃ S x for all x ∈ X. Note that for example the Auslander-Reiten translationτ, itsinverseτ − and all tubular shifts are in Aut 0 (H). We will usually assume(without loss of generality) that a point fixing automorphism σ of X (that is, aghost) satisfies σ(A) =A (equality) for all objects A ∈H 0 .1.1.2. We fix a line bundle L (“structure sheaf”). Then L determines the degreefunction such that deg(L) = 0 (see 0.3.15). There is an indecomposable L ∈H +such that there is an irreducible map L −→ L. ThenT = L ⊕ L is a tilting bundleon H such that Λ = End(T ) is a tame hereditary bimodule algebra over k and theEnd(L)-End(L)-bimodule M =Hom(L, L) serves as underlying tame bimodule.The rank of L coincides with the numerical type ε of M, hence is one or two. TheAuslander-Reiten quiver (species) of H + has the following shape:··· τLM ∗ LM ∗ τ − LMMτ −2 L ···MM ∗··· τLLτ − Lτ −2 L ···where the dotted lines indicate the Auslander-Reiten orbits and M ∗ denotes thedual bimodule of M. A line bundle is (up to isomorphism) uniquely determined byits degree. The precise value depends on whether L is a line bundle or not. If it is aline bundle (that is, ε =1)thenwehavedeg(τ −n L)=2n and deg(τ −n L)=2n +1for all n ∈ Z. If it is not a line bundle (that is, ε = 2), then deg(τ −n L)=n for alln ∈ Z.Definition 1.1.3 (Efficient automorphism). Let σ ∈ Aut(H). We call σ efficientif it is point fixing and such that deg(σL) > 0 is minimal with this property.Obviously, if σ is efficient and γ is a ghost automorphism, then γ ◦ σ and σ ◦ γare efficient.Lemma 1.1.4. Let X be a homogeneous exceptional curve. Then there exists anefficient automorphism σ. Moreover, such an automorphism σ is uniquely determinedup to a ghost automorphism.Proof. For the existence it is sufficient to remark that the inverse Auslander-Reiten translation τ − is point fixing with deg(τ − L)=2/ε > 0. Thus there is anefficient automorphism σ such that 1 ≤ deg(σL) ≤ 2/ε. (Moreover,eitherσ(L) ≃ Lor σ(L) ≃ τ − (L).) If σ ′ is also efficient then σ −1 ◦ σ ′ fixes all objects in H andhence is a ghost automorphism.□1.1.5 (The orbit cases). Here we present a division of tame bimodules. Eachtame bimodule M belongs to precisely one of the following three classes, calledorbit cases:M