MEMOIRS

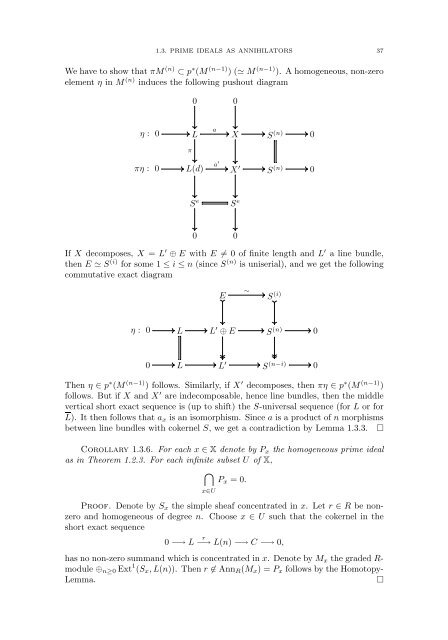
1.3. PRIME IDEALS AS ANNIHILATORS 37We have to show that πM (n) ⊂ p ∗ (M (n−1) )(≃ M (n−1) ). A homogeneous, non-zeroelement η in M (n) induces the following pushout diagram0 0η : 0LaX S (n) 0ππη : 0L(d)a ′ X ′ S (n) 0S eS e0 0If X decomposes, X = L ′ ⊕ E with E ≠ 0 of finite length and L ′ a line bundle,then E ≃ S (i) for some 1 ≤ i ≤ n (since S (n) is uniserial), and we get the followingcommutative exact diagramE∼S (i)η : 0 L L ′ ⊕ E S (n) 00 L L ′ S (n−i) 0Then η ∈ p ∗ (M (n−1) ) follows. Similarly, if X ′ decomposes, then πη ∈ p ∗ (M (n−1) )follows. But if X and X ′ are indecomposable, hence line bundles, then the middlevertical short exact sequence is (up to shift) the S-universal sequence (for L or forL). It then follows that a x is an isomorphism. Since a is a product of n morphismsbetween line bundles with cokernel S, we get a contradiction by Lemma 1.3.3. □Corollary 1.3.6. For each x ∈ X denote by P x the homogeneous prime idealas in Theorem 1.2.3. For each infinite subset U of X,⋂P x =0.x∈UProof. Denote by S x the simple sheaf concentrated in x. Letr ∈ R be nonzeroand homogeneous of degree n. Choose x ∈ U such that the cokernel in theshort exact sequence0 −→ L −→ rL(n) −→ C −→ 0,has no non-zero summand which is concentrated in x. DenotebyM x the graded R-module ⊕ n≥0 Ext 1 (S x ,L(n)). Then r ∉ Ann R (M x )=P x follows by the Homotopy-Lemma.□