2. Kinetics of Particles: Newton's Second Law ∑ ∑ ∑

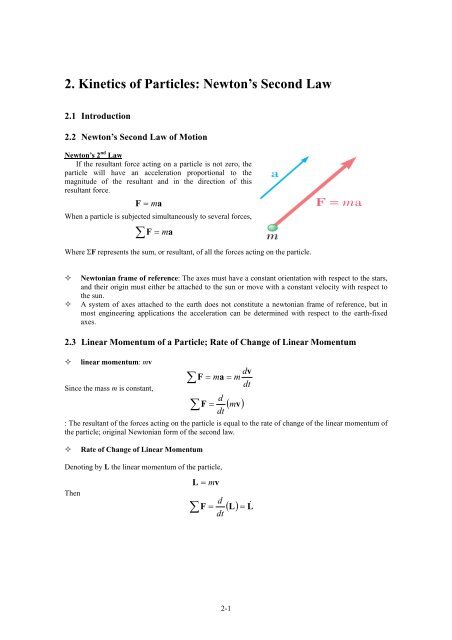
2. Kinetics of Particles: Newton’s Second Law2.1 Introduction2.2 Newton’s Second Law of MotionNewton’s 2 nd LawIf the resultant force acting on a particle is not zero, theparticle will have an acceleration proportional to themagnitude of the resultant and in the direction of thisresultant force.F = maWhen a particle is subjected simultaneously to several forces,∑F = maWhere ΣF represents the sum, or resultant, of all the forces acting on the particle.Newtonian frame of reference: The axes must have a constant orientation with respect to the stars,and their origin must either be attached to the sun or move with a constant velocity with respect tothe sun.A system of axes attached to the earth does not constitute a newtonian frame of reference, but inmost engineering applications the acceleration can be determined with respect to the earth-fixedaxes.2.3 Linear Momentum of a Particle; Rate of Change of Linear Momentum linear momentum: mvdv∑F = ma= mdtSince the mass m is constant,d∑F = ( mv)dt: The resultant of the forces acting on the particle is equal to the rate of change of the linear momentum ofthe particle; original Newtonian form of the second law.Rate of Change of Linear MomentumDenoting by L the linear momentum of the particle,ThenL = mv∑F =ddt( L) = L&2-1

2.4 Systems of UnitsInternational system of Units (SI Units): absolute system of units- length: meter (m)- mass: kilogram (kg)- time: second (s)‣ force: newton (N):1N = (1kg) (1m/s 2 ) =1 kg.m/s 2U.S. customary units: gravitational system of units- length: foot (ft)- force: pound (lb)- time: second (s)‣ mass: slug1slug = (1 lb) / (1ft/s 2 ) = 1 lb. s 2 /ftConversion from One System of Units to Another- Length: 1 ft = 0.3048 m- Force: 1 lb = 4.448 N- Mass: 1slug = 14.59 Kg1 pound-mass = 0.4536 kg2.5 Equations of Motion Rectangular Components∑∑F =Fx∑dvF = ma= mdt∑( Fxi+ Fyj + Fzk) = ma= m( axi+ ayj + azk)max= mx&∑ Fy= may= my && ∑ Fz= maz=& = mz &&Tangential and Normal Components∑∑F =t∑F = ma =( F e + F e ) = ma= m( a e + a e )tttndvmdtn∑Fn= mantt= m2vρnn2-2

2.6 Dynamic equilibriumDynamic equilibrium∑∑F − ma= 0F − mtdvdt= 0∑Fn2v− m = 0ρInertia vector / force (-ma, -mv 2 /ρ): Particles offer resistance force when we try to set them inmotion or when we try to change the conditions of their motion. Not acting forces; Shadow forcesExample 2.4)The 12-lb block B starts from rest and slides on the 30-lbwedge A, which is supported by a horizontal surface.Neglecting friction, determine (a) the acceleration of the sedge,(b) the acceleration of the block relative to the sedge.Ans)Kinematicsa A : horizontal acceleration of wedge Ba B : acceleration of block Ba B/A : relative acceleration of block B to wedge A.a = a + aKineticsWe draw the free-body diagrams of the wedge and of the block and apply Newton’s 2 nd law.BAB AWedge A:∑N1Fx0.5N1= mosin 30=AaA= mAaA( W Ag) a A(1)Block B:∑−WaFBB A∑Fx= msin 30= ay( a )o WBN −WBcos30 = −ga. Acceleration of wedge A:N 1 in (1) (3)a A= 5.07ft sA= mBoW=gcos30Bb. Acceleration of Block B relative to wedge A:a A (2)2a B A= 20.5ft sBxo( a )BBaAcos30+ g sin 301 Ay2aooW−gsin 30oBaB A(2)(3)2-3

Example 2.5)The bob of a 2-m pendulum describes an arc of circle in a vertical plane. If the tension in the cord is 2.5times the weight of the bob for the position shown, find the velocity and the acceleration of the bob in thatposition.HomeworkProblems 12.10, 12.15, 12.16, 12.20,12.45, 12.53, 12.59Ans)a n : acceleration of the bob toward Oa t : acceleration of the bob perpendicular to an and toward left.∑mg sin 30a2.5mg− mg cos30avt∑n2F = mat= g sin 30Fn = ma= 16.03= ρanot= manot= 4.92( m s )= ± 5.66o2( m s )= ma2v=ρ( m / s)n2.7 Angular Momentum of a Particle; Rate of Change of Angular MomentumFigure 12.12 Angular Momentum (moment of momentum): The moment about O of the vector mv, H O :H O= r × mvNote that H O is a vector perpendicular to the plane containing r and mv and of magnitudeH O= rmvsinφWhere φ is the angle between r and mv.The unit of angular momentum is in SI units2-4

Thus∑( & 2&− rθ) F = m( r && θ + r&& θ )F r= m r ∑ θ22.9 Motion under a Central Force: Conservation of Angular MomentumFig. 12.13 ~12.15When the only force acting on a particle P is a force F directed toward or away from a fixed point O, theparticle is said to be moving under a central force, and the point O is referred to as the center of force.Since the line of action of F passes through O, we must have ∑M= 0 at any given instant.OThereforeH &O= 0H = constantO: The angular momentum of a particle moving under a central force is constant, in both magnitude anddirection. Recalling the definition of the angular momentum,r × mv = HO= constant: Thus, a particle under central force moves in a fixed plane perpendicular to H O .Areal Velocity:H O= mr 2 θ &2-6

Recalling2H = mr &Oθ = constant2r & θ = h = constantwhere h is the angular momentum per unit mass. In Fig.12.15dA =dAdt=12122r dθr2dθdtwhere r is assumed constant during dt. Thus, when aparticle moves under a central force, its areal velocity isconstant.2.10 Newton’s Law of GravitationFig. 12.16In his law of universal gravitation, Newton states that two particles of masses M and m at a distance rfrom each other attract each other with equal and opposite forces F and –F directed along the line joiningthe particles. The common magnitude F of the two forces isMmF = G2rwhere G is a universal constant, called the constant of gravitation of( )2−123G = 66.73 ± 0.03 × 10 m kg.sSince the force exerted by the earth on a body of mass m located on or near its surface is defined as theweight W of the body,MmW = mg = G2RMg = G2Rwhere M is the mass of the earth and R the radius of the earth.2-7

Example 2.7) A block B of mass m can slide freely on a frictionless arm OA which rotates in a horizontalplane at a constant rate . Knowing that B is released at a distance r 0 from O, express as a function of0r, (a) the component v r of the velocity B along OA, (b) the magnitude of the horizontal force F exerted onB by the arm OA.Ans)Equations of Motion:(a) Component v r of Velocity:∑∑FFrθ= mar= maθθ & ( )& 20 = m && r − rθF = m r( && θ + 2r&& θ )(1)(2)vr= r&dvrdvrdr dvr&& r = v&r= = = vrdt dr dt drSubstituting for & r& in (1), recalling that & θ = & θ , and separating the variables,2&& r = r & θv2r(b) Horizontal Force Fdvr2vr= r & θOdr2vrdvr= r & θOdrv = & θr= & θO2O2 2( r − r )r2O− r2OOEq. (2) yields& θ = & θO&& θ = 0r & = vr2 2 22mOr − rOF = & θ2-8

HomeworkProblems 12.66, 12.72, 12.86, 12.891.11 Trajectory of a Particle under a Central ForceConsider a particle P moving under a central force F.The governing equations of motion areEq. (2.32) is replaced by the expressionwhich leads toThen− F =0 = m r( & 2m && r − rθ)( && θ + 2r&& θ )2 dθr = hdtdθ=dtr&=drdth&& r = −rh2rdr dθh= =2dθdt rdr&dr&dθh&& r = = =2dt dθdt r222d2dθ⎛ 1 ⎞⎜ ⎟⎝ r ⎠dr d ⎛ 1 ⎞= −h⎜ ⎟dθdθ⎝ r ⎠dr&h=2dθrd ⎡ d ⎛ 1 ⎞⎤⎢−h ⎜ ⎟dθ⎥⎣ dθ⎝ r ⎠⎦(2.31)(2.32)(2.34)(2.36)Substituting for θ and & r&u = 1/r, we obtain&& r = −−FmFmfrom (2.34) and (2.36), respectively, in Eq. (2.31) and introducing the function+ r & θF 2 3 2− + h u = −hum2d u F+ u =22 2dθmh u22⎛ h ⎞+ r⎜2⎟⎝ r ⎠h= −rh= −r222222d ⎛ 1 ⎞2⎜ ⎟dθ⎝ r ⎠2d ⎛ 1 ⎞2⎜ ⎟dθ⎝ r ⎠2d u2dθ2.12 Application to Space MechanicswhereGMmF = = GMmu2r22-9

M = massoftheearthm = massofaspacevehicler = dis tan cefrom thecenter oftheearth tothevehicleu = 1 rWe obtain the differential equationThe homogeneous solution isuh= C cosθ+ Dsinθ= C cos θ −θ0GMup=2hu = uh+ up1 GM= + C cosθ2(2.39)r hwhere the polar axis is chosen and θ 0 = 0. The origin O of thecoordinates, which is located at the center of the earth, is a focus ofthe conic section (ellipse, parabola, hyperbola). The ratio of theconstants C and GM/h 2 defines the eccentricity ε of the conic section;lettingWe can write Eq. (2.39) in the formThe equation represents three possible trajectories.1. ε > 1, hyperbola2. ε = 1, parabola3. ε < 1, ellipseSee Fig. 12.18.2d u GM+ u =22dθh= CGM h= constant2ChGMε = 21 GM= ( 1+ε cosθ )h2r( )2.13 Kepler’s Laws of Planetary Motion1. Each planet describes an ellipse, with the sun located at one of its foci.2. The radius vector drawn from the sun to a planet sweeps equal areas in equal times.3. The squares of the periodic times of the planets are proportional to the cubes of the semi-major axes oftheir orbits.HomeworkProblems 12.125, 12.126, 12.1332-10