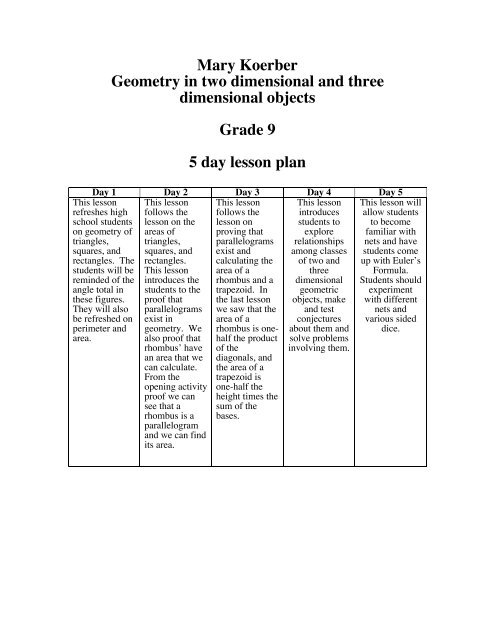
Mary Koerber Geometry in two dimensional and three dimensional ...

Developmental Activity Ditto handout1)8cm12cmPerimeter:Area:2)BC = 3.32 cmCCA = 6.85 cmCD = 2.40 cmDDB = 2.29 cmBAB = 4.13 cmAPerimeter:Area:Koeber – Page 5

3)Perimeter:AArea:m AB = 14.97 cmm AE = 6.18 cmm CA = 8.86 cmBCD4)Square:m BC = 7.27 cmm DC = 6.46 cm4cmArea:Perimeter:Koeber – Page 6

3.)i = 8 mj = 17 mh = 15 mP =_____________A=_____________Find the area and perimeter of the rectangle.1. 6 ft 7 ftP = _________ ftA = _________ square ft2. 6 km 9 kmP = _________ kmA = _________ square km3.) 11 mm 6 mmP = _________ mmA = _________ square mm4.) 18.4 mi 14.8 miP = _________ miA = _________ square miKoeber – Page 8

Opening ActivityFor the opening activityall triangles add up to 180 °all squares add up to 360 °Developmental Activity1) 8cm 12cmPerimeter: 8+8+12+12=40cmArea: 12*8=962) Perimeter: 3.32+6.85+4.13=14.3Area: =4.9563) Perimeter: 7.27+8.86+14.97= 31.1cmArea: =22.46434) Square: 4cmArea: 4+4+4+4=16cmPerimeter:4*4=16Closing ActivityA photocopied handout is given to accompany the closing activityto show how to fold the triangle.Homework AssignmentgivenP1P = 56 ftA = 84 ft 21.)P = 69 cmA = 300 cm? 22.)P = 50 mA = 120 m 23.)P = 40 mA = 120 m 21.)P = 26 ftA = 42 ft 22.)P = 30 kmA = 54 km 23.)P = 34 mmA = 66 mm 24.)P = 66.4 miA = 33.2 mi 2Koeber – Page 9

Day 2Name : Mary KoerberMathematical Concepts Addressed : Proof that parallelograms exist and the area of a____rhombus________________________________________________________________Grade Level : Grade 9Textbook : Geometry – Tools for Changing a World _____________________________This lesson follows the lesson on the areas of triangles , squares, and rectangles. Thislesson introduces the students to the proof that parallelograms exist in geometry. We alsoproof that rhombus’ have an area that we can calculate. From the opening activity proofwe can see that a rhombus is a parallelogram and we can find its area.Objectives:• To show students an introduction to proof.• To show the students the proof that a rhombus exists and to calculate its area.• To show students the formula to calculate the area of a trapezoid.• To see the angular measurements of a rhombus and how we use them to obtain thearea equation.Prerequisites:• Students should know logical steps of proof.• Students should know what a parallelogram is.• Students should have a firm grasp on algebra and angles.Materials and Facilities:• A classroom with desks, overhead projector, and chalkboard are needed.• Students will be receiving a handout for developmental and closing activities.Koeber – Page 10

Performance Standards:The NYS Learning Standards for mathematics that are addressed in this topic isMathematical Reasoning. This is used because we prove that a parallelogram exists andthen we use this conjecture to show the equation of a rhombus.The NCTM Principles and Standards for School Mathematics states that students mustestablish the validity of geometric conjectures using deduction, prove theorems, andcritique arguments made by others. In this lesson we prove the geometric figure of aparallelogram and make conjectures to make the equation of a rhombus.Opening Activity:In the opening activity the students will be guided through the proof that a parallelogramexists with the teacher. We are given that segment AC and segment BD bisect each othera E (view teacher’s notes). Then there are several other conjectures that we can say,such as angle AEB is congruent to angle CED, because vertical angles are congruent.By the same reasoning, we can say that angle BEC is congruent to angle DEA. We canalso see that segment AE is congruent to segment CE, because of the definition of asegment bisector. From here we can say that triangle AEB is congruent to triangle CEDand triangle BEC is congruent to triangle DEA; both by side- angle- side. Bycorresponding parts of congruent triangles are congruent, we have that angle BAE iscongruent to angle DCE and angle ECB is congruent to angle EAD. So if we havecongruent alterior angles then we have that segment AB is parallel to segment CD andsegment BC is parallel to segment AD. After all of these steps we can finally say thatABCD is a parallelogram.Developmental Activity:In the beginning of the developmental activity we ask students several questions about acertain rhombus. These questions expand on what the students know about prooftechniques. The students will be required to know how to answer questions about sideangle side, corresponding parts of congruent triangles are congruent, and anglemeasurement. In the second part of the developmental activity we use the proof thatsegment AC is perpendicular to segment BD to show that the area of a rhombus is equalto one-half the product of the diagonals. We are first given that ABCD is a rhombus,and by definition of a rhombus segment AB is congruent to segment AD. Since segmentAC is a diagonal of a rhombus it bisects angle BAD, and therefore angle BAE and DAEare congruent. By the Reflexsive Property of Congruence we have that segment AE iscongruent to segment AE; and by side – angle - side we see that triangle ABE iscongruent to triangle ADE. By corresponding parts of congruent triangles are congruentwe get that angle AEB is congruent to angle AED; and because these angles are bothcongruent and supplementary , they are right angles. We can now finish that by thedefinition of perpendicular we have that segment AC is perpendicular to segment BD.Once we have proven this we can now develop another proof that allows us to use aformula to calculate the area. The proof will be given as a homework assignment, so thatthe students can see where the formula comes from. It is essential that the teacher goesover the homework with the students so that anyone who gets the proof wrong knowswhere they went wrong.Closing Activity:In the closing activity we tell the students the formula to the area of a rhombus; and westate that a square is also a rhombus. Later in the homework the students will see whythe area is one-half the product of the diagonals. The teacher will also show the studentsthe formula to find the area of a trapezoid. The students will learn that in a trapezoid theparallel sides are the bases, and the non-parallel sides are the legs. Also, the height is theperpendicular distance between the two parallel bases.Koeber – Page 11

Opening Activity (done on overhead)BCGiven: AC and BD bisect each other at E.EProve: ABCD is a parallelogram. A DAC and BD bisect each other at EGiven

Day 3Name : Mary KoerberMathematical Concepts Addressed : Finding the area of polygons by using the areas ofpreviously known areas and measurement of the interior angles in polygons._________Grade Level : Grade 9 ___________________________________________Textbook : Geometry – Tools for Changing a World _____________________________This lesson follows the lesson on proving that parallelograms exist and calculating thearea of a rhombus and a trapezoid. In the last lesson we saw that the area of a rhombus isone-half the product of the diagonals, and the area of a trapezoid is one-half the heighttimes the sum of the bases.Objectives:• To show students how to use different shapes to find the area of polygons.• To show the students what the total sum of the angles are in certain polygons.Prerequisites:• Students should know what polygons are.• Students should know how to calculate area in polygons from previous lesson plans.• Students should have a firm grasp on algebra and angles.Materials and Facilities:• A classroom with desks, overhead projector, and chalkboard are needed.• Students will be receiving a handout for developmental and closing activities.• Students need a ruler and will receive triangles, squares, rectangles, pentagons,hexagons, and octagons from the teacher.Performance Standards:The NYS Learning Standards for mathematics that are addressed in this topic isModeling/ Multiple Representations to provide a means of presenting, interpreting,communicating, and connecting mathematical information and relationships. Thisstandard is used because we try to fit the areas of figures that we already know intofigures that we don’t know to find their areas.The NCTM Principles and Standards for School Mathematics states that students mustexplore relationships ( including congruence and similarity) amount classes of two- andthree- dimensional geometric objects, make and test conjectures about them, and solveKoeber – Page 14

problems using them. As stated before we correlate this to the lesson by take the areas offigures that we already know to find the areas of figures that we don’t know.Opening Activity :For the opening activity the students will break up into groups of four and receivedifferent sized triangles, squares, rectangles, pentagons, hexagons, and octagons. Eachgroup will discuss how they can fit their shapes into the polygon that is provided. Byusing this technique the students can find the area of the unknown polygon from the areasof the known polygons.Developmental Activity:The developmental activity builds upon the opening activity. Again we use this sameconcept of using the areas of previously known shapes to find the area of shapes that wedo not have a formula for. In this activity we first start by breaking a hexagon down intothree pieces. We have two of the same triangles and one rectangle. The base of thehexagon is 15 cm and the height is 25 cm. With a little measurement we find that theheight of the triangles is 5 cm. We now have enough information to find the area of thetwo triangles and the square. The area of one triangle is 62.5 cm, so the total area of bothtriangles 125 cm. The area of the rectangle is 375 cm, so the area of the hexagon is 125cm plus 375 cm, which is 500 cm 2 . We then move on to finding the area of an octagon.This is about the same procedure, except we will have two trapezoids and one rectangle.First have the students “cut up” the octagon into the shapes stated above. When we breakup the octagon we will get the dimensions of 5 cm for the width of the rectangle and 10cm for the height. When we calculate the area we get 50 cm 2 . For the trapezoids we getthat the bases are 3 cm and 10 cm and the height is 2 cm. When we calculate the area ofone trapezoid we get 13 cm 2 , and for two we get 26 cm 2 . So the total area of the octagonis 76 cm 2 when we add the areas together. When we are done with this activity have thestudents work one other shapes and see if they can find the areas.Closing Activity:In the closing activity the teacher will have the students draw polygons that have4,5,6,7,and 8 sides on their papers. The teacher will then instruct the students to use aruler to make triangles within each polygon by drawing all the diagonals from one vertexto the middle of the polygon. This is an easy way to find the total interior anglemeasurement within multi-sided polygons. Have the students count up the number oftriangles within the polygons and multiply that number by 180 degrees to get the totalinterior angle measurement. By following a pattern the students should see that thisworks for any type of polygon.Koeber – Page 15

Opening Activity (day 3)Go over finding the area of a trapezoid.Example:24.3 cm8.5cmArea: 144.5 cm 29.7cmHand out different sized triangles, squares, rectangles, pentagons, hexagons, andoctagons to each group. Have each group discuss how they can find the area of theseshapes without knowing a formula for these specific shapes.Students should come up with that the area can be found by adding up the area of alreadyknown shapes that fit inside the new shape.Koeber – Page 16

Developmental Activity ( teachers notes)Find the area of the polygon:5cm25cm15cm5cmFirst find the area of the triangles: (25 cm * 5 cm) / 2 = 62.5cm 2There is two of them so 2*62.5 cm =125cm 2Now find the area of the rectangle: 25 cm *15 cm = 375cm 2The total sum of the area of the hexagon is; 375 cm 2 +125 cm 2 = 500cm 2Have students in groups draw polygons with 4,5,6,7,and 8 sides.Divide each polygon into triangles by drawing all the diagonals from one vertexMultiply the number of triangles by 180 to find the sum of the measures of the interiorangles of each polygon.Have students try to come up with the polygon interior angle-sum Theorem by inductivereasoning. Hint: tell them to look for a hint in the pattern in their table.Koeber – Page 17

This will be part of homework.Opening ActivityIn the opening activity we must recallarea of a triangle = (1/2)b?harea of a rectangle = b?harea of a rhombus = (length of diagonal 1 ? length of diagonal 2/2)area of a trapezoid = ((length of base 1 + length of base 2)?h/2)We can use these areas to find the areas of teh shapes we hand out tothe students (pentagon, hexagon, octagon).Developmental ActivityArea of the triangles = (25 cm ?5 cm /2) = 62.5 cm(^2) = 62.5 cm(^2) ? 2 = 125 cm(^2)Area of rectangle = 25 cm ? 15 cm = 375 cm(^2)Total sum of areas = 375 cm(^2) + 125 cm(^2) = 500 cm(^2)Closing Activity3 -sided total interior angle measurement - 180deg4 - sided - 360deg5 - sided - 540deg6 - sided - 720deg7 - sided - 900deg8 - sided - 1080degThe students should come up with the Polygon Interior Angle - Sum Theoremfrom doing thisThe Polygon Interior Angle - Sum Theorem states that the sum of the measuresof the interior angles of an n-gon is (n - 2) ? 180degHomework Assignment -pg 80Problems 10,11,12,14,15,16,19,20,21,23,24,2510.) 108;7211.) 140;4012.) 160;2014.) 515.) 1016.) 2019.) 10220.) 14521.) y = 103 ; z = 7023.) x = 69 ; w = 111Koeber – Page 18

Day 4Name : Mary KoerberMathematical Concepts Addressed : Surface area in 3-D objects._________Grade Level : Grade 9 ___________________________________________Textbook : Geometry – Tools for Changing a World _____________________________Objectives:Have students learn how to calculate Surface area.Have students understand how to use surface area in real life situations.Equipment: This lesson should be taught in a classroom equipped with a writing boardor overhead. Materials needed are scissors, pipe cleaners, and rulers.Performance Standards: Analyze properties and determine attributes of two and threedimensional objects. Explore relationships among classes of two and three dimensionalgeometric objects, make and test conjectures about them, and solve problems involvingthem.NY State: Modeling / Representation and Measurement.Opening Activity: Show students a three dimensional figure such as a pyramid and askhow they think they can find the area of the three dimensional shape. Have discussionuntil idea is understood.Developmental Activity: Have students working in groups creating their own pyramidout of pipe cleaners. Have students use the ruler to make the measurements and havethem calculate the surface area of their polygon.Closing Activity: Review with a real world situation.Koeber – Page 19

Opening ActivityIntroduce a pyramid to students. Ask students how they think you can find the surfaceareas of this pyramid.Expected response: find the areas of the different parts and add them up.Discuss the difference between bases that are of a regular shape and bases that are not.Ask students which kind of shapes would be quicker to find out the surface area and askthem why.Expected response:A regular shape with a regular base . This way the area of one lateral side is needed sincethey will all be the same.Introduce how to calculate surface area and go into the developmental activity.Developmental Activity (Surface Areas)PyramidsHave students make their own regular pyramids out of pipe cleaners.Have students measure the slant height and the length of an edge of a base.The surface area of a regular pyramid is the sum of the lateral area and the area of thebase.s = side. l = slanted s - *lheightThe area of each lateral face is2 .Multiply this area by the number of sides on your base to get the lateral area.Find the area of the base and add it to the lateral area to get the surface area.After students have completed this activity, ask a few volunteers to discuss their pyramidthey made with the lengths and explain how they got the surface area.Koeber – Page 20

Developmental Activity (Surface Areas)PyramidsHave students make their own regular pyramids out of pipe cleaners.Have students measure the slant height and the length of an edge of a base.The surface area of a regular pyramid is the sum of the lateral area and the area of thebase.s = side. l = slanted s - *lheightThe area of each lateral face is2 .Multiply this area by the number of sides on your base to get the lateral area.Find the area of the base and add it to the lateral area to get the surface area.After students have completed this activity, ask a few volunteers to discuss their pyramidthey made with the lengths and explain how they got the surface area.Closing ActivityRelating to the real worldThe Great Pyramid at Giza, Egypt, was built about 2580 B.C. as a final resting place forPharoe Khufu. At the time it was built, its height was about 481 ft. Each edge of thesquare base was about 756 ft long. What was the lateral area of the pyrami??Koeber – Page 21

ABC. The legs of DABC are the height of the pyramid and the apothem 756of the base. Theheight of the pyramid is 481 ft. The apothem of the base is or 378 ft. You can usePythagorean Theorem to find the slant height.l 2 = 481 2 + 378 2l = (481 2 + 378 2 )l = 611.75567Now use the formula for the lateral area of a pyramid. The perimeter of the base isapproximately 4 * 756, or 3024 ft.L.A.= 3024*611.755672=924974.57. The L.A was about 925,000 ft 2 .2Koeber – Page 22

HomeworkShow all WORK!• The Transamerica building in San Fransisco is a pyramid. The length of each edge ofthe square base is 149 ft and the slant height of the pyramid is 800 ft. What is theLateral Area of the pyramid???2) Make up your own shape with measurements and find the surface area of your shape:Koeber – Page 23

Assignment Teacher Notes• The Transamerica building in San Fransisco is a pyramid. The length of each edge ofthe square base is 149 ft and the slant height of the pyramid is 800 ft. What is theLateral Area of the pyramid???800*1492* 4 = 238, 400 ft 22) Make up your own shape with measurements and find the surface area of your shape:Koeber – Page 24

Day 5Lesson PlanObjectives:Students will understand what nets are and how to create them.Students will be able to create three dimensional models using nets.Equipment:Different sided dice, overhead, scissors, tape, and dittos.Performance Standards:Analyze characteristics and properties of two and three dimensional geometric shapesand develop mathematical arguments about geometric relationships.Use visualization, spatial reasoning, and geometric modeling to solve problems.NY State: Modeling/RepresentationPrerequisites:Students should know how to find perimeter, area, and know basic attributes aboutshapes.Opening Activity:Have Students work in groups of four.Pass out opening activity ditto to students. Have students cut the nets out of the ditto tomake three dimensional models.Developmental Activity:Using a cube, a pyramid, and the other three models the students made in the openingactivity, we are going to work together to make a table containing the Polyhedron,Number of faces (F), Number of vertices (V), and Number of edges (E).PolyhedronCubePyramidFigure 1Figure 2Figure 3Number of faces(F)Number of vertices(V)Number of edges(E)Koeber – Page 25

Closing Activity:Have students try do draw their own net that has to fold to form a three dimensionalfigure.Have students use the Euler’s Formula they came up with to make sure it works withtheir figure.Students should have various figures and notice that Euler’s formula works for all ofthem.Opening ActivityEuler’s FormulaSpace figures and Nets:Review:Most buildings are polyhedrons. A polyhedron is a three dimensional figure whosesurfaces are polygons. The Polygons are the faces of the polyhedron. An edge is asegment that is the intersection of two faces. A vertex is a point where edges intersect.A net is a two dimensional pattern that you can fold to form a three dimensional figure.Packagers use nets to design boxes.Have Students work in groups of four.Pass out opening activity ditto to students. Have students cut the nets out of the ditto tomake three dimensional models.Ask students if the nets can look different to make the three dimensional shape.Koeber – Page 26

Developmental ActivityUsing a cube, a pyramid, and the other three models the students made in the openingactivity, we are going to work together to make a table containing the Polyhedron,Number of faces (F), Number of vertices (V), and Number of edges (E).PolyhedronCubePyramidFigure 1Figure 2Figure 3Number of faces(F)Number of vertices(V)Number of edges(E)Look for a pattern in the pattern. Write a formula E in terms of F and V.Students should be able to come up with this formula. Discuss how this relationship istrue for any polyhedron. This formula is known as Euler’s Formula.Closing ActivityHand out different sided dice to check Euler’s Formula.Have students try do draw their own net that has to fold to form a three dimensionalfigure.Have students use the Euler’s Formula they came up with to make sure it works withtheir figure.Students should have various figures and notice that Euler’s formula works for all ofthem.Homework: from textbook.Koeber – Page 27

Opening Activity handoutKoeber – Page 28

Koeber – Page 29