Beam Weapons

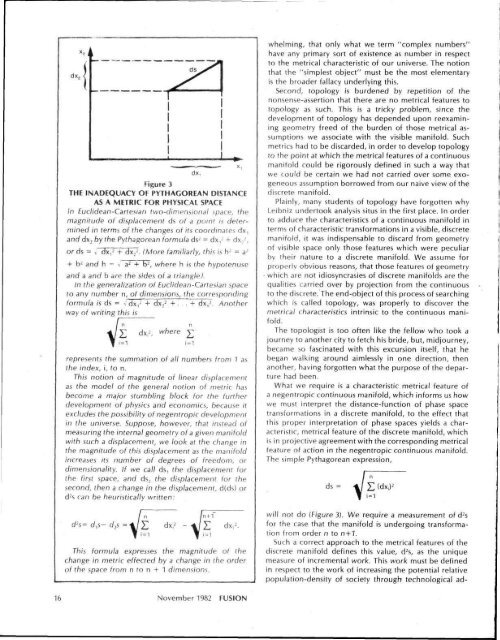
Figure 3THE INADEQUACY OF PYTHAGOREAN DISTANCEAS A METRIC FOR PHYSICAL SPACEIn Euclidean-Cartesian two-dimensional space, themagnitude of displacement ds of a point is determinedin terms of the changes of its coordinates dx,and dx, by the Pythagorean formula ds 2 = dx, 2 + dx, 2 ,or ds = v 1 dx, 2 + dx 2 2 . (More familiarly, this is h 2 = a 2+ b 2 and h = %• a 2 + b 2 , where h is the hypotenuseand a and b are the sides of a triangle).In the generalization of Euclidean-Cartesian spaceto any number n, of dimensions, the correspondingformula is ds = J dx, 2 + dx 2 2 +. . + dx 2 n . Anotherway of writing this isVnn£ dx 2 , where £1-1 wrepresents the summation of all numbers from 1 asthe index, i, to n.This notion of magnitude of linear displacementas the model of the general notion of metric hasbecome a major stumbling block for the furtherdevelopment of physics and economics, because itexcludes the possibility of negentropic developmentin the universe. Suppose, however, that instead ofmeasuring the internal geometry of a given manifoldwith such a displacement, we look at the change inthe magnitude of this displacement as the manifoldincreases its number of degrees of freedom, ordimensionality. If we call ds, the displacement forthe first space, and ds 2 the displacement for thesecond, then a change in the displacement, d(ds) ord 2 s can be heuristically written:jn/n+fd 2 s=d 1 s-d 2 s=-^2: dXi 2 --l/Z dx; 2 .This formula expresses the magnitude of thechange in metric effected by a change in the orderof the space from n to n + 1 dimensions.whelming, that only what we term "complex numbers"have any primary sort of existence as number in respectto the metrical characteristic of our universe. The notionthat the "simplest object" must be the most elementaryis the broader fallacy underlying this.Second, topology is burdened by repetition of thenonsense-assertion that there are no metrical features totopology as such. This is a tricky problem, since thedevelopment of topology has depended upon reexamininggeometry freed of the burden of those metrical assumptionswe associate with the visible manifold. Suchmetrics had to be discarded, in order to develop topologyto the point at which the metrical features of a continuousmanifold could be rigorously defined in such a way thatwe could be certain we had not carried over some exogeneousassumption borrowed from our naive view of thediscrete manifold.Plainly, many students of topology have forgotten whyLeibniz undertook analysis situs in the first place. In orderto adduce the characteristics of a continuous manifold interms of characteristic transformations in a visible, discretemanifold, it was indispensable to discard from geometryof visible space only those features which were peculiarbv their nature to a discrete manifold. We assume forproperly obvious reasons, that those features of geometry• which are not idiosyncrasies of discrete manifolds are thequalities carried over by projection from the continuousto the discrete. The end-object of this process of searchingwhich is called topology, was properly to discover themetrical characteristics intrinsic to the continuous manifold.The topologist is too often like the fellow who took ajourney to another city to fetch his bride, but, midjourney,became so fascinated with this excursion itself, that hebegan walking around aimlessly in one direction, thenanother, having forgotten what the purpose of the departurehad been.What we require is a characteristic metrical feature ofa negentropic continuous manifold, which informs us howwe must interpret the distance-function of phase spacetransformations in a discrete manifold, to the effect thatthis proper interpretation of phase spaces yields a characteristic,metrical feature of the discrete manifold, whichis in projective agreement with the corresponding metricalfeature of action in the negentropic continuous manifold.The simple Pythagorean expression,ds = i Z (dx,) 2will not do (Figure 3). We require a measurement of d 2 sfor the case that the manifold is undergoing transformationfrom order n to n + 1.Such a correct approach to the metrical features of thediscrete manifold defines this value, d 2 s, as the uniquemeasure of incremental work. This work must be definedin respect to the work of increasing the potential relativepopulation-density of society through technological ad-16 November 1982 FUSION