A Chaotic RC Oscillator

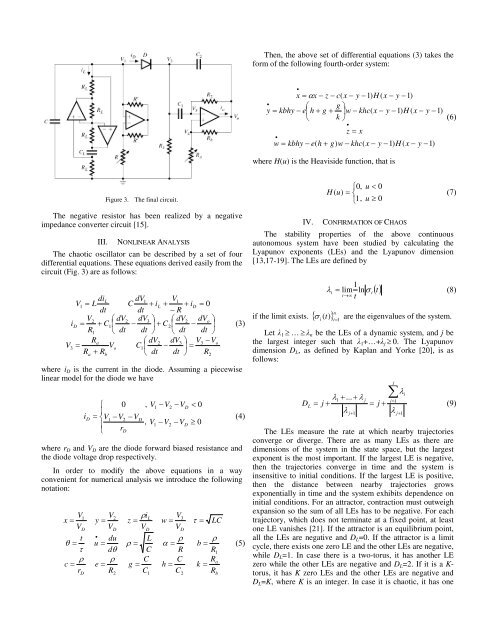
Then, the above set of differential equations (3) takes theform of the following fourth-order system:•x = αx − z − c(x − y −1)H ( x − y −1)•⎛ g ⎞y = kbhy − e⎜h + g + ⎟w− khc(x − y −1)H ( x − y −1)⎝ k ⎠•z = x•w = kbhy − e(h + g)w − khc(x − y −1)H ( x − y −1)(6)where H(u) is the Heaviside function, that isFigure 3. The final circuit.The negative resistor has been realized by a negativeimpedance converter circuit [15].III.NONLINEAR ANALYSISThe chaotic oscillator can be described by a set of fourdifferential equations. These equations derived easily from thecircuit (Fig. 3) are as follows:diLdV1V1V1 = L C + iL+ + iD= 0dt dt − RV2⎛ dV2dV3⎞ ⎛ dV2dVo⎞iD= + C1⎜− ⎟ + C2⎜− ⎟R1⎝ dt dt ⎠ ⎝ dt dt ⎠Ra⎛ dV2dV3⎞ V3−VV3= VoC1⎜− ⎟ =R + R ⎝ dt dt ⎠ Rabwhere i D is the current in the diode. Assuming a piecewiselinear model for the diode we have2o(3)⎧ 0 , V1−V2−VD< 0⎪iD= ⎨V1−V2−V(4)D⎪, V1−V2−VD≥ 0⎩ rDwhere r D and V D are the diode forward biased resistance andthe diode voltage drop respectively.In order to modify the above equations in a wayconvenient for numerical analysis we introduce the followingnotation:V1x =VDtθ =τρc =rDV2y =VD•duu =dθρe =R2ρiLz =VDLρ =CCg =C1V3w =VDρα =RCh =C2τ =LCρb =R1Rak =Rb(5)IV.⎧0,u < 0H ( u)= ⎨(7)⎩1,u ≥ 0CONFIRMATION OF CHAOSThe stability properties of the above continuousautonomous system have been studied by calculating theLyapunov exponents (LEs) and the Lyapunov dimension[13,17-19]. The LEs are defined by1λi= lim lnσi( t)(8)t →∞t} n i( ti=1if the limit exists. { σ ) are the eigenvalues of the system.Let λ 1 ≥ … ≥ λ n be the LEs of a dynamic system, and j bethe largest integer such that λ 1 +…+λ j ≥ 0. The Lyapunovdimension D L , as defined by Kaplan and Yorke [20], is asfollows:DLj+1j∑λiλ1 + ... + λji=1= j + = j +λλThe LEs measure the rate at which nearby trajectoriesconverge or diverge. There are as many LEs as there aredimensions of the system in the state space, but the largestexponent is the most important. If the largest LE is negative,then the trajectories converge in time and the system isinsensitive to initial conditions. If the largest LE is positive,then the distance between nearby trajectories growsexponentially in time and the system exhibits dependence oninitial conditions. For an attractor, contraction must outweighexpansion so the sum of all LEs has to be negative. For eachtrajectory, which does not terminate at a fixed point, at leastone LE vanishes [21]. If the attractor is an equilibrium point,all the LEs are negative and D L =0. If the attractor is a limitcycle, there exists one zero LE and the other LEs are negative,while D L =1. In case there is a two-torus, it has another LEzero while the other LEs are negative and D L =2. If it is a K-torus, it has K zero LEs and the other LEs are negative andD L =K, where K is an integer. In case it is chaotic, it has onej+1(9)