Nuclear and Particle Physics SubAtomic Physics

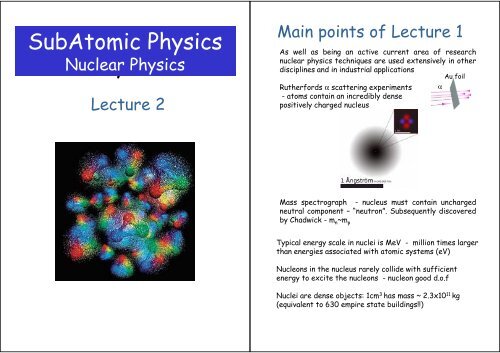
Nuclear SubAtomic and Physics ParticleNuclear PhysicsLecture 2Main points of Lecture 1As well as being an active current area of researchnuclear physics techniques are used extensively in otherdisciplines and in industrial applicationsAu foilRutherfords α scattering experiments- atoms contain an incredibly densepositively charged nucleusαDr Daniel WattsMass spectrograph - nucleus must contain unchargedneutral component – “neutron”. Subsequently discoveredby Chadwick - m n ~m pTypical energy scale in nuclei is MeV - million times largerthan energies associated with atomic systems (eV)Nucleons in the nucleus rarely collide with sufficientenergy to excite the nucleons - nucleon good d.o.fNuclei are dense objects: 1cm 3 has mass ~ 2.3x10 11 kg(equivalent to 630 empire state buildings!!)

1. Strong (nuclear) forceThe forces of nature• acts on all particles except leptons• always attractive (on average)• short range (10 -15 m)4. Gravitational force• acts between all particles with mass• always attractive• always presentbut inverse square lawshort rangeforceZAP10 -15 m2. Electromagnetic forceSTRONGEST FORCEF strong = 1m 2~ 1/R 2long rangem 1forceWEAKEST FORCEF grav ~ 10 -40 F strongbut dominant force in themacrocosmos (large masses)… binds Earth ,solar systemgalaxies…q 2~ 1/R 2long rangeq 1 force3. Weak force→ + + νproton electron(T 1/2 ~ 15 min.)neutrino• acts between all particles with charge• attractive/repulsive• always presentbut inverse square lawSECOND STRONGEST FORCEF em ~ 10 -2 F strongdominant force in atoms,molecules, solid bodies (binding)• acts on all particles• very short range (10 -18 m)• e.g. responsible for β decaySECOND WEAKEST FORCEF weak ~ 10 -7 F strongWhich forces are important to understanding the nucleus??Bound system of protons and neutronsInteracting under the competing influence ofAttractive nuclear (strong) and repulsiveElectromagnetic forces (between protons)Weak forces are negligible in terms of binding the nucleus,but important in that they can change neutrons ↔ protonsβ decay (see later)Nuclear force does not win over EM repulsion indefinitelyFinite number of naturally occurring atoms (Z≤ 92)Last completely stable nucleus Lead (Z=82)electromagnetic force (~Z 2 ) wins at Z≥92

NotesNotes

Strength of forces at different distance scalesQuantum mechanicsRevision of some basic concepts to be used later in the course• observable = physical, measurable quantity ⇔ operatorexamplestotal energy ⇔ Hamiltonianposition ⇔ xˆlinear momentum ⇔ pˆangular momentum ⇔ Lˆ2Ĥ• system described by wave function ψ(x,t) obeyingtime dependent Schrödinger equation (TDSE)Role of the different forces depends on the distance scaleExample: Which forces dominate in Rutherford’s α particlescattering measurement?Need to get within few fm of thenucleus for strong force to contributesignificantly. In Rutherford’sexperiment (~7 MeV) α’s cannot getwithin this range of the nucleus →therefore Coulomb force dominates.See Tutorial question !19779 Au(charge = +79e !)?Would need higher incidentα energies to see effectsof the strong force in thescattering → large deviationsfrom simple Coulombscattering2 2⎧ h ∂⎨ −⎩ 2m∂x2⎫ ∂+ V(x,t)⎬Ψ(x,t)= i h Ψ(x,t)⎭ ∂t• static potential - stationary states exist. Described by spatial wavefunction ψ(x) obeying time independent Schrödinger equation (TISE)⎧ 2 2h d⎨ −⎩ 2mdxeigenfunction2⎫+ V(x) ⎬ψE(x) = EψE(x)⎭Ĥψ (x) = Eψ (x)Eeigenvalue equation only satisfied by certain values of Eenergy is QUANTISEDmeasurements of total energy can only yield values which areeigenvalues of Hamiltonian operatorEeigenvalue

NotesNotes

2x2Orbital angular momentum ⇔ Lˆ2Lˆ = Lˆ + Lˆ + Lˆ square of magnitude of angular momentum2y2zIn spherical polar coordinates:2[ Lˆ ,Lˆ ] 0iLˆ2= −hImportant property:Lˆ2Choose:2⎡⎢⎣1sin θ∂ ⎛ ∂ ⎞ 1⎜sin θ ⎟ +∂θ⎝ ∂θ⎠ sin2∂2θ ∂φcommutes with any Cartesian component of angular momentum= both operators have simultaneous eigenfunctionsspherical harmonics Ylm (θ,φ)∂Lˆz = −ih z-component of angular momentum vector∂φ2• Eigenvalue equation for Lˆ2Lˆ Yml2 ml(θ,φ) = l ( l + 1) h Y (θ,φ) l = 0,1,2,3 ,...• Eigenvalue equation for LˆzmmLˆ Y (θ,φ) = mhY(θ, φ) − l ≤ m ≤ l in integer stepszlangular momentum is QUANTISEDlEigenstates of Lˆ2are DEGENERATE ⇔ (2l+1) possible valuesexperimental evidence: Stern and Gerlach experiment (spatial quantisation)2⎤⎥⎦orbital angular momentumquantum numbermagnetic momentumquantum numberN.B. we refer to a particle in a state of angular momentum l meaningl ( l + 1)hIntrinsic angular momentum ⇔No good classical analogueElectrons, protons, neutrons all have half integer spin: FERMIONSŜŜzhas eigenvalue s = ½has eigenvalue m s = ± ½Ŝspin upspin downAddition of angular momentum vectors in quantum mechanicswhen two states of angular momentum quantum numbers j 1 and j 2add (or couple) together,they form a state with definite TOTAL angular momentum j anddefinite j z component⏐j 1 -j 2 ⏐ ≤ j ≤ j 1 + j 2-j ≤ m ≤ jthese states can be expressed as linear combination of statesof the uncoupled basis {⏐j 1 ,m 1 ,j 2 ,m 2 >} with coefficients known• Eigenvalue equation for2ĴĴas Clebsch-Gordan coefficients2Total angular momentum JSum of orbital angular momentum and spin of nucleon J = L + S• Eigenvalue equation for2j,m = j(j + 1) hĴ zĴzj,mj,m = mhj,m − j ≤ m ≤ j1 3j = 0, ,1, ,2,...2 2in integer steps(2j+1) possible projectionsset of (2j+1) states { j,m } called MULTIPLET

NotesNotes

Central symmetric potentialPotential energy function V=V(r) is function of r only, not of θ and ϕSolution of TISE:• radial wavefunction R(r) solution of radial TISE:2( l 1)⎡ h 1 d ⎛ 2 d ⎞ l + h⎢−r + V(r) +2µ2⎜ ⎟r dr dr2⎣ ⎝ ⎠ 2µr2centrifugal potential(or barrier)⎤⎥R( r) = ER( r)⎦• spherical harmonics Y(θ,ϕ) solution of angular TISE:⎡− ⎢⎣1sin θu(r,θ, φ) = R(r)Y(θ,φθ,φ)∂ ⎛ ∂ 1sin θ⎞⎜ ⎟ +∂θ⎝ ∂θ⎠ sin2∂2θ ∂φ2⎤⎥Y θ,φ⎦( ) = λY( θ,φ)Parity πParity operator ⇒ inversion in the origin coordinatesPˆ uIn polar coordinates:nlmm( r, θ, φ) = Pˆ R( r) Y ( θ,φ)r → rθ → π − θφ → π + φr r→ −Behaviour of eigenfunction under parity transformation determinedby properties of spherical harmonics.ml m[ ] = R( r) Y ( π − θ, π + φ) = R( r)( − 1) Y ( θ,φ)lPˆunlmEigenfunctions have definite parity:ll( r, θ,φ) = ( − 1) u ( r, θ,φ)nlmEVEN (or positive) for even lODD (or negative) for odd llN.B. potential V(r) does not appear in angular equation⇒always solutions, no matter what form for V(r)Ylm ( θ,φ)Very general result following from spherical symmetry of potentialm| ( θ,φ)Y l2|Distance of eachpoint from the originproportional to themagnitude in thatdirectionPolar plots of sections at y=0 through spherical harmonicszz+0 2| Y0 ( θ , φ)|Even parityy+-0 2| Y1 ( θ,φ)|Odd parityWe will see in later lectures how conservation of total angular momentum andparity are of crucial importance in understanding nuclear structure andreactions This overview deals with the QM of stationary states produced in astatic potential. To calculate the probability of transitions between thesestates we need more advanced QM. (We will touch a little on this later whenwe look at electron scattering -Fermi’s golden rule)y+-+ -0 2| Y2 ( θ,φ)|Even parity+, - sign offunction ineach region

NotesNotes

The nucleus and its propertiesTime evolution of the nuclear chartNucleus = central part of an atomit contains A nucleonsA = Z (protons) + N (neutrons)atomic numbermass number(nucleon = proton or neutron)AXZ NZ ≡ X chemical symbol)Proton nmber (Z)A bit of nomenclature…NUCLIDE element with given N and ZISOTOPES elements with same Z but different NISOTONES elements with same N but different ZISOBARS elements with same AISOMERSelements in metastable (i.e. very long-lived) stateChart of nuclidesNeutron number (N)isotonesisobarsChart still being added to as experimental facilities improveWe are still quite some way from reaching the limits of nuclearexistence – particularly on the neutron rich side.New facilities coming online in the near future like FAIR inGermany (http://www.gsi.de/fair/) should remedy this!isotopes

NotesNotes

External nuclear propertiesCharge Ze: protons have +ve charge e = 1.6022x10 -19 Cneutrons have zero chargeneutral atom: (A,Z)contains Z electrons orbiting around nucleussymbolically:AZXN(Z ≡ X chemical symbol)Mass M:nuclear and atomic masses are expressed inATOMIC MASS UNITS (u)definition: 1/12 of mass of neutral 12 C ⇒ M( 12 C) = 12 u1u = 1.6605x10 -27 kg or 931.494 MeV/c 2 (E=mc 2 )M(A,Z) < Zm p + Nm ndifference:∆M = Zm p + Nm n - M(A,Z) ⇒ mass defect (excess)accounts for BINDING ENERGY of nuclei (see later)N.B. we typically use ATOMIC and not NUCLEAR masses⇒ mass of electrons also includedSize R: nuclear radii are expressed in fermis (fm) 1 fm = 10 -15 mcompare with atomic dimensions1 Å = 10 -10 mmatteressentiallyEMPTY SPACE!

NotesNotes

… more on nuclear sizeWhat do we define as nuclear size?NotesConsider the following:• the nucleus has a net positive charge Ze (Z protons)• take into account Coulomb + nuclear forceVBResulting potentialextends to ∞as 1/R 2Coulombrepulsivehas short(~10 -15 m) rangeDefine:barrier height B at a distancefrom centre R:B = Zze 24πε 00 RRrfor incident charge ze-V 0 nuclear attractiveR = POTENTIAL RADIUS ⇒ related to distribution of protons &neutrons AND the range of nuclear forcepotential radius > charge (or mass) radiusCHARGE RADIUS⇒ related to charge (proton) distribution

Notes