PDF (361 K) - Laboratoire Matière et Systèmes Complexes - Paris 7

More documents

Recommendations

Info

(1) holds everywhere except possibly in a boundary layer (cf. the discussion in the Introduction), region M 1 must be a spherical cap almost within the whole gap. Therefore, the radius of the spherical cap M1 is not r x a, but: r x a 1 G=s (14) This is our second central result. For G ¼ 0, we recover the standard approximation that M 1 is a half-sphere matching the tube radius. 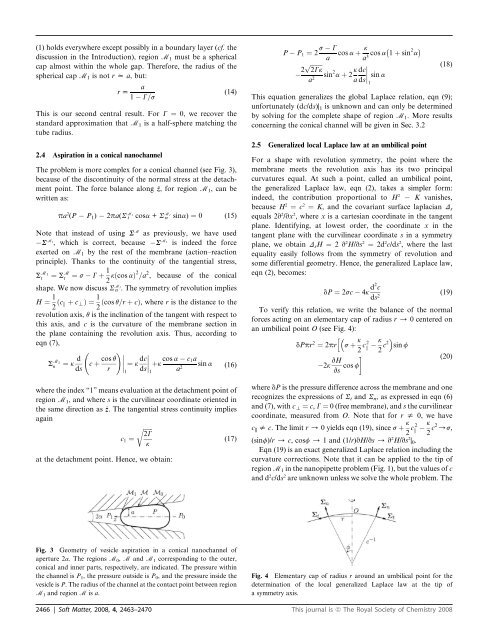2.4 Aspiration in a conical nanochannel The problem is more complex for a conical channel (see Fig. 3), because of the discontinuity of the normal stress at the detachment point. The force balance along ^z, for region M1, can be written as: pa 2 (P P1) 2pa(S M 1 t cosa + S M 1 n sina) ¼ 0 (15) Note that instead of using S M as previously, we have used S M 1 , which is correct, because S M 1 is indeed the force exerted on M1 by the rest of the membrane (action–reaction principle). Thanks to the continuity of the tangential stress, S M1 t ¼ SM t ¼ s G þ 1 2 kðcos aÞ2 =a 2 , because of the conical shape. We now discuss S M 1 n . The symmetry of revolution implies H ¼ 1 2 ðck þ ctÞ ¼ 1 ðcos q=r þ cÞ, where r is the distance to the 2 revolution axis, q is the inclination of the tangent with respect to this axis, and c is the curvature of the membrane section in the plane containing the revolution axis. Thus, according to eqn (7), S M ! d cos q 1 n ¼ k c þ ¼ k ds r 1 dc cos a c1a þk ds 1 a2 sin a (16) where the index ‘‘1’’ means evaluation at the detachment point of region M1, and where s is the curvilinear coordinate oriented in the same direction as ^z. The tangential stress continuity implies again rffiffiffiffiffiffi 2G c1 ¼ (17) k at the detachment point. Hence, we obtain: Fig. 3 Geometry of vesicle aspiration in a conical nanochannel of aperture 2a. The regions M 0, M and M 1 corresponding to the outer, conical and inner parts, respectively, are indicated. The pressure within the channel is P 1, the pressure outside is P 0, and the pressure inside the vesicle is P. The radius of the channel at the contact point between region M 1 and region M is a. s G P P1 ¼ 2 cos a þ a k a3 cos a 1 þ sin2a 2 ffiffiffiffiffiffiffiffi p 2Gk a2 sin 2 a þ 2 k dc sin a a ds 1 (18) This equation generalizes the global Laplace relation, eqn (9); unfortunately (dc/ds)|1 is unknown and can only be determined by solving for the complete shape of region M1. More results concerning the conical channel will be given in Sec. 3.2 2.5 Generalized local Laplace law at an umbilical point For a shape with revolution symmetry, the point where the membrane meets the revolution axis has its two principal curvatures equal. At such a point, called an umbilical point, the generalized Laplace law, eqn (2), takes a simpler form: indeed, the contribution proportional to H2 K vanishes, because H2 ¼ c2 ¼ K, and the covariant surface laplacian Ds equals 2v2 /vx2 , where x is a cartesian coordinate in the tangent plane. Identifying, at lowest order, the coordinate x in the tangent plane with the curvilinear coordinate s in a symmetry plane, we obtain DsH ¼ 2 v2H/vs2 ¼ 2d2c/ds2 , where the last equality easily follows from the symmetry of revolution and some differential geometry. Hence, the generalized Laplace law, eqn (2), becomes: dP ¼ 2sc 4k d2c ds2 (19) To verify this relation, we write the balance of the normal forces acting on an elementary cap of radius r / 0 centered on an umbilical point O (see Fig. 4): dPpr2 h ¼ 2pr s þ k 2 ck 2 2k vH cos f vs k 2 c2 sin f (20) where dP is the pressure difference across the membrane and one recognizes the expressions of St and Sn, as expressed in eqn (6) and (7), with ct ¼ c, G ¼ 0 (free membrane), and s the curvilinear coordinate, measured from O. Note that for r s 0, we have c|| s c. The limit r / 0 yields eqn (19), since s þ k 2 c 2 k k 2 c2 /s, (sinf)/r / c, cosf / 1 and (1/r)vH/vs / v 2 H/vs 2 |0. Eqn (19) is an exact generalized Laplace relation including the curvature corrections. Note that it can be applied to the tip of region M1 in the nanopipette problem (Fig. 1), but the values of c and d 2 c/ds 2 are unknown unless we solve the whole problem. The Fig. 4 Elementary cap of radius r around an umbilical point for the determination of the local generalized Laplace law at the tip of a symmetry axis. 2466 | Soft Matter, 2008, 4, 2463–2470 This journal is ª The Royal Society of Chemistry 2008
method we used above is therefore more efficient. Note, however, that this equation can be used at the tip of region M 0, implying that eqn (13) takes the exact form: DP ¼ 2s 1 a 1 R G sa þ k a 3 4k d2 c ds 2 where d 2 c/ds 2 is evaluated at the tip of region M0. 3 Energy minimization analysis (21) In this section we give the differential equations and the boundary conditions governing the shape of the free membrane inside the cylindrical or conical nanochannels of Fig. 1 and 3. As a first application, we discuss numerically the features of the solution that cannot be predicted analytically. We then redemonstrate analytically the detachment condition eqn (17) and the generalized Laplace eqn (18) and (19) that we have obtained from the stress tensor analysis. 3.1 Differential equations and boundary conditions We consider generically the region M1 in the conical nanochannel of Fig. 3. Indeed, the nanopipette geometry of Fig. 1 is a particular case where a ¼ 0, while the region M 0 of the conical nanochannel of Fig. 3 corresponds to the case a < 0. The minimization of the free energy shown ineqn (4) for axisymmetric shapes yields the set of differential equations for the profile of the free part M1: 27,28 j 00 ¼ g sin j kr þ ðP1 PÞr cos j 2k g 0 ¼ 1 2 k j 0 2 sin2 j r 2 þðP1 PÞr sin j j 0 cos j sin 2j þ r 2r2 þ s (22) (23) r 0 ¼ cosj (24) z 0 ¼ sinj (25) where j is the angle that the tangent to the profile forms with the radial direction r and a prime indicates derivation with respect to the arclength s. We set s ¼ 0 at the detachment point of M1 and s ¼ s1 at the revolution axis r ¼ 0; s1 is thus the length of the profile. The function g(s) is a Lagrange multiplier field that enforces the condition that s is everywhere the arclength of the profile. The boundary conditions are obtained by setting to zero the boundary terms of the free energy variation: 27,28 jð0Þ ¼ p þ a; rð0Þ ¼a; zð0Þ ¼0 (26) 2 j(s1) ¼ p, r(s1) ¼ 0 (27) P 2 gð0Þtan a P1 ¼ a2 2 s k cos a þ þ a cos a a3 k a cos a j 02 ð0Þ (28) P P1 ¼ 2 gð0Þtan a a2 2ðs GÞ k cos a þ þ a cos a a3 (29) Eqn (26) and (27) reflect the geometry of the problem, eqn (28) is obtained by varying the length of the axisymmetric contour (taking into account the existence of a conserved Hamiltonian29 ), and eqn (29) is obtained by displacing the detachment point of region M1, taking into account the free energy FM of the adhering part M: FM ¼ 2p ð z1 z0 k cos a 2rðzÞ ðs GÞrðzÞ þ þ cos a ðP1 PÞr2ðzÞ 2 dz (30) Here, r(z) ¼ a ztana is the equation of the cone, z0 is the z coordinate of the detachment point of M 0 and z 1 ¼ 0 is the z coordinate of the detachment point of M 1, at which r ¼ a. Eqn (22)–(25) with the seven boundary conditions (26)–(29) fully determine the problem. Indeed, we have five first-order partial differential equations, plus the two unknowns s1 and P P1. 3.2 Numerical analysis We solve numerically the shape eqn (22)–(25) with the boundary conditions shown in eqn (26)–(29) by using a standard finite difference scheme with deferred correction and Newtonian iteration 30 . We introduce the normalized quantities: z ¼ z r s ; r ¼ ; s ¼ a a a ; s1 ¼ s1 a s ¼ sa2 3 ðP P1Þa ; p ¼ k k (31) (32) G ¼ Ga2 ga ; g ¼ (33) k k To cope with the unknown parameters s1 and p, we write the differential equations in terms of the independent parameter t ¼ s/s1, with 0 # t # 1, and we treat s1 and p1 as two extra functions of the parameter t, obeying the differential equations ds1/dt ¼ dp/dt ¼ 0. Our problem is thus transformed into a standard set of seven first-order differential equations obeying seven boundary conditions: five at the left boundary t ¼ 0(s¼ 0) and two at the right boundary t ¼ 1, (s ¼ s1). Because of the singularity of the differential equations at r ¼ 0, we replace the two right boundary conditions, eqn (27), with the following conditions at s ¼ s1 3 (with 0 < 3 s1): r (s1 3) ¼ 3 (34) jðs1 3Þ ¼p 3 1 þ 3 3 gðs1 3Þ dj ds s1 3 (35) that are obtained by solving the shape equations up to order 3 2 around s ¼ s1. Shapes in the cylindrical nanochannel In the absence of adhesion (G ¼ 0), we find that the vesicle has a prolate shape at small tensions (see top curves of Fig. 5). As the This journal is ª The Royal Society of Chemistry 2008 Soft Matter, 2008, 4, 2463–2470 | 2467
Page 1 and 2: PAPER www.rsc.org/softmatter | Soft
Page 3: Note that eqn (5)-(7) satisfy the a
Page 7 and 8: Fig. 8 The deviation p 2scosa of th

PDF (361 K) - Laboratoire Matière et Systèmes Complexes - Paris 7

You also want an ePaper? Increase the reach of your titles

Delete template?

Save as template?