repaso de fundamentos matemáticos - QueGrande

PÁG. 18 DE 24 TEMA 0: REPASO FUNDAMENTOS MATEMÁTICOS
P) La diferencial total permite hallar el cambio completo de la función cuando se modifican
ligeramente los valores de todas las variables de las que depende.
EJEMPLO 0. 28: Contestando a la pregunta final planteada en el Ejemplo 0. 27, obtenemos
2
EK
EK
v
dEK
= dm+ dv = dm+
mvdv
m v 2
(T0. 38)
Este cambio específico se expresa en Julios.
La definición puede extenderse a funciones de muchas variables. Por ejemplo, si y depende de N
variables x 1 , x 2 , …., x N , la diferencial total de w es:
N
y y y y
y = yx ( , x ,..., x ) Þ dy = dx + dx + ... + dx = dx
1 2 N 1 2
N n
x1 x2
xN
n=
1
xn
å
(T0. 39)
PREGUNTA: ¿Cómo se lee esta fórmula
En esta asignatura sólo trabajaremos con funciones de hasta 3 variables independientes.
0.2.9. INTEGRAL INDEFINIDA (FUNCIÓN UNIVARIABLE)
Q) ¿Qué es La integral indefinida es la operación inversa de la derivada. Por eso también se la
denomina antiderivada.
EJEMPLO 0. 29: Si tienes una función g(x)=cos(x), piensa que g es una función que ya está derivada;
entonces, pregúntate: ¿Cuál es la función f(x) que tengo que derivar para obtener g(x) En este caso ya
conoces la respuesta: f(x)=sen(x), porque df(x)/dx=cos(x)=g(x). En realidad, es cualquier función
sen(x)+constante, puesto que al derivar una constante obtienes cero, es decir:
f(x)=sen(x)+CÞdf/dx=d[sen(x)]/dx+dC/dx=cos(x)+0=cos(x). (T0. 40)
C) ¿Cómo se representa y cómo se calcula
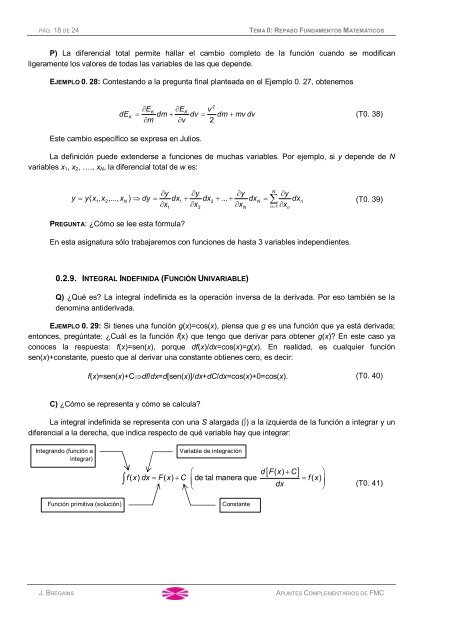
La integral indefinida se representa con una S alargada (∫) a la izquierda de la función a integrar y un
diferencial a la derecha, que indica respecto de qué variable hay que integrar:
Integrando (función a
integrar)
Función primitiva (solución)
ò
Variable de integración
[ ( ) + C]
æ
dFx
ö
f( x) dx = Fx ( ) + C de tal manera que = f( x)
ç ÷
è
dx ø
(T0. 41)
Constante
J. BRÉGAINS APUNTES COMPLEMENTARIOS DE FMC