Trigonometrische Substitutionen - imng
Trigonometrische Substitutionen - imng
Trigonometrische Substitutionen - imng
Sie wollen auch ein ePaper? Erhöhen Sie die Reichweite Ihrer Titel.
YUMPU macht aus Druck-PDFs automatisch weboptimierte ePaper, die Google liebt.
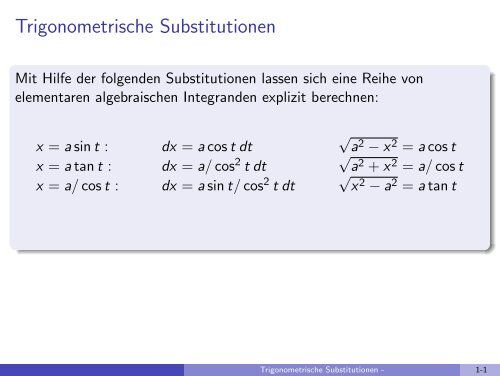
<strong>Trigonometrische</strong> <strong>Substitutionen</strong><br />
Mit Hilfe der folgenden <strong>Substitutionen</strong> lassen sich eine Reihe von<br />
elementaren algebraischen Integranden explizit berechnen:<br />
x = a sin t :<br />
x = a tan t :<br />
x = a/ cos t :<br />
dx = a cos t dt<br />
dx = a/ cos 2 t dt<br />
dx = a sin t/ cos 2 t dt<br />
√<br />
√ a 2 − x 2 = a cos t<br />
√ a 2 + x 2 = a/ cos t<br />
x 2 − a 2 = a tan t<br />
<strong>Trigonometrische</strong> <strong>Substitutionen</strong> - 1-1
<strong>Trigonometrische</strong> <strong>Substitutionen</strong><br />
Mit Hilfe der folgenden <strong>Substitutionen</strong> lassen sich eine Reihe von<br />
elementaren algebraischen Integranden explizit berechnen:<br />
x = a sin t :<br />
x = a tan t :<br />
x = a/ cos t :<br />
dx = a cos t dt<br />
dx = a/ cos 2 t dt<br />
dx = a sin t/ cos 2 t dt<br />
√<br />
√ a 2 − x 2 = a cos t<br />
√ a 2 + x 2 = a/ cos t<br />
x 2 − a 2 = a tan t<br />
Gegebenenfalls müssen die Argumente der Wurzel zunächst durch<br />
quadratische Ergänzung auf Standardform gebracht werden.<br />
<strong>Trigonometrische</strong> <strong>Substitutionen</strong> - 1-2
Beispiel:<br />
∫ 1/2<br />
0<br />
√<br />
1 − x 2 dx<br />
<strong>Trigonometrische</strong> <strong>Substitutionen</strong> - 2-1
Beispiel:<br />
∫ 1/2<br />
0<br />
√<br />
1 − x 2 dx<br />
trigonometrische Substitution:<br />
x = sin t, dx = cos t dt, x = 0 → t = 0, x = 1/2 → t = π/6<br />
<br />
∫ π/6<br />
0<br />
[ ] 1 π/6<br />
cos 2 t dt =<br />
2 (sin t cos t + t) =<br />
0<br />
√<br />
3<br />
8 + π 12<br />
<strong>Trigonometrische</strong> <strong>Substitutionen</strong> - 2-2
Beispiel:<br />
trigonometrische Substitution:<br />
<br />
∫ 1/2<br />
0<br />
√<br />
1 − x 2 dx<br />
x = sin t, dx = cos t dt, x = 0 → t = 0, x = 1/2 → t = π/6<br />
∫ π/6<br />
0<br />
[ ] 1 π/6<br />
cos 2 t dt =<br />
2 (sin t cos t + t) =<br />
0<br />
√<br />
3<br />
8 + π 12<br />
Rücktransformation von [. . .] Stammfunktion<br />
∫ √1<br />
− x 2 dx = x √<br />
1 − x<br />
2<br />
2 + 1 2 arcsin x + c<br />
(cos t = √ 1 − sin 2 t = √ 1 − x 2 )<br />
<strong>Trigonometrische</strong> <strong>Substitutionen</strong> - 2-3
(ii) geometrisches Argument:<br />
<strong>Trigonometrische</strong> <strong>Substitutionen</strong> - 2-4
(ii) geometrisches Argument:<br />
1<br />
B<br />
A<br />
0 x 1<br />
<strong>Trigonometrische</strong> <strong>Substitutionen</strong> - 2-5
(ii) geometrisches Argument:<br />
1<br />
B<br />
A<br />
0 x 1<br />
Fläche unter dem Graph von √ 1 − x 2 : Summe von zwei Teilflächen<br />
<strong>Trigonometrische</strong> <strong>Substitutionen</strong> - 2-6
(ii) geometrisches Argument:<br />
1<br />
B<br />
A<br />
0 x 1<br />
Fläche unter dem Graph von √ 1 − x 2 : Summe von zwei Teilflächen<br />
Dreieck: |A| = x √ 1 − x 2 /2<br />
<strong>Trigonometrische</strong> <strong>Substitutionen</strong> - 2-7
(ii) geometrisches Argument:<br />
1<br />
B<br />
A<br />
0 x 1<br />
Fläche unter dem Graph von √ 1 − x 2 : Summe von zwei Teilflächen<br />
Dreieck: |A| = x √ 1 − x 2 /2<br />
Kreissektor mit Öffnungswinkel t: |B| = t/2 = (1/2) arcsin x<br />
<strong>Trigonometrische</strong> <strong>Substitutionen</strong> - 2-8
(ii) geometrisches Argument:<br />
1<br />
B<br />
A<br />
0 x 1<br />
Fläche unter dem Graph von √ 1 − x 2 : Summe von zwei Teilflächen<br />
Dreieck: |A| = x √ 1 − x 2 /2<br />
Kreissektor mit Öffnungswinkel t: |B| = t/2 = (1/2) arcsin x<br />
gleiche Stammfunktion |A| + |B|<br />
<strong>Trigonometrische</strong> <strong>Substitutionen</strong> - 2-9
Beispiel:<br />
∫<br />
dx<br />
x √ 1 + x 2<br />
<strong>Trigonometrische</strong> <strong>Substitutionen</strong> - 3-1
Beispiel:<br />
∫<br />
dx<br />
x √ 1 + x 2<br />
trigonometrische Substitution x = tan t,<br />
∫<br />
dt/ cos 2 ∫<br />
t<br />
tan t/ cos t =<br />
dx = 1/ cos 2 t dt <br />
dt<br />
sin t = ln | tan t 2 | + c<br />
( √ 1 + x 2 = 1/ cos t, Formel für Stammfunktion von 1/ sin)<br />
<strong>Trigonometrische</strong> <strong>Substitutionen</strong> - 3-2
Beispiel:<br />
∫<br />
dx<br />
x √ 1 + x 2<br />
trigonometrische Substitution x = tan t,<br />
∫<br />
dt/ cos 2 ∫<br />
t<br />
tan t/ cos t =<br />
dx = 1/ cos 2 t dt <br />
dt<br />
sin t = ln | tan t 2 | + c<br />
( √ 1 + x 2 = 1/ cos t, Formel für Stammfunktion von 1/ sin)<br />
Rücksubstitution =⇒<br />
∫<br />
dx<br />
x √ 1 + x = ln | tan(1 arctan x)| + c<br />
2 2<br />
<strong>Trigonometrische</strong> <strong>Substitutionen</strong> - 3-3
estimmtes Integral<br />
∫ ∞<br />
1<br />
dx<br />
x √ 1 + x 2<br />
<strong>Trigonometrische</strong> <strong>Substitutionen</strong> - 3-4
estimmtes Integral<br />
∫ ∞<br />
1<br />
dx<br />
x √ 1 + x 2<br />
Verwendung der berechneten Stammfunktion <br />
ln | tan π 4 | − ln | tan π 8 | = ln 1 − ln(√ 2 − 1) = ln(1 + √ 2)<br />
Berechnung von tan(π/8) mit Hilfe der Diagonale einer Raute mit spitzem<br />
Winkel π/4<br />
(1/ √ 2, 1/ √ 2) (1 + 1/ √ 2, 1/ √ 2)<br />
(0, 0) (1, 0)<br />
=⇒ tan(π/8) = (1/ √ 2)/(1/ √ 2 + 1) = 1 √<br />
2+1<br />
= √ 2 − 1<br />
<strong>Trigonometrische</strong> <strong>Substitutionen</strong> - 3-5
Beispiel:<br />
∫<br />
dx<br />
√<br />
x 2 − 6x + 5<br />
<strong>Trigonometrische</strong> <strong>Substitutionen</strong> - 4-1
Beispiel:<br />
∫<br />
dx<br />
√<br />
x 2 − 6x + 5<br />
quadratische Ergänzung Standardform der Wurzel<br />
∫<br />
dx<br />
√<br />
(x − 3) 2 − 4 = 1 ∫<br />
dx<br />
√<br />
2 ((x − 3)/2) 2 − 1<br />
<strong>Trigonometrische</strong> <strong>Substitutionen</strong> - 4-2
Beispiel:<br />
∫<br />
dx<br />
√<br />
x 2 − 6x + 5<br />
quadratische Ergänzung Standardform der Wurzel<br />
∫<br />
dx<br />
√<br />
(x − 3) 2 − 4 = 1 ∫<br />
dx<br />
√<br />
2 ((x − 3)/2) 2 − 1<br />
vorbereitende Substitution y = (x − 3)/2, dx = 2dy Vereinfachung:<br />
∫<br />
∫<br />
dx<br />
√<br />
x 2 − 6x + 5 = dy<br />
√<br />
y 2 − 1<br />
<strong>Trigonometrische</strong> <strong>Substitutionen</strong> - 4-3
trigonometrische Substitution y = 1/ cos t, dy = sin t/ cos 2 t dt,<br />
√<br />
y 2 − 1 = tan t <br />
∫<br />
∫<br />
dy<br />
√<br />
y 2 − 1 =<br />
∣ ∣<br />
dt ∣∣∣<br />
cos t = ln 1 ∣∣∣<br />
cos t + tan t + c<br />
(Formel für ∫ cos −1 , Überprüfung durch Differenzieren)<br />
<strong>Trigonometrische</strong> <strong>Substitutionen</strong> - 4-4
trigonometrische Substitution y = 1/ cos t, dy = sin t/ cos 2 t dt,<br />
√<br />
y 2 − 1 = tan t <br />
∫<br />
∫<br />
dy<br />
√<br />
y 2 − 1 =<br />
∣ ∣<br />
dt ∣∣∣<br />
cos t = ln 1 ∣∣∣<br />
cos t + tan t + c<br />
(Formel für ∫ cos −1 , Überprüfung durch Differenzieren)<br />
Rücksubstitution <br />
∣<br />
ln ∣y + y √ √ (x )<br />
y 2 − 1∣ + c = ln<br />
x − 3 − 3 2 +<br />
− 1<br />
∣ 2 2<br />
∣ + c<br />
<strong>Trigonometrische</strong> <strong>Substitutionen</strong> - 4-5