Verfahren von Gram-Schmidt - imng
Verfahren von Gram-Schmidt - imng
Verfahren von Gram-Schmidt - imng
Sie wollen auch ein ePaper? Erhöhen Sie die Reichweite Ihrer Titel.
YUMPU macht aus Druck-PDFs automatisch weboptimierte ePaper, die Google liebt.
<strong>Verfahren</strong> <strong>von</strong> <strong>Gram</strong>-<strong>Schmidt</strong><br />
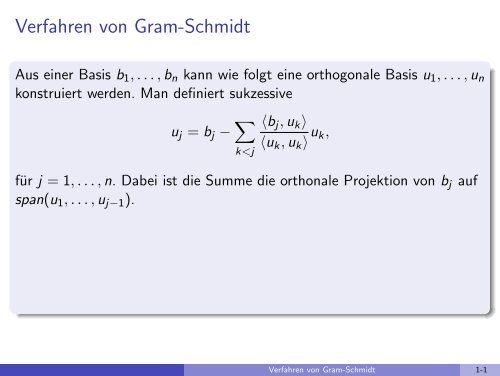
Aus einer Basis b 1 , . . . , b n kann wie folgt eine orthogonale Basis u 1 , . . . , u n<br />
konstruiert werden. Man definiert sukzessive<br />
u j = b j − ∑ k
<strong>Verfahren</strong> <strong>von</strong> <strong>Gram</strong>-<strong>Schmidt</strong><br />
Aus einer Basis b 1 , . . . , b n kann wie folgt eine orthogonale Basis u 1 , . . . , u n<br />
konstruiert werden. Man definiert sukzessive<br />
u j = b j − ∑ k
Beweis:<br />
Zeige induktiv: u 1 , . . . , u l bilden orthogonale Basis für span (b 1 , . . . , b l ).<br />
<strong>Verfahren</strong> <strong>von</strong> <strong>Gram</strong>-<strong>Schmidt</strong> 2-1
Beweis:<br />
Zeige induktiv: u 1 , . . . , u l bilden orthogonale Basis für span (b 1 , . . . , b l ).<br />
l = 1 : u 1 = b 1 ̌<br />
<strong>Verfahren</strong> <strong>von</strong> <strong>Gram</strong>-<strong>Schmidt</strong> 2-2
Beweis:<br />
Zeige induktiv: u 1 , . . . , u l bilden orthogonale Basis für span (b 1 , . . . , b l ).<br />
l = 1 : u 1 = b 1 ̌<br />
Induktionsschritt (l → l + 1):<br />
<strong>Verfahren</strong> <strong>von</strong> <strong>Gram</strong>-<strong>Schmidt</strong> 2-3
Beweis:<br />
Zeige induktiv: u 1 , . . . , u l bilden orthogonale Basis für span (b 1 , . . . , b l ).<br />
l = 1 : u 1 = b 1 ̌<br />
Induktionsschritt (l → l + 1):<br />
für j ≤ l<br />
〈u l+1 , u j 〉 = 〈b l+1 , u j 〉 − ∑ 〈b l+1 , u k 〉<br />
〈u k , u k 〉 〈u k, u j 〉 = 0<br />
} {{ }<br />
k≤l<br />
δ k,j |u k | 2<br />
=⇒ u 1 , . . . , u l+1 orthogonal<br />
<strong>Verfahren</strong> <strong>von</strong> <strong>Gram</strong>-<strong>Schmidt</strong> 2-4
Beweis:<br />
Zeige induktiv: u 1 , . . . , u l bilden orthogonale Basis für span (b 1 , . . . , b l ).<br />
l = 1 : u 1 = b 1 ̌<br />
Induktionsschritt (l → l + 1):<br />
für j ≤ l<br />
〈u l+1 , u j 〉 = 〈b l+1 , u j 〉 − ∑ 〈b l+1 , u k 〉<br />
〈u k , u k 〉 〈u k, u j 〉 = 0<br />
} {{ }<br />
k≤l<br />
δ k,j |u k | 2<br />
=⇒ u 1 , . . . , u l+1 orthogonal<br />
b l+1 − u l+1 ∈ span(u 1 , . . . , u l ) = span(b 1 , . . . , b l )<br />
<strong>Verfahren</strong> <strong>von</strong> <strong>Gram</strong>-<strong>Schmidt</strong> 2-5
Beweis:<br />
Zeige induktiv: u 1 , . . . , u l bilden orthogonale Basis für span (b 1 , . . . , b l ).<br />
l = 1 : u 1 = b 1 ̌<br />
Induktionsschritt (l → l + 1):<br />
für j ≤ l<br />
〈u l+1 , u j 〉 = 〈b l+1 , u j 〉 − ∑ 〈b l+1 , u k 〉<br />
〈u k , u k 〉 〈u k, u j 〉 = 0<br />
} {{ }<br />
k≤l<br />
δ k,j |u k | 2<br />
=⇒ u 1 , . . . , u l+1 orthogonal<br />
b l+1 − u l+1 ∈ span(u 1 , . . . , u l ) = span(b 1 , . . . , b l )<br />
⇒<br />
Beide Basen spannen denselben Unterraum auf.<br />
<strong>Verfahren</strong> <strong>von</strong> <strong>Gram</strong>-<strong>Schmidt</strong> 2-6
Beispiel:<br />
Konstruktion einer bzgl. des Skalarproduktes<br />
〈f , g〉 =<br />
∫ 1<br />
−1<br />
f (x)g(x) dx<br />
orthogonalen Folge <strong>von</strong> Polynomen p 0 , p 1 , . . . aus den Monomen<br />
q j (x) = x j .<br />
<strong>Verfahren</strong> <strong>von</strong> <strong>Gram</strong>-<strong>Schmidt</strong> 3-1
Beispiel:<br />
Konstruktion einer bzgl. des Skalarproduktes<br />
〈f , g〉 =<br />
∫ 1<br />
−1<br />
f (x)g(x) dx<br />
orthogonalen Folge <strong>von</strong> Polynomen p 0 , p 1 , . . . aus den Monomen<br />
q j (x) = x j .<br />
<strong>Verfahren</strong> <strong>von</strong> <strong>Gram</strong>-<strong>Schmidt</strong>:<br />
p 0 = q 0 = 1<br />
<strong>Verfahren</strong> <strong>von</strong> <strong>Gram</strong>-<strong>Schmidt</strong> 3-2
Beispiel:<br />
Konstruktion einer bzgl. des Skalarproduktes<br />
〈f , g〉 =<br />
∫ 1<br />
−1<br />
f (x)g(x) dx<br />
orthogonalen Folge <strong>von</strong> Polynomen p 0 , p 1 , . . . aus den Monomen<br />
q j (x) = x j .<br />
<strong>Verfahren</strong> <strong>von</strong> <strong>Gram</strong>-<strong>Schmidt</strong>:<br />
p 0 = q 0 = 1<br />
∫ 1<br />
−1<br />
p 1 = q 1 −<br />
x · 1 dx<br />
∫ 1<br />
−1 1 · 1 dx · 1 = x − 0 <strong>Verfahren</strong> <strong>von</strong> <strong>Gram</strong>-<strong>Schmidt</strong> 3-3
Beispiel:<br />
Konstruktion einer bzgl. des Skalarproduktes<br />
〈f , g〉 =<br />
∫ 1<br />
−1<br />
f (x)g(x) dx<br />
orthogonalen Folge <strong>von</strong> Polynomen p 0 , p 1 , . . . aus den Monomen<br />
q j (x) = x j .<br />
<strong>Verfahren</strong> <strong>von</strong> <strong>Gram</strong>-<strong>Schmidt</strong>:<br />
p 0 = q 0 = 1<br />
∫ 1<br />
−1<br />
p 1 = q 1 − ∫ x · 1 dx<br />
1<br />
−1 1 · 1 dx · 1 = x − 0<br />
p 2 = q 2 −<br />
∫ 1<br />
−1 x 2 · x dx<br />
∫ 1<br />
−1 x · x dx · x − ∫ 1<br />
−1 x 2 · 1 dx<br />
∫ 1<br />
−1 1 · 1 dx · 1 = x 2 − 0 · x − 1 3<br />
<strong>Verfahren</strong> <strong>von</strong> <strong>Gram</strong>-<strong>Schmidt</strong> 3-4
Beispiel:<br />
Konstruktion einer bzgl. des Skalarproduktes<br />
〈f , g〉 =<br />
∫ 1<br />
−1<br />
f (x)g(x) dx<br />
orthogonalen Folge <strong>von</strong> Polynomen p 0 , p 1 , . . . aus den Monomen<br />
q j (x) = x j .<br />
<strong>Verfahren</strong> <strong>von</strong> <strong>Gram</strong>-<strong>Schmidt</strong>:<br />
p 0 = q 0 = 1<br />
∫ 1<br />
−1<br />
p 1 = q 1 − ∫ x · 1 dx<br />
1<br />
−1 1 · 1 dx · 1 = x − 0<br />
p 2 = q 2 −<br />
∫ 1<br />
−1 x 2 · x dx<br />
∫ 1<br />
−1 x · x dx · x − ∫ 1<br />
−1 x 2 · 1 dx<br />
∫ 1<br />
−1 1 · 1 dx · 1 = x 2 − 0 · x − 1 3<br />
allgemeine Formel: p n+1 = q n+1 − ∑ n 〈q n+1 ,p j 〉<br />
j=0 〈p j ,p j 〉<br />
p j<br />
<strong>Verfahren</strong> <strong>von</strong> <strong>Gram</strong>-<strong>Schmidt</strong> 3-5
Vereinfachung durch Verwendung <strong>von</strong> ˜q n+1 (x) = xp n (x) anstelle <strong>von</strong><br />
q n+1 (x) = x n+1 .<br />
<strong>Verfahren</strong> <strong>von</strong> <strong>Gram</strong>-<strong>Schmidt</strong> 3-6
Vereinfachung durch Verwendung <strong>von</strong> ˜q n+1 (x) = xp n (x) anstelle <strong>von</strong><br />
q n+1 (x) = x n+1 .<br />
Legitim, da<br />
span{p 1 , . . . , p n , q n+1 } = span{p 1 , . . . , p n , ˜q n+1 }<br />
<strong>Verfahren</strong> <strong>von</strong> <strong>Gram</strong>-<strong>Schmidt</strong> 3-7
Vereinfachung durch Verwendung <strong>von</strong> ˜q n+1 (x) = xp n (x) anstelle <strong>von</strong><br />
q n+1 (x) = x n+1 .<br />
Legitim, da<br />
span{p 1 , . . . , p n , q n+1 } = span{p 1 , . . . , p n , ˜q n+1 }<br />
alle Summanden bis auf j = n − 1 Null<br />
<strong>Verfahren</strong> <strong>von</strong> <strong>Gram</strong>-<strong>Schmidt</strong> 3-8
Vereinfachung durch Verwendung <strong>von</strong> ˜q n+1 (x) = xp n (x) anstelle <strong>von</strong><br />
q n+1 (x) = x n+1 .<br />
Legitim, da<br />
span{p 1 , . . . , p n , q n+1 } = span{p 1 , . . . , p n , ˜q n+1 }<br />
alle Summanden bis auf j = n − 1 Null<br />
j = n : auf Grund der Antisymmetrie des Integranden<br />
〈˜q n+1 , q n 〉 =<br />
∫ 1<br />
−1<br />
x q n (x) q n (x)dx = 0<br />
<strong>Verfahren</strong> <strong>von</strong> <strong>Gram</strong>-<strong>Schmidt</strong> 3-9
Vereinfachung durch Verwendung <strong>von</strong> ˜q n+1 (x) = xp n (x) anstelle <strong>von</strong><br />
q n+1 (x) = x n+1 .<br />
Legitim, da<br />
span{p 1 , . . . , p n , q n+1 } = span{p 1 , . . . , p n , ˜q n+1 }<br />
alle Summanden bis auf j = n − 1 Null<br />
j = n : auf Grund der Antisymmetrie des Integranden<br />
〈˜q n+1 , q n 〉 =<br />
∫ 1<br />
−1<br />
x q n (x) q n (x)dx = 0<br />
j < n − 1 : [x p j (x)] Linearkombination <strong>von</strong> p k (x), k < n =⇒<br />
〈˜q n+1 , p j 〉 =<br />
∫ 1<br />
−1<br />
p n (x) [x p j (x)] dx = 0 ,<br />
da p n ⊥ p k<br />
<strong>Verfahren</strong> <strong>von</strong> <strong>Gram</strong>-<strong>Schmidt</strong> 3-10
andere Normierung Legendre-Polynome<br />
p n (x) = α n x n + . . . , α n = (2n)!<br />
2 n (n!) 2<br />
<strong>Verfahren</strong> <strong>von</strong> <strong>Gram</strong>-<strong>Schmidt</strong> 3-11
andere Normierung Legendre-Polynome<br />
p n (x) = α n x n + . . . , α n = (2n)!<br />
2 n (n!) 2<br />
<strong>Gram</strong>-<strong>Schmidt</strong>-Prozess 3-Term-Rekursion<br />
mit p 0 (x) = 1 und p 1 (x) = x<br />
(n + 1)p n+1 (x) = (2n + 1)xp n (x) − np n−1 (x)<br />
<strong>Verfahren</strong> <strong>von</strong> <strong>Gram</strong>-<strong>Schmidt</strong> 3-12