On the Stochastic Cahn-Hilliard/Allen-Cahn equation - Isaac Newton ...

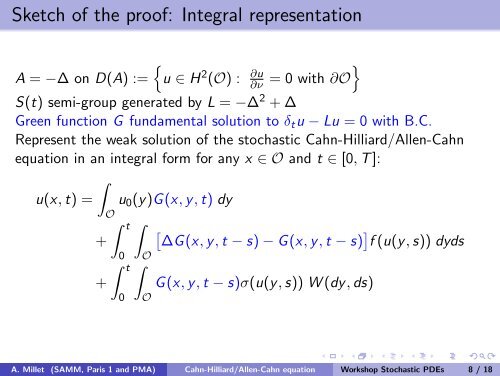
Sketch of the proof: Integral representation
A = −∆ on D(A) :=
�
u ∈ H 2 (O) : ∂u
∂ν
�
= 0 with ∂O
S(t) semi-group generated by L = −∆2 + ∆
Green function G fundamental solution to δtu − Lu = 0 with B.C.
Represent the weak solution of the stochastic Cahn-Hilliard/Allen-Cahn
equation in an integral form for any x ∈ O and t ∈ [0, T ]:
�
u(x, t) = u0(y)G(x, y, t) dy
O
� t �
� �
+ ∆G(x, y, t − s) − G(x, y, t − s) f (u(y, s)) dyds
0 O
� t �
+ G(x, y, t − s)σ(u(y, s)) W (dy, ds)
0
O
A. Millet (SAMM, Paris 1 and PMA) Cahn-Hilliard/Allen-Cahn equation Workshop Stochastic PDEs 8 / 18